filmov
tv
Calculus 3 Lecture 12.4: Velocity and Acceleration of Vector Functions

Показать описание
Calculus 3 Lecture 12.4: Velocity and Acceleration of Vector Functions: How to find the Velocity and Acceleration of a particle when the particular motion is described by a vector function (space curve). How to integrate to find a position function from acceleration or velocity. How to define acceleration as "T" and "N". A look at Projectile Motion.
Calculus 3 Lecture 12.4: Velocity and Acceleration of Vector Functions
Calculus 3: Vector Calculus: Motion in Plane (3 of 15) Motion Along a Circle
Calculus 3: Lecture 12.4 Tangent Vectors and Normal Vectors
Calculus 3: Lecture 12.3 Velocity and Acceleration
Calculus 3: Vector Calculus in 2D (29 of 39) Motion Along a Circle
Calculus 3: Vector Calculus: Motion in Plane (5 of 15) Find Velocity and Position
Calculus 3: Integration - Equations of Lines & Planes (13 of 27) Plane - Find Scalar & Norma...
Calculus 3: Vector Calculus: Motion in Plane (4 of 15) Acceleration in a Plane
OSCILLATION || 5.9. Graphical Representation of S.H.M. || LECT NO 05 || AARAMBH 4.0 BATCH #hsc
Calculus 3: Partial Derivative (4 of 30) Understanding What is a Partial Derivative: Example 2
Calculus 3: Vector Calculus: Motion in Plane (9 of 15) Position, Velocity, Acceleration (Part 2)
Calculus 3: Ch 2.1 Lines and Vectors in 3-D (4 of 20) Find Velocity and Acceleration Vectors
Multivariate Calculus: Lecture 12 part 1: kinematics in 3D
Calculus 3 Lecture 12.3: Arc Length/Parameterization, TNB (Frenet-Serret) Intro
Calculus 3 Ch 12.4 Unit Tangent and Normal vectors
Calculus 3 - Intro To Vectors
Week 5 Calculus 3 Position, Velocity, Acceleration
Speed (magnitude of velocity) Calculus 3
What are the big ideas of Multivariable Calculus?? Full Course Intro
Calculus 3 Ch 12.1-12.3 Vector-valued functions part 1
Calculus 3: Vector Calculus: Motion in Plane (12 of 15) Finding the Acceleration Ex. 1 (Part 1)
(Calculus 3) calculus on vector valued curves motion in space and initial value problems
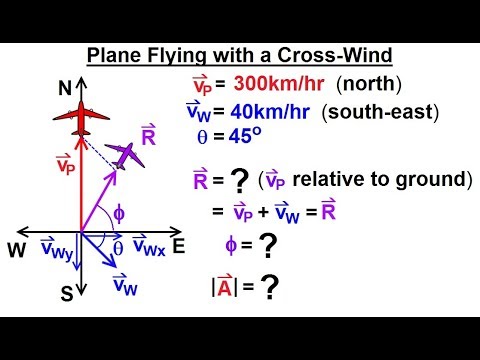
Calculus 3: Vector Calculus in 2D (11 of 39) Plane Flying with a Cross-Wind
Calculus 3 Ch 12.1-12.3 Vector-valued functions part 2
Комментарии
 1:02:04
1:02:04
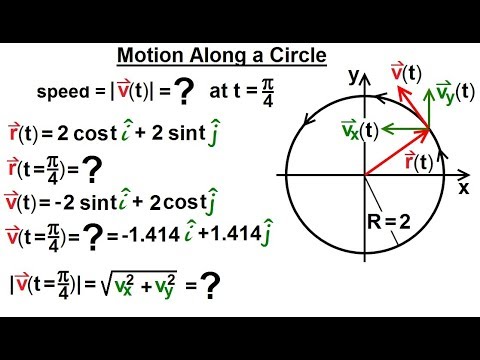 0:03:09
0:03:09
 1:13:21
1:13:21
 0:22:42
0:22:42
 0:04:08
0:04:08
 0:04:33
0:04:33
 0:06:31
0:06:31
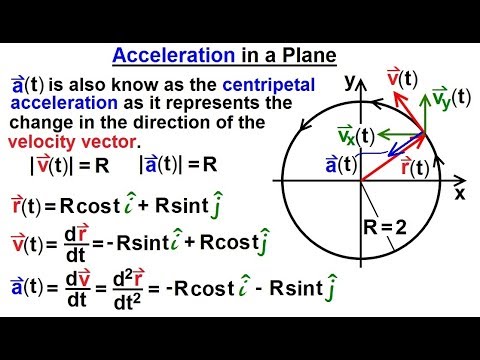 0:03:46
0:03:46
 0:35:16
0:35:16
 0:05:31
0:05:31
 0:03:57
0:03:57
 0:02:25
0:02:25
 0:59:51
0:59:51
 2:12:45
2:12:45
 0:56:49
0:56:49
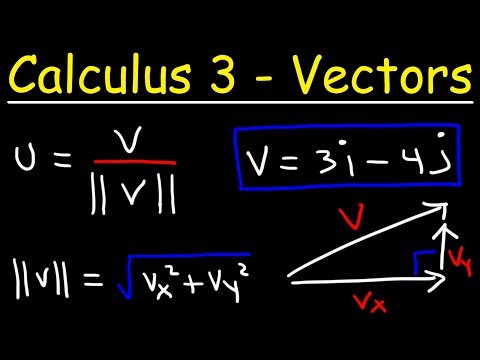 0:57:54
0:57:54
 0:04:12
0:04:12
 0:02:58
0:02:58
 0:16:50
0:16:50
 0:22:35
0:22:35
 0:05:25
0:05:25
 0:11:56
0:11:56
 0:08:04
0:08:04
 0:35:47
0:35:47