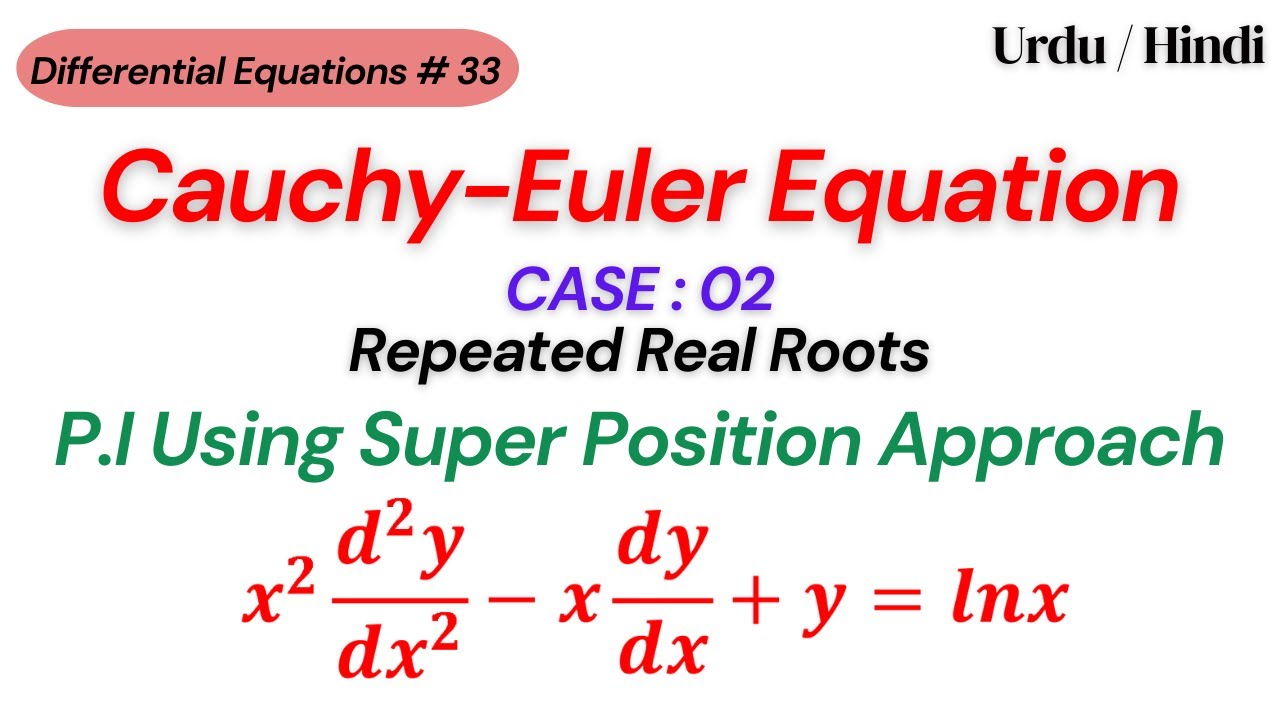
Cauchy Euler Equations and Super Position Approach || Differential Equations

x^2y''-xy'+y=ln(x)

This is a good problem involving a Cauchy - Euler equation where we'll use the method of super position approach to find a particular solution. Be careful: there's a common error that many students make! We take our time and point out the error. You might want to review the method super position approach before trying this problem; link below. More problems are linked below.

Reference Videos:
▶️Cauchy Euler Equations and Variation of Parameters || Differential Equations

▶️Use Variation of Parameters to Solve y'''+ y'= tanx

▶️Variation of Parameters || Nonhomogeneous Second Order Differential Equations

▶️Integrals of powers of tan using Integral Reduction

▶️Annihilator Operators || Differential Operator || Differential Equation

▶️Method of Undetermined Coefficients | Superposition Approach | 20 Examples

▶️Method of Undetermined Coefficients Superposition Approach || Differential Equations

▶️Linear Higher Order Differential Equation | Distinct Roots | Repeated Roots | Complex Roots

▶️Homogeneous Differential Equation With Constant Coefficients | Higher Order | Concept | Examples

▶️Homogeneous Differential Equation With Constant Coefficients | Conjugate Complex Roots

⏩Comment Below If This Video Helped You 💯
Like 👍 & Share With Your buddies

📚 Important Course Playlists
▶️ Partial Differential Equations (Full Course):

▶️ Definite Integrals:

Example will help Engineering and Basic Science students to understand the following topic of Mathematics:
1. How to use the super position approach Method to Solve a Cauchy Euler Equation
2. Example Of super position approach Method to Solve a Cauchy Euler Equation
3. how to solve cauchy euler differential equation using x=e^t
4.Concept Of particular solution using method of undetermined coefficients using super position approach
5. This is helpful For CSIR NET, IIT-JAM, GATE Exams.
6. This is Part Of the Differential Equation.

cauchy euler equation
cauchy euler differential equation
cauchy euler
differential equations
euler cauchy equations
cauchy euler equation example
cauchy linear differential equation
euler differential equation
euler cauchy differential equations
cauchy euler differential equation nonhomogeneous
cauchy homogeneous linear differential equation
cauchy,cauchy linear equation
euler
cauchy-euler equation
cauchy euler equation problem
using substitution to solve a cauchy euler equation
solve cauchy euler
nonhomogeneous cauchy euler equation
cauchy euler non homogeneous equation
cauchy euler non homogeneous differential equation
non homogeneous cauchy euler differential equations
euler's equation
superposition approach
method of undetermined coefficients
undetermined coefficients superposition approach
method of undetermined coefficients differential equations
superposition principle approach
method of undetermined coefficient
examples of superposition principle
method to find particular integral
undetermined coefficients method - superposition principle approach
particular integral method
all possible cases of undetermined coefficients method
#OrdinaryDifferentialEquations #ODEs#ODEExamples #ODEIdentification #ODEImportance #ODEHistory #ODEInventor #ODEExplanation #ODEApplications #ODESolvingMethods #ODEInnovators #ODELimitations #ODETips #ODEResources #higherorderdifferentialequations #modellingwithdifferentialequations #differentialequations #cauchyeulerequation #lineardifferentialequation #exercise4.6 #undeterminedcoefficientsmethod #undeterminedcoefficients
#particularintegral #annihilator #annihilatoroperator #annihilatormethod

My Social Media Ends:
Instagram:
Facebook:
📧 Contact:

Thank you for embarking on this mathematical exploration with us! Don't forget to [subscribe/like/share] if you find value in our content.