Perpendicular Bisector of a Line Segment and Triangle

Показать описание

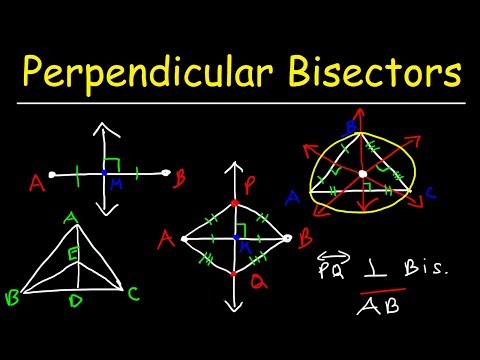
This geometry video tutorial provides a basic introduction into the perpendicular bisector of a line segment and a triangle. it discusses the perpendicular bisector theorem and the definition of perpendicular bisectors in addition to how to use them in a geometry two column proof problem with statements and reasons. This tutorial also describes how to identify the location of the circumcenter of a acute, right, and obtuse triangle after drawing all 3 altitudes from the vertex of the triangle to the midpoint of the opposite. This video contains plenty of examples and geometry practice problems.

Triangle Congruence - SSS, SAS, ASA:

CPCTC - 2 Column Proofs With Triangles:

Hypotenuse Leg Theorem - HL Postulate:

Detour Proofs - Double Triangle Congruence:

___________________________________
The Midpoint Formula:

Missing Diagrams - 2 Column Proofs:

Right Angle Theorem - SSS & SAS:

Altitude, Median, Midpoint & P. Bisector:

Parallel & Perpendicular Lines - Slope:

__________________________________
Indirect Proofs - Example Problems:

Proving Parallel Lines With Proofs:

The Exterior Angle Theorem:

Exterior Angle Inequality Theorem:

Polygons:

_______________________________________
Final Exams and Video Playlists:

Full-Length Videos and Worksheets:

Рекомендации по теме

Комментарии

IT's actually helpful thanks you for being there for us I'm really happy to keep going

lovelylovelyabunchlovely

MR. Organic Chemistry Tutor, thank you for an incredible video/lecture on the Perpendicular Bisector of a Line Segment and Triangle in Geometry. The discussion of this topis is clear and to the point, however more examples could help for a solid understanding of the theory.

georgesadler

For the last question I believe there was actually three ways you could've proven the triangles to be congruent. It could've been the SSS Postulate, the SAS Postulate, and the HL Postulate.

Edit: I also believe you could've proved the statement "AB = CB" with the Perpendicular Bisector Theorem since B is a point on the perpendicular bisector, making it equidistant from A to C.

PurpleABlueAPlays

sir I know its not the object but I have a problem in hnmr

يوسفشعبان-وو

At 16:17, should it be line AB is congruent to line AC (vs. line CB)? A lies on the perpendicular bisector, but C does not. Am I wrong?

Kriffle

the perpendiculary of the length of the time of a video does not affect the conclusion statement whether or not the photosynthesis goes through the diameter

creproductions