filmov
tv
Normal Distribution - Explained Simply (part 1)
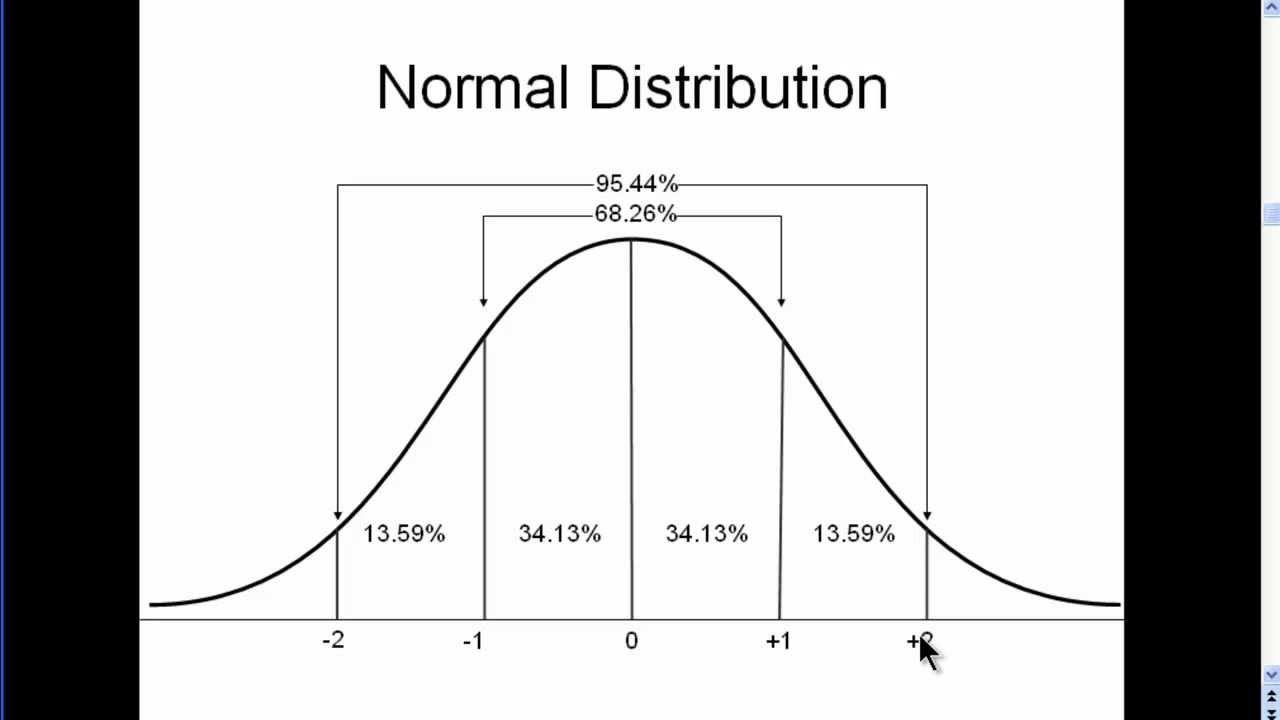
Показать описание
I describe the standard normal distribution and its properties with respect to the percentage of observations within each standard deviation. I also make reference to two key statistical demarcation points (i.e., 1.96 and 2.58) and their relationship to the normal distribution. Finally, I mention two tests that can be used to test normal distributions for statistical significance.
normal distribution, normal probability distribution, standard normal distribution, normal distribution curve, bell shaped curve
The Normal Distribution, Clearly Explained!!!
Normal Distribution EXPLAINED with Examples
Normal Distribution - Explained Simply (part 1)
The Bell Curve (Normal/Gaussian Distribution) Explained in One Minute: From Definition to Examples
Normal Distribution - Explained Simply (Improved Version!)
The Normal Distribution and the 68-95-99.7 Rule (5.2)
The Normal Distribution Explained Simply
Z-Scores, Standardization, and the Standard Normal Distribution (5.3)
Statistics for Data Science_II Live Stream
The Normal (Gaussian) Distribution - Clearly Explained
Normal Distribution - Explained Simply (part 2)
Normality test [Simply Explained]
Normal distribution - Intro to Psychology
Normal Distribution - Explained Simply (part 1)
Normal Distribution and z Scores Explained - Introductory Statistics
Normal Distribution & Probability Problems
Understanding the normal distribution - statistics help #Statistics #Probability
Standard Normal Distribution Tables, Z Scores, Probability & Empirical Rule - Stats
z-Score, z-Standardization, Standard Normal Distribution, z-Distribution Table - Simply explained
An Introduction to the Normal Distribution
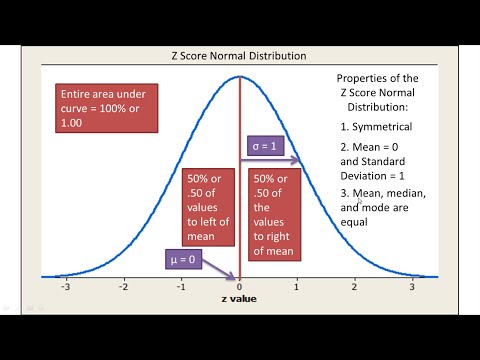
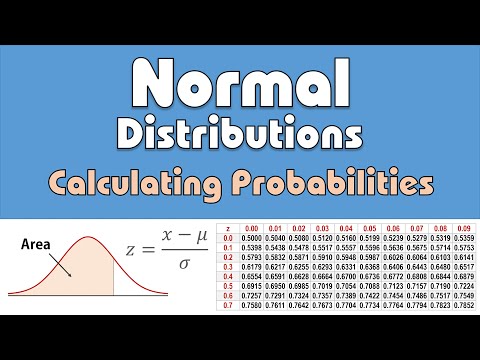
Normal Distribution: Calculating Probabilities/Areas (z-table)
Intro to the Normal Distribution and how to Calculate Probability
The Central Limit Theorem, Clearly Explained!!!
What is Normal Distribution in Statistics ? How to solve Normal (Gaussian) distribution problems ?
Комментарии
 0:05:13
0:05:13
 0:10:59
0:10:59
 0:05:04
0:05:04
 0:01:04
0:01:04
 0:10:46
0:10:46
 0:08:50
0:08:50
 0:06:14
0:06:14
 0:06:57
0:06:57
 2:13:42
2:13:42
 0:05:10
0:05:10
 0:04:25
0:04:25
 0:07:52
0:07:52
 0:01:32
0:01:32
 0:13:51
0:13:51
 0:03:28
0:03:28
 0:29:30
0:29:30
 0:07:39
0:07:39
 0:51:03
0:51:03
 0:14:08
0:14:08
 0:05:27
0:05:27
 0:05:21
0:05:21
 0:12:11
0:12:11
 0:07:35
0:07:35
 0:12:35
0:12:35