filmov
tv
GRAVITATION| INPHO 2013 Q6| RADIAL OSCILLATIONS OF ORBITS| ORBIT STABILITY UNDER CENTRAL FIELDS..

Показать описание
In This Video I have Presented My Analysis of INPHO 2013 Problem 6. This Problem requires one to find the time period of radial oscillations of planetary orbits and also finding the general condition of stability for orbits under central force motion. Hope you will enjoy my analysis....
Please do like, share and subscribe...
As you know that planets move in elliptical orbits under action of gravity of Sun. Suppose we assume that orbit is circular of radius ro and planet is slightly disturbed from its position such that its position is r = ro+δ where δ/ro much less than 1. Show that planet will oscillate simple harmonically around mean position ro. Obtain time period (Tr) for these radial oscillations. Given mass of sun is M and gravitational constant is G.
For this part we assume that planet moves under the force expressible as a power of r as F=-crn where c (c 0) is a constant. For what values of n a stable orbit is possible? (Orbit may not necessarily be nearly circular).
Please do like, share and subscribe...
As you know that planets move in elliptical orbits under action of gravity of Sun. Suppose we assume that orbit is circular of radius ro and planet is slightly disturbed from its position such that its position is r = ro+δ where δ/ro much less than 1. Show that planet will oscillate simple harmonically around mean position ro. Obtain time period (Tr) for these radial oscillations. Given mass of sun is M and gravitational constant is G.
For this part we assume that planet moves under the force expressible as a power of r as F=-crn where c (c 0) is a constant. For what values of n a stable orbit is possible? (Orbit may not necessarily be nearly circular).
Комментарии
 0:16:07
0:16:07
 0:15:28
0:15:28
 1:11:18
1:11:18
 1:48:36
1:48:36
 0:16:56
0:16:56
 0:16:24
0:16:24
 0:06:28
0:06:28
 0:13:46
0:13:46
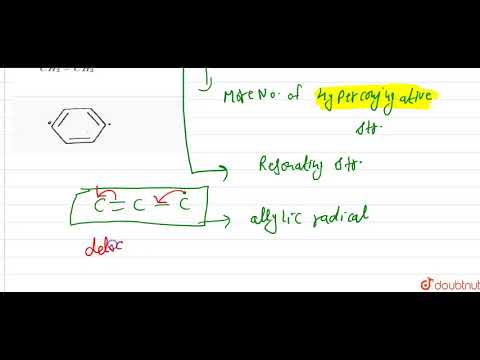 0:04:45
0:04:45
 0:06:51
0:06:51
 0:49:20
0:49:20
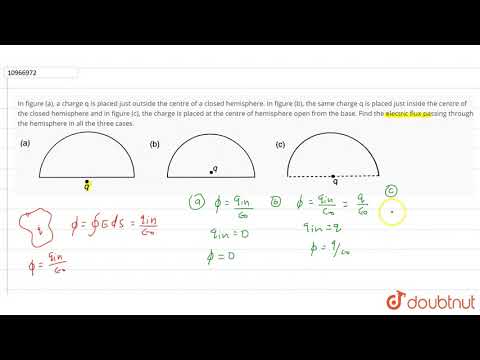 0:03:11
0:03:11
 0:21:15
0:21:15
 0:31:41
0:31:41
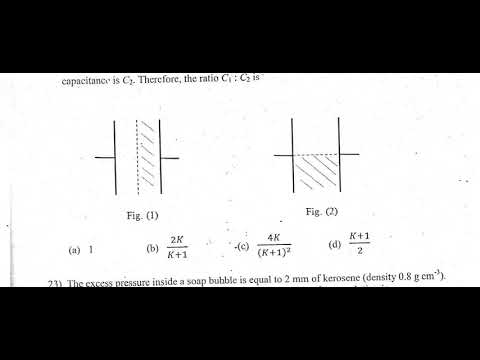 0:13:30
0:13:30
 0:05:49
0:05:49
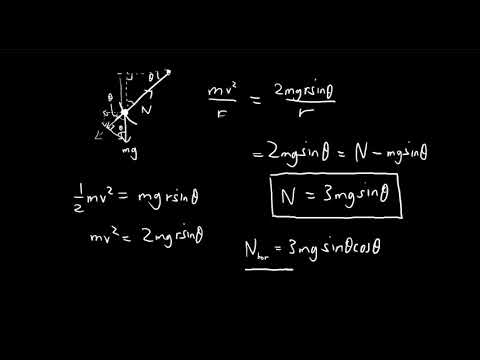 0:08:16
0:08:16