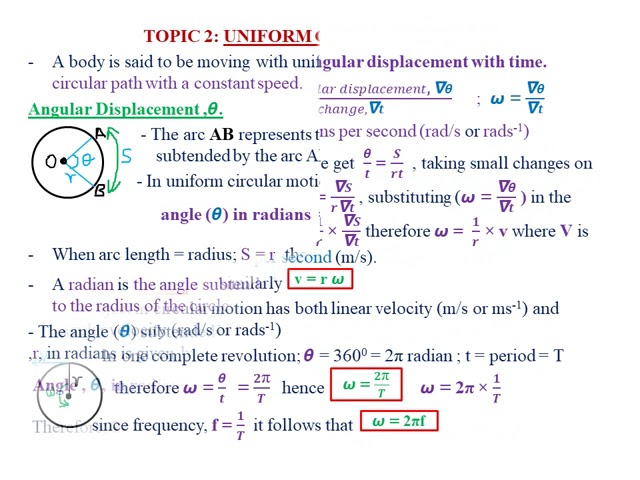
TOPIC 2: UNIFORM CIRCULAR MOTION: LESSON 1

#UNIFORM #CIRCULAR #MOTION #ANGULAR #DISPLACEMENT #VELOCITY #RADIAN #DISTANCE #LINEAR #SPEED #METRE #SECOND #PERIOD #FREQUENCY #OMEGA #TIME #REVOLUTION #HERTZ #Hz #PHYSICS #KCSE #IGCSE

[tiffanyasu]

He has the best explained video about this topic and cover all the formulas God bless u man

[JoséAntonioBottino]

Many people wonder why radians do not appear when we have radians*meters (rad • m).
Here is an attempt at an explanation:

Let s denote the length of an arc of a circle whose radius measures r.

If the arc subtends an angle measuring β = n°, we can pose a rule of three:
360° 2 • 𝜋 • r
n° s

Then
s = (n° / 360°) • 2 • 𝜋 • r

If β = 180° (which means that n = 180, the number of degrees), then
s = (180° / 360°) • 2 • 𝜋 • r

The units "degrees" cancel out and the result is
s = (1 / 2) • 2 • 𝜋 • r
s = 𝜋 • r

that is, half of the circumference 2 • 𝜋 • r.

If the arc subtends an angle measuring β = θ rad, we can pose a rule of three:
2 • 𝜋 rad 2 • 𝜋 • r
θ rad s

Then
s = (θ rad / 2 • 𝜋 rad) • 2 • 𝜋 • r

If β = 𝜋 rad (which means that θ = 𝜋, the number of radians), then
s = (𝜋 rad / 2 • 𝜋 rad) • 2 • 𝜋 • r

The units "radians" cancel out and the result is
s = (1 / 2) • 2 • 𝜋 • r
s = 𝜋 • r

that is, half of the circumference 2 • 𝜋 • r.

If we take the formula with the angles measured in radians, we can simplify
s = (θ rad / 2 • 𝜋 rad) • 2 • 𝜋 • r
s = θ • r

where θ denotes the "number of radians" (it does not have the unit "rad").
θ = β / (1 rad)

and θ is a dimensionless variable [rad/rad = 1].

However, many consider θ to denote the measure of the angle and for the example believe that
θ = 𝜋 rad

and radians*meter results in meters
rad • m = m

since, according to them, the radian is a dimensionless unit. This solves the problem of units for them and, as it has served them for a long time, they see no need to change it. But the truth is that the solution is simpler, what they have to take into account is the meaning of the variables that appear in the formulas, i.e. θ is just the number of radians without the unit rad.

Mathematics and Physics textbooks state that
s = θ • r

and then
θ = s / r

It seems that this formula led to the error of believing that
1 rad = 1 m/m = 1

and that the radian is a dimensionless derived unit as it appears in the International System of Units (SI), when in reality
θ = 1 m/m = 1

and knowing θ = 1, the angle measures β = 1 rad.

In the formula
s = θ • r

the variable θ is a dimensionless variable, it is a number without units, it is the number of radians.

When confusing what θ represents in the formula, some mistakes are made in Physics in the units of certain quantities, such as angular speed.

My guess is that actually the angular speed ω is not measured in rad/s but in
(rad/rad)/s = 1/s = s^(-1).

[dddm]

Thanks 🙏🙏🙏 mwalimu waiting for the next

[jjkoriginal]

Great, highly predicted topic this year

[hasannor]

Keep going mwalimu. My team are keenly following you. Each day you post new video, i notify them. Some of my students miss one or two lessons, am telling them to benefit from you.

[MuochLam-vc]

Really appreciate mwalimu God bless 🎉🎉🎉

[SychKings]

Mwalimu you assist the last(3) question you have given as an exercise on the uniform circular motion.

[JoséAntonioBottino]

Starting at minute 1:59 you talk about “angular displacement, θ”. You state that the “angles are measured in radians”. This is not so. The variable θ refers to the number of radians, it is a dimensionless variable, and its unit is rad/rad = 1.

We can also read that "When arc length = radius: S = r then
θ = S / r = 1 radian".

This is the same as what the majority of the scientific community believes and the International System of Units (SI) says when it states that the radian is a dimensionless unit and that in terms of base units
1 rad = 1 m/m = 1.

They are wrong. The reality is that when S = r, then
θ = S / r = 1

and the angle measures
β = θ rad
β = 1 rad

using for the angle measure the variable β.

You state that “A radian is the angle subtended at the center of a circle by an arc length equal to the radius of the circle”. The radian is not an angle, but a unit of measurement of angles. Sometimes the distinction is not made, but in this case it is necessary.

Starting at minute 9:24 you speak of the “angular velocity, ω”. You state that
ω = Δθ / Δt

and that “Angular velocity is measured in radians per second (rad/s or rad - s^(-1))”. Also most of the scientific community and the SI believe this to be the case, but again they are wrong. It should actually be
(rad/rad)/s = 1/s = s^(-1).


Also you show the relationship
v = ω • r

Here we can see the compatibility of units. If we use the units shown in the video
m/s = (rad/s) • m

and the radian is not clear. The explanation usually given is that the radian is a dimensionless unit and is placed or not conveniently. This is not true, the reality is that the units are
m/s = [(rad/rad)/s] • m
m/s = (1/s) • m
m/s = m/s.

In the example starting at minute 17:29 you say “we know that frequency is simply the number or revolutios made in one second”, and in calculating the frequency you say that it is equal to
f = 0.75 (1/s)

and you calculate the angular velocity
ω = 2 • 𝜋 • f
ω = 2 • 3.142 • [0.75 (1/s)]
ω = 4.713 rad/s.

Again the radian appears, only because the units of the variables are established. The explanation of the dimensionless radian returns.

The reality is that the calculations with the units are as follows
f = 0.75 (rev/rev)/s

and the number of revolutions has unit rev/rev
f = 0.75 Hz
f = 0.75 (1/s)

and the angular velocity is
ω = 2 • 𝜋 • f
ω = 2 • 3.142 • [0.75 (rev/rev)/s]
ω = 4.713 (rad/rad)/s
ω = 4.713 (1/s).

There the 2𝜋 allows us to go from “number of revolutions” (rev/rev) to “number of radians” (rad/rad).

I am going to write two more comments. In the first one I will try to clarify the Uniform Circular Motion and in the second one how to obtain the formula
s = θ • r

and what the variables represent, especially θ.

[JoséAntonioBottino]

In a Uniform Circular Motion, the linear speed (tangential speed) v remains constant.

If the object makes n revolutions (cycles) in a time t, then it travels a distance s
s = 2 • 𝜋 • r • n

where n is the “number of revolutions”, n is dimensionless, n has unit rev/rev = 1.

Since v = s / t, then
v = (2 • 𝜋 • r • n) / t

Since v = ω • r, then
ω • r = (2 • 𝜋 • r • n) / t.

This implies that
ω = (2 • 𝜋 • n) / t

If ω = 2 • 𝜋 • f, where f is the frequency, then.
2 • 𝜋 • f = (2 • 𝜋 • n) / t.

This implies that
f = n / t

or what is the same, the frequency f is the number of revolutions (cycles) per unit time (usually seconds).

The unit of f should be
(rev/rev)/s = Hz = 1/s

equal to the number of revolutions per second [nrps = (rev/rev)/s, if the custom is to be maintained], and not in revolutions per second (rps = rev/s).

The unit hertz (Hz) replaced the unit cycles per second, which was actually the number of cycles per second.

Given that the period T = 1 / f, then
T = t / n.

Since the period T is the time it takes for the object to complete one revolution (one cycle), then the unit of T is:
s/(rev/rev) = s

equal to seconds per number of revolutions (second per number of cycles).

As
ω = θ / t

and θ is the number of radians, θ is dimensionless, θ is measured in rad/rad = 1, that means that ω must be measured in
(rad/rad)/s = 1/s = s^(-1)

and not in rad/s.

It is understood that in the formula
ω = 2 • 𝜋 • f

the unit conversion is
1 (rad/rad)/s = 2 • 𝜋 • (rev/rev)/s

so
1 (rad/rad) = 2 • 𝜋 • (rev/rev).

There the 2𝜋 allows us to go from “number of revolutions” (rev/rev) to “number of radians” (rad/rad).

I will highlight the difference between the unit of angular speed, which seems to be 1/s and the unit of frequency which also appears to be 1/s. They are different. Hertz is number of revolutions per second (nrps) while angular speed is the number of radians per second (nrad/s, stretching the notation a bit).

I will leave another comment where I show how to obtain the formula
s = θ • r

and what the variables represent.

[m.g]

Thanx mwalimu for your good work. sir in your playlist, all form 4 lessons are not there.

[redimadaya]

Kindly sir can u help me in specific topics please

[margaretgichuki]

Hey mwalimu, , , , where can I kindly get your notes.

[fridaharithi]

Pliz help in the last question of the exercise thanks in advance

[Maskedsinging-zl]

I have a problem with the last question number 3

[Sir-AJ-]

If possible please work on pp2 especially form 4 work be4 the end of this year

[LewisMburu-vhzx]

kindly assist me to do the last question

[margaretgichuki]

Hey mwalimu, , , , where can I kindly get your notes.