filmov
tv
The principle of least action
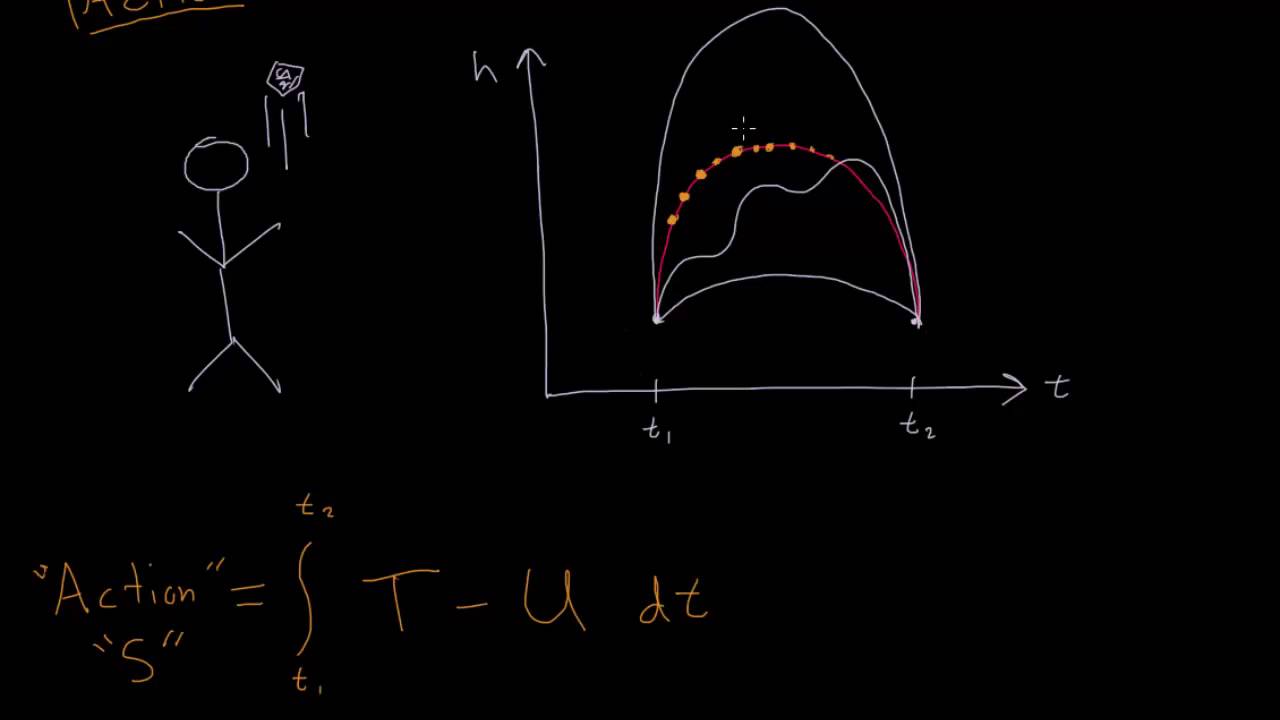
Показать описание
Here's a qualitative introduction to another way of looking at physics.
Explaining the Principle of Least Action: Physics Mini Lesson
The principle of least action
The Principle of Least Action: Derivation of Newton's Second Law
Is ACTION The Most Fundamental Property in Physics?
Physics 70 Principle of Least Action (1 of 27) What is the Principle of Least Action?
Your Daily Equation #19 : At the Core of Fundamental Physics: The Principle of Least Action
Lagrangian Mechanics - A beautiful way to look at the world
The Closest We Have to a Theory of Everything
Principles of Financial Freedom | Jim Rohn Powerful Motivational Speech
The Beauty of Physics - The Principle of Least Action
Physics 70 Principle of Least Action (2 of 27) Why the Principle of Least Action?
Quantum Mechanics and the Principle of Least Time
The Most Important Idea in Physics: The Principle of Least Action - Ask a Spaceman!
Lagrangian Mechanics: How powerful is it?
Euler-Lagrange equation explained intuitively - Lagrangian Mechanics
Physics in 10 minutes - The principle of least action
Physics 70 Principle of Least Action (19 of 27) The Principle Derived (Part 1/4)
Physics 70 Principle of Least Action (11 of 27) Practical Application?
Principle of Least Action | Classical Mechanics | LetThereBeMath |
QED Prerequisites Principle of Least Action: Introduction
Principle of Least Action
The Principle of Least Action - In Action
What is the Principle of Least Action? Alas Lewis & Barnes
PRINCIPLE OF LEAST ACTION || STATEMENT AND PROOF || CLASSICAL MECHANICS || WITH EXAM NOTES ||
Комментарии
 0:17:55
0:17:55
 0:10:51
0:10:51
 0:24:30
0:24:30
 0:19:40
0:19:40
 0:04:12
0:04:12
 0:36:06
0:36:06
 0:12:26
0:12:26
 0:13:28
0:13:28
 1:21:40
1:21:40
 0:02:45
0:02:45
 0:04:05
0:04:05
 0:24:53
0:24:53
 0:17:14
0:17:14
 0:10:01
0:10:01
 0:18:22
0:18:22
 0:10:26
0:10:26
 0:05:56
0:05:56
 0:03:59
0:03:59
 0:10:15
0:10:15
 0:48:52
0:48:52
 0:09:45
0:09:45
 0:09:51
0:09:51
 0:15:19
0:15:19
 0:23:23
0:23:23