filmov
tv
Berkeley Math Tournament calculus tiebreaker
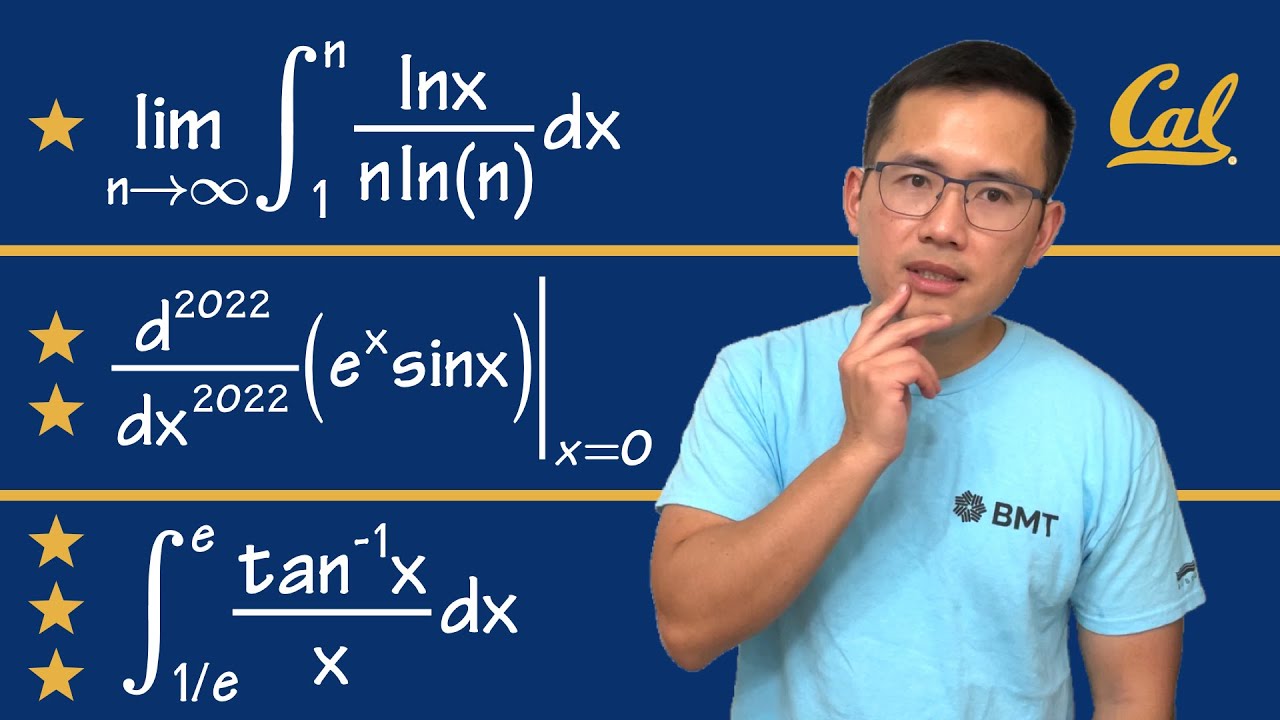
Показать описание
This calculus tutorial contains the solution to the 2022 Berkeley Math Tournament calculus tiebreaker! It contains a limit, derivative, and an integral at calculus 2 (i.e. AP calculus BC) level. The time limit is 15 minutes but you will also have to race with other times during the tournament. I love these math competition problems because they really force you to think and they really challenge your problem-solving skills. It's okay if you can't solve these competition problems. I, too, can't solve many of them either. It really takes lots of effort and practice in order to be good at these. Finally, I just want to tell you, to be sure to enjoy the learning process and have fun!
0:00 2022 Berkeley Math Tournament calculus tiebreaker
0:08 Q1, evaluate a limit with an integral
3:10 Q2, find the 2022nd derivative of f(x)=e^x*sin(x) at x=0
7:36 Q3, evaluate the integral of arctan(x)/x from 1/e to e
#calculus #tutorial #berkeley #blackpenredpen
----------------------------------------
AP-IP Ben Delo Marcelo Silva Ehud Ezra 3blue1brown Joseph DeStefano
Mark Mann Philippe Zivan Sussholz AlkanKondo89 Adam Quentin Colley
Gary Tugan Stephen Stofka Alex Dodge Gary Huntress Alison Hansel
Delton Ding Klemens Christopher Ursich buda Vincent Poirier Toma Kolev
Tibees Bob Maxell A.B.C Cristian Navarro Jan Bormans Galios Theorist
Robert Sundling Stuart Wurtman Nick S William O'Corrigan Ron Jensen
Patapom Daniel Kahn Lea Denise James Steven Ridgway Jason Bucata
Mirko Schultz xeioex Jean-Manuel Izaret Jason Clement robert huff
Julian Moik Hiu Fung Lam Ronald Bryant Jan Řehák Robert Toltowicz
Angel Marchev, Jr. Antonio Luiz Brandao SquadriWilliam Laderer Natasha Caron Yevonnael Andrew Angel Marchev Sam Padilla ScienceBro Ryan Bingham
Papa Fassi Hoang Nguyen Arun Iyengar Michael Miller Sandun Panthangi
Skorj Olafsen Riley Faison Rolf Waefler Andrew Jack Ingham P Dwag Jason Kevin Davis Franco Tejero Klasseh Khornate Richard Payne Witek Mozga Brandon Smith Jan Lukas Kiermeyer Ralph Sato Kischel Nair Carsten Milkau Keith Kevelson Christoph Hipp Witness Forest Roberts Abd-alijaleel Laraki Anthony Bruent-Bessette Samuel Gronwold Tyler Bennett christopher careta Troy R Katy Lap C Niltiac, Stealer of Souls Jon Daivd R meh Tom Noa Overloop Jude Khine R3factor. Jasmine Soni L wan na Marcelo Silva Samuel N Anthony Rogers Mark Madsen Robert Da Costa Nathan Kean Timothy Raymond Gregory Henzie Lauren Danielle Nadia Rahman Evangline McDonald Yuval Blatt Zahra Parhoun Hassan Alashoor Kaakaopuupod bbaa Joash Hall Andr3w11235 Cadentato Joe Wisniewski Eric Maximilian Mecke Jorge Casanova Alexis Villalobos Jm Law Siang Qi Tancredi Casoli Steven Sea Shanties Nick K Daniel Akheterov Roy Logan
----------------------------------------
Thank you all!
Комментарии
 0:14:24
0:14:24
 0:10:20
0:10:20
 0:03:47
0:03:47
 0:04:24
0:04:24
 0:07:03
0:07:03
 0:10:13
0:10:13
 0:22:28
0:22:28
 0:04:56
0:04:56
 0:14:50
0:14:50
 0:04:45
0:04:45
 0:14:11
0:14:11
 0:06:21
0:06:21
 0:03:14
0:03:14
 0:21:26
0:21:26
 0:02:11
0:02:11
 0:01:23
0:01:23
 0:04:17
0:04:17
 0:00:15
0:00:15
 0:06:22
0:06:22
 0:16:15
0:16:15
 0:06:59
0:06:59
 0:07:58
0:07:58
 0:11:12
0:11:12
 0:07:07
0:07:07