Integral of e^x*sqrt(1 - e^(2x)) using Trigonometric Substitution

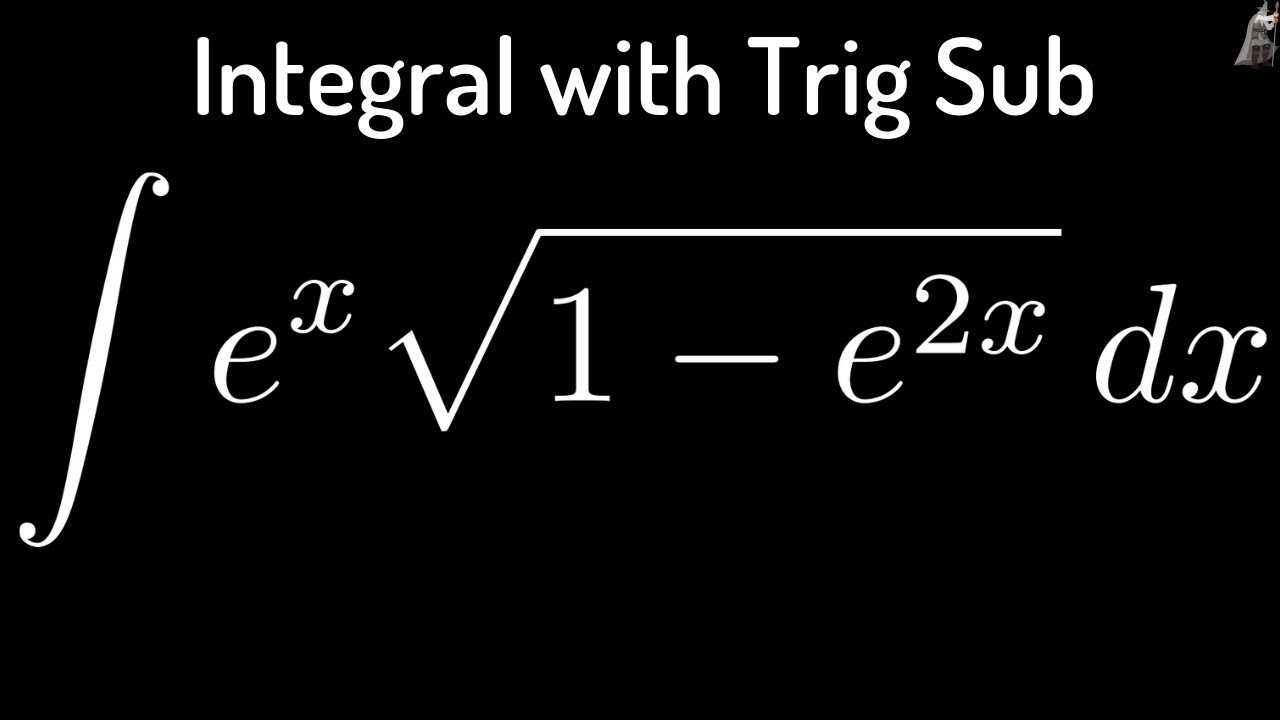
preview_player
Показать описание
Integral of e^x*sqrt(1 - e^(2x)) using Trigonometric Substitution
  1. The Math Sorcerer
  2. Integration By Substitution
  3. Trigonometric Substitution
  4. Exponential Function
  5. integral
  6. integrate
Рекомендации по теме
Integral of e^x*sqrt(1 0:06:16

Integral of e^x*sqrt(1 - e^(2x)) using Trigonometric Substitution

Integral of e^x/sqrt(1 0:03:43

Integral of e^x/sqrt(1 - e^2x)

Integral e^x/sqrt(1-e^(2x)), informal 0:02:42

Integral e^x/sqrt(1-e^(2x)), informal approach (chain rule backwards) vs. u-substitution.

Evaluate the Integral 0:01:55

Evaluate the Integral e^x sqrt(1 + e^x) dx. Natural exponential function

Integral e^(2x)*sqrt(1-e^(2x)) using 0:04:44

Integral e^(2x)*sqrt(1-e^(2x)) using the chain rule backwards vs. explicit substitution approach.

100 integrales por 0:05:17

100 integrales por sustitución o cambio de variable ejemplo 32 integral de (e^x)/(sqrt(1-e^{2x))}

Integral of Sqrt(e^2x 0:13:20

Integral of Sqrt(e^2x + 1) dx - This one is fun!

The Integral of 0:09:16

The Integral of e^x /sqrt(1 - e^2x)

Ramblings from Calculus 0:41:06

Ramblings from Calculus 1 before Exam 3 (Fall 2024)

Integral of e^2x/sqrt(e^(2x)+1) 0:04:46

Integral of e^2x/sqrt(e^(2x)+1)

Integrating Exponential Functions 0:11:16

Integrating Exponential Functions By Substitution - Antiderivatives - Calculus

Evaluate the Integral 0:02:31

Evaluate the Integral e^(2x)/sqrt(1- e^(4x)) dx. Inverse Trig Functions

Integral of (e^2x)/(e^x+1) 0:02:56

Integral of (e^2x)/(e^x+1)

∫ (e^x)/(1+e^2x) dx 0:00:40

∫ (e^x)/(1+e^2x) dx #calculus #shorts #integration #integrationclass12

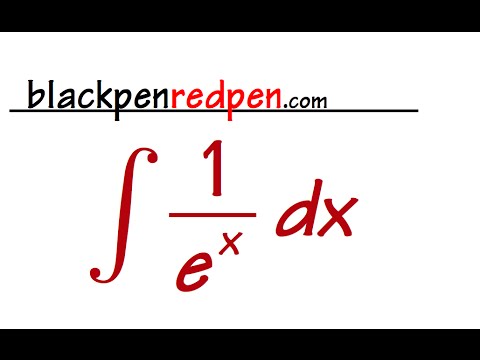
Integral of 1/e^x 0:00:41

Integral of 1/e^x

Integrales | 100 0:07:58

Integrales | 100 Integrales por Sustitución o CV 47 - integral de (e^{2x})/(sqrt(e^x+1))dx

Evaluate the Definite 0:03:00

Evaluate the Definite Integral with the Exponential Function e^(-2x)

Integral of sqrt(1+e^x) 0:03:37

Integral of sqrt(1+e^x) - Integral example

The Integral of 0:07:03

The Integral of sqrt(e^2x - 1)

5. Integrales por 0:09:46

5. Integrales por sustitución trigonométrica - Integral de ∫ e^x √(1-e^2x) dx

Integral of e^sqrt(x) 0:05:43

Integral of e^sqrt(x)

`int(dx)/(sqrt(1-e^(2x)))=?` 0:03:34

`int(dx)/(sqrt(1-e^(2x)))=?`

`int(dx)/(sqrt(1-e^(2x)))` is equal 0:01:49

`int(dx)/(sqrt(1-e^(2x)))` is equal to

Learn how to 0:01:55

Learn how to integrate e^(2x)

Комментарии
Автор

This problem covered a lot of calculus i've learned, Very cool problem!

NaftaliSpodek
Автор

Dear Math Sorcerer,

Was just wondering: after using power reduction angle identity to convert cos^2x into 1/2 +1/2cos2x why do we have to use double angle identity to change the "pesky" 1/2 cos2x into 2sinxcosx before plugging in values from triangle for our final solution?

Can we not plug in values from triangle and just multiply by two?

Btw your exercises are awesome and very helpful, thank you!

AmirJacob_
Автор

Thanks for the solution. I made y=e^x and the problem simplified to int(sqrt(1-y^2))dy and got the same result.

cesarvillegas
Автор

0:50 - how did you differentiate that? implicit differentiation? i'm confused. wouldn't you need to differentiate both sides with respect to theta, giving:
d (e^x) / dθ = cos(θ)
d e^x = cos(θ)dθ
my answer doesn't really make sense to myself, i'm just so confused

tb
Автор

Why didn't you substitute the dx?

josemanuelsanchezmunoz
Автор

But what if you have a 5 instead of the 1???

leahrussinko
Автор

How e^2x=(e^x)^2 ?
Can any one help plz

VaibhaviPhadtare-dspl
Автор

How is e^x = sinø or even u=asinø?? You lost me there.

menzihxni