filmov
tv
Visual Inclusion/Exclusion

Показать описание
In this video, we show how to begin to deal with the general Sum principle for Sets when you have more than two sets involved. Can you see how to keep extending this idea for more and more sets?
#logic #settheory #inclusion/exclusion #intersection #setdifference #setminus #setconnectives #subsets #venndiagram #visualproof #math #manim #discretemathematics #sumprinciple
To learn more about animating with manim, check out:
#logic #settheory #inclusion/exclusion #intersection #setdifference #setminus #setconnectives #subsets #venndiagram #visualproof #math #manim #discretemathematics #sumprinciple
To learn more about animating with manim, check out:
Visual Inclusion/Exclusion
Three Set Inclusion/Exclusion (visual proof) [Discrete Math Class]
S07.1 The Inclusion-Exclusion Formula
Inclusion/Exclusion
Inclusion-Exclusion Part 1 (full lecture)
Visualizing Inclusion Exclusion Principle in Probability!
Set Theory. Inclusion-exclusion Principle.
3-way Venn diagram, principle of inclusion-exclusion
Inclusion Exclusion Principle
Inclusion Exclusion Principle for 3 sets #shorts #matematika
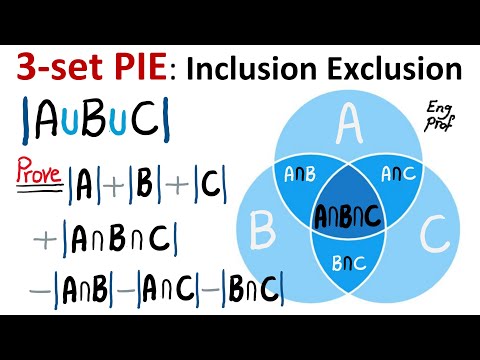
3-set PIE: Principle of Inclusion Exclusion quick proof
Application of Inclusion/Exclusion with 3 Sets
Lecture 5: i. Inclusion–exclusion for three events — [Probability | Santosh S. Venkatesh]
Inclusion-Exclusion.
Do This to understand the Inclusion-Exclusion principle in 2 minutes
Inclusion Exclusion Principle C++
Discrete Math II - 8.5.1 The Principle of Inclusion-Exclusion
The Inclusion-Exclusion Principle
Discrete Math II - 8.5.1 The Principle of Inclusion Exclusion
Inclusion - Exclusion Principle: Application
The inclusion exclusion principle for 2 and 3 sets
Inclusion Makes the World More Vibrant
inclusion-exclusion exercise
Venn Diagrams and Inclusion-Exclusion Principle - Manim/Python
Комментарии
 0:00:57
0:00:57
 0:02:11
0:02:11
 0:11:13
0:11:13
 0:05:37
0:05:37
 0:28:35
0:28:35
 0:04:54
0:04:54
 0:05:13
0:05:13
 0:05:00
0:05:00
 0:07:32
0:07:32
 0:00:17
0:00:17
 0:07:38
0:07:38
 0:02:48
0:02:48
 0:04:49
0:04:49
 0:10:37
0:10:37
 0:02:30
0:02:30
 0:16:59
0:16:59
 0:20:49
0:20:49
 0:07:22
0:07:22
 0:20:12
0:20:12
 0:15:31
0:15:31
 0:11:43
0:11:43
 0:03:07
0:03:07
 0:02:26
0:02:26
 0:02:06
0:02:06