filmov
tv
Advanced Engineering Mathematics, Lecture 3.1: Fourier series and orthogonality
Показать описание
Advanced Engineering Mathematics, Lecture 3.1: Fourier series and orthogonality.
In this lecture, we revisit how to define an inner product on the space of 2π-periodic functions so that the set consisting of all cos(nx) and sin(mx) functions are pairwise orthogonal. Analogously to how we decompose a vector in R^n into components, this allows us to decompose an arbitrary 2π-periodic function into an sum of sine and cosine waves, which we call its Fourier series. The so-called Fourier coefficients are simply the inner products of the function with these individual trigonometric functions.
In this lecture, we revisit how to define an inner product on the space of 2π-periodic functions so that the set consisting of all cos(nx) and sin(mx) functions are pairwise orthogonal. Analogously to how we decompose a vector in R^n into components, this allows us to decompose an arbitrary 2π-periodic function into an sum of sine and cosine waves, which we call its Fourier series. The so-called Fourier coefficients are simply the inner products of the function with these individual trigonometric functions.
0:29:29
Advanced Engineering Mathematics, Lecture 3.1: Fourier series and orthogonality
0:41:08
Advanced Engineering Mathematics, Lecture 1.3: Linear maps
0:06:53
Chapter 1.5 Problem 3 (Advanced Engineering Mathematics)
0:37:03
Advanced Engineering Mathematics, Lecture 3.6: Real vs. complex Fourier series
0:14:35
KREYSZIG | Advanced Engineering Mathematics 10th edition | Problem set 14.1 Question 1 to 3.
0:25:44
Laplace Transform Introduction - Advanced Engineering Mathematics
1:07:50
KREYSZIG #6 | Advanced Engineering Mathematics - Kreyszig | Problem Set 1.3 | Problems 1 - 10
0:09:20
Kreyszig - Advanced Engineering Mathematics 10th Ed - Problem 1.1 Question 1-4
0:43:02
Engineering Mathematics 13 | Probability Distributions Part - 3 | GATE - For All Branches
0:00:48
MIT Entrance Exam Problem from 1869 #Shorts #math #maths #mathematics #problem #MIT
0:49:37
Advanced Engineering Mathematics, Lecture 3.7: Fourier transforms
0:58:54
Lecture # 3 || Introduction to Partial Differential Equations || Advanced Engineering Mathematics
0:31:48
Advanced Engineering Mathematics, Lecture 3.3: Solving ODEs with Fourier series
0:20:46
Evaluating Laplace Transform By Table Part 1 - Advanced Engineering Mathematics
0:01:00
Oxford student reacts to India’s JEE Advanced exam paper *really hard* #shorts #viral #jeeadvanced
0:40:32
Advanced Engineering Mathematics, Lecture 5.3: The transport and heat equations
0:00:16
This chapter closes now, for the next one to begin. 🥂✨.#iitbombay #convocation
0:05:12
Chapter 1.1 Problem 1 (Advanced Engineering Mathematics)
0:29:05
Complex Numbers Operations - Advanced Engineering Mathematics
0:11:06
The Map of Mathematics
0:38:56
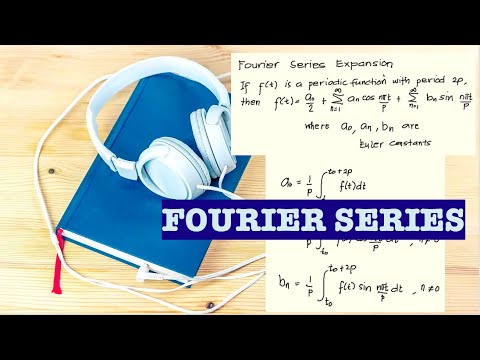
FOURIER SERIES | Advanced Engineering Math
0:00:27
Asking MIT students their major
0:12:49
Infinite Series | Advanced Engineering Mathematics
0:44:24
Advanced Engineering Mathematics, Lecture 2.5: Power series solutions to ODEs
Комментарии