filmov
tv
Proof: Bipartite Graphs have no Odd Cycles | Graph Theory, Bipartite Theorem, Proofs
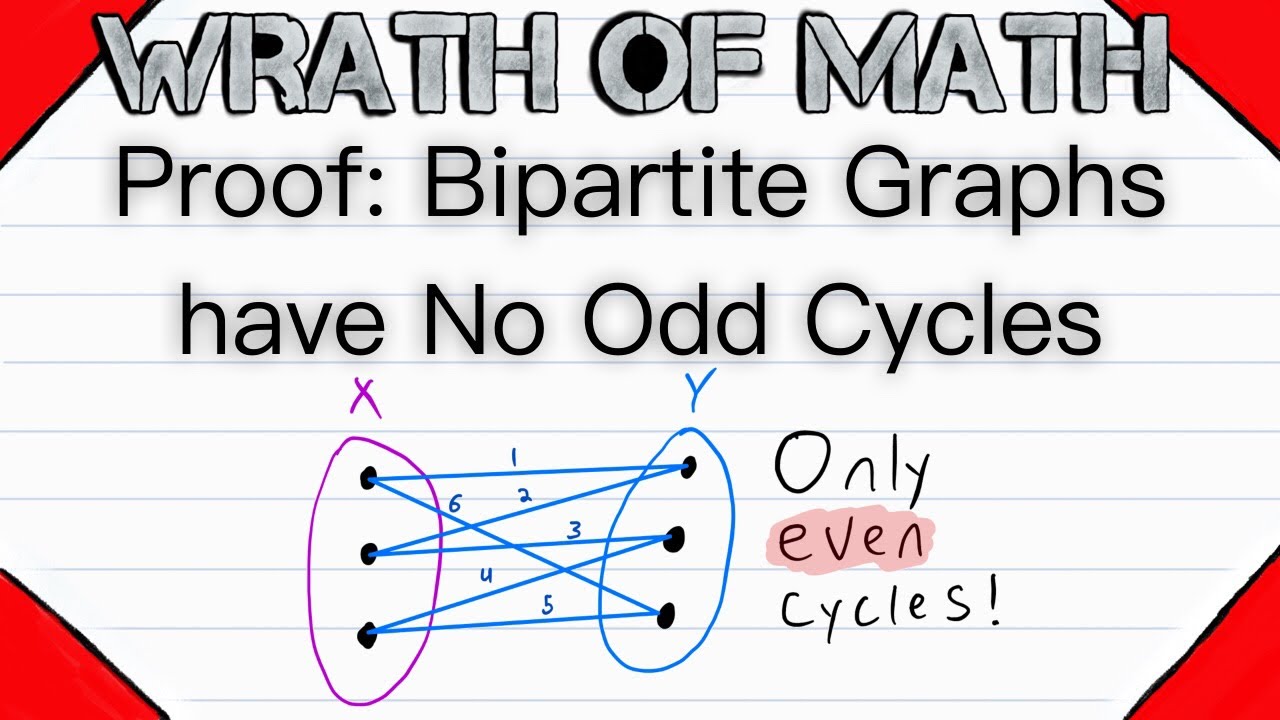
Показать описание
If a graph is bipartite then it has no odd cycles. This fact seems like it might not be obvious at first, but with a little bit of drawing and thinking, we can quickly demonstrate this result! In today's video graph theory lesson, we actually present two proofs of this result. The second proof uses contradiction, a favorite of mine! We'll be using the definition of bipartite graphs, and the definition of cycle, and it will lead us to our solution!
I hope you find this video helpful, and be sure to ask any questions down in the comments!
********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.
********************************************************************
+WRATH OF MATH+
Follow Wrath of Math on...
I hope you find this video helpful, and be sure to ask any questions down in the comments!
********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.
********************************************************************
+WRATH OF MATH+
Follow Wrath of Math on...
0:06:58
Proof: Bipartite Graphs have no Odd Cycles | Graph Theory, Bipartite Theorem, Proofs
0:20:05
Proof: If a Graph has no Odd Cycles then it is Bipartite | Graph Theory, Bipartite Theorem
0:08:55
How to Tell if Graph is Bipartite (by hand) | Graph Theory
0:09:53
Proof: A Graph or its Complement is not Bipartite | Graph Theory, Bipartite Graphs
0:05:17
What is a Bipartite Graph? | Graph Theory
0:25:13
Graph Theory: 19. Graph is Bipartite iff No Odd Cycle
0:05:50
Every Tree Graph is Bipartite | Graph Theory
0:09:32
Bipartite Graphs with Isolated Vertices | Graph Theory, Complete Bipartite Graphs
0:55:38
Extremal Combinatorics Lecture 12: Mantel's Theorem
0:07:58
A characterization of bipartite graphs
0:36:14
If Graph has no odd cycle then it is bipartite
0:22:22
Proof: Bipartite Graph have No odd cycles
0:02:46
Proof a graph is bipartite if and only if it contains no odd cycles (4 Solutions!!)
0:10:00
If Graph is bipartite then it contains no odd cycle
0:07:55
What are Complete Bipartite Graphs? | Graph Theory, Bipartite Graphs
0:08:58
How to check whether a given graph is Bipartite graph or not?
0:21:42
CG6 Bipartite Graphs & their Cycles
0:05:43
Proof: Regular Bipartite Graph is Balanced | Graph Theory, Partite Sets
0:16:23
Example: Proving a graph has no Hamilton cycle
0:09:35
A Proof on Hamiltonian Complete Bipartite Graphs | Graph Theory, Hamiltonian Graphs
0:07:24
Proof: Regular Bipartite Graph has a Perfect Matching | Graph Theory
0:49:29
Lecture 14b Bipartite Graphs
0:13:11
A graph is bipartite if and only if it contains no odd cycles. (Part 1)
0:11:17
Is Graph Bipartite? - Leetcode 785 - Python
Комментарии