filmov
tv
Find ∠ BAC. || Angle Chasing.|| ∠BAE = ∠ADC.|| BE : EC = 2 : 1. || Triangles with common vertex.

Показать описание
In this video clip, we have to find the value of ∠ BAC. It is an angle chasing problem.
In a ∆ ABC, D is the mid point of AB. E is a point on BC such that BE : EC = 2 : 1. Given that ∠BAE = ∠ADC.
#Geometry
#Right_Triangle
#Triangles_with_common_vertex.
#Angle_Chasing_Problem
Useful for :
#NTSE, #GRE, #IITJEE, #IOQM, #RMO, #INMO, #ISI, #CMI, #MAT, #CAT, #SAT, #BITSAT, #PET, #CET, #MCA, #NDA, #CDS, #SSC, #KVPY, #RRB, etc...
In a ∆ ABC, D is the mid point of AB. E is a point on BC such that BE : EC = 2 : 1. Given that ∠BAE = ∠ADC.
#Geometry
#Right_Triangle
#Triangles_with_common_vertex.
#Angle_Chasing_Problem
Useful for :
#NTSE, #GRE, #IITJEE, #IOQM, #RMO, #INMO, #ISI, #CMI, #MAT, #CAT, #SAT, #BITSAT, #PET, #CET, #MCA, #NDA, #CDS, #SSC, #KVPY, #RRB, etc...
Learn to find the missing angles for a triangle using inverse trig functions
find angle bac in each figure class 7 | math problems solve | very easy method |
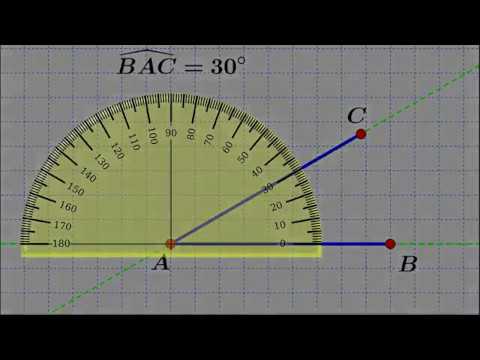
Angles - measuring the angle BAC - examples
Challenge Question: Can you find the Angle BAC?| Step-by-Step Explanation
In a triangle ABC, incentre is at O. Find the angle BAC if angle BOC = 110°.
AC=6 cm, AB=5 cm and angle BAC =30. Find the area of the triangle.
Which equation can be used to find the measure of angle bac
In figure, if AB || DE, ∠BAC = 35° and ∠CDE = 53°, find ∠DCE. #class9th #linesandangle #chapter6...
Class 9 Maths | Chapter 9 | Circles | Exercise 9.3 Q2 | NCERT
Ques-18. In the cyclic quadrilateral ABCD; AD=BC, angle BAC=30° and angle CBD = 70° ; find angle --...
Circles / how to find the angle BAC / #easy #maths #tutorial
In the adjoining figure, it is given that CE || BA, angle BAC = 80° and angle ECD = 35°. Find....
In the following figure AD bisects angle BAC. find the length of BD
Find m(∠BAC), if median AM, angle bisector AD and altitude AP quadrisect ∠BAC of a ∆ ABC || Geometry...
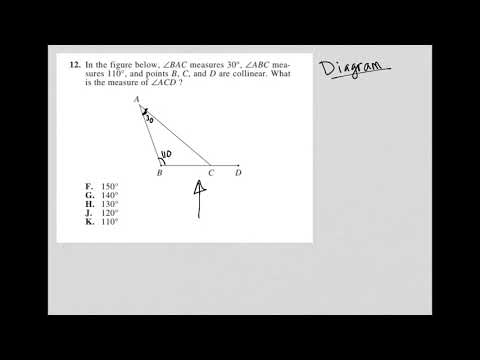
In the figure below, angle BAC measures 30 degrees, angle ABC measures 110 degrees, and points B, C
In a ∆ABC = AC and ∠ACD = 105°. Find ∠BAC.
AC=6 AB=5 and angle BAC =30⁰. Find the area of the triangle.
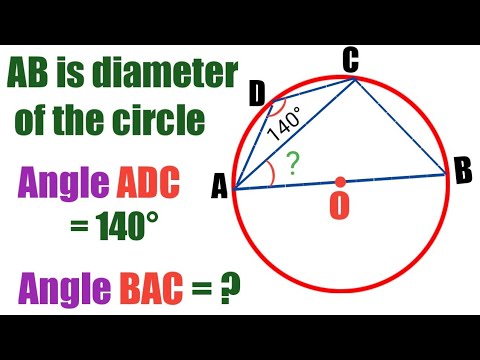
।। Geometry ।। angle ADC=140°, find the angle BAC @mathscuriosity494
Missing Angles Geometry Problem | Tricky Math Question | JusticeTheTutor #maths #math #shorts
(10) In the adjacent figure,AC=6cm,AB=5cm and angle BAC =30°.Find the area of the triangle.
AB||DE, angle BAC=35° and CDE=53°, Find angle DCE
Find ∠ BAC. || Angle Chasing.|| ∠BAE = ∠ADC.|| BE : EC = 2 : 1. || Triangles with common vertex....
In fig 6.41, if AB||DE, ∠ BAC = 35° and ∠ CDE = 53°, find ∠ DCE | Important [Q3]
In the given fig. AB = AC and DB = DC. if ∠BAC = 80° and ∠ACD = 15°. Find the measure of ∠DBC....
Комментарии
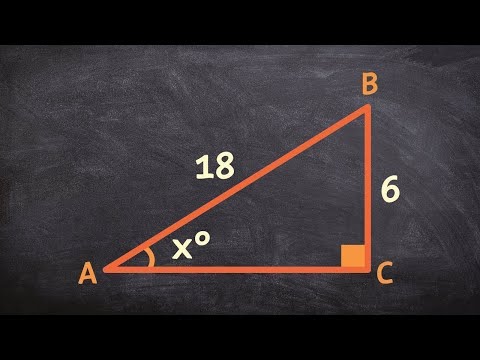 0:06:40
0:06:40
 0:02:48
0:02:48
 0:02:21
0:02:21
 0:15:00
0:15:00
 0:04:06
0:04:06
 0:03:53
0:03:53
 0:07:55
0:07:55
 0:01:48
0:01:48
 0:14:46
0:14:46
 0:07:55
0:07:55
 0:01:00
0:01:00
 0:12:36
0:12:36
 0:01:43
0:01:43
 0:09:45
0:09:45
 0:03:04
0:03:04
 0:07:42
0:07:42
 0:02:36
0:02:36
 0:04:16
0:04:16
 0:00:37
0:00:37
 0:06:42
0:06:42
 0:04:53
0:04:53
 0:06:18
0:06:18
 0:02:36
0:02:36
 0:02:40
0:02:40