filmov
tv
The Weak Derivative

Показать описание
Have you ever wondered how to differentiate a function that is not differentiable? In this video, I will show you how! It all relies on a simple integration by parts and a tiny bit of linear algebra. I will start by calculating the derivative of x^2, then I will calculate the derivative of |x|, and, more surprisingly, the second derivative of |x|. Be prepared to be amazed! :D
The Weak Derivative
Lecture04 Weak differentiability and weak derivative
I finally understood the Weak Formulation for Finite Element Analysis
Lecture 14 (part 1): Weak derivatives in L2(a,b), motivation and example
Generalized functions and weak derivatives - A very brief introduction
Mathematics: Extension of weak derivatives in Bochner spaces
Weak Solutions of a PDE and Why They Matter
Lecture05 Sobolev spaces
Class 10 th Maths- For weak Students| Final Revision| Real Numbers , Polynomial, linear equations
Differentiability of Curves in Banach Spaces
W8L2 Weak Solutions 2
W8L3 Weak Solutions 3
Sobolev Inequality (Embedding) via Riesz Potential
Math539 Lect21 part1
Lec 12 Properties and Examples
Muckenhoupt Ap Weighted Sobolev Functions
FEM: Session 5: Notion of strong vs. weak forms
More estimates for a Cauchy type operator and weak Wirtinger derivatives.
Theory of partial differential equations: discussion on syllabus and textbooks needed
0: Introduction and preliminaries
A weak quantitative Liouville theorem and introduction to Sobolev differential inclusions.
W8L4 Weak Solutions 4
Lecture 14 (Part 2): Integration operator and its realtion with weak derivatives
W8L1 Weak Solutions 1
Комментарии
 0:31:46
0:31:46
 0:30:08
0:30:08
 0:30:27
0:30:27
 0:26:18
0:26:18
 0:01:40
0:01:40
 0:10:02
0:10:02
 0:20:30
0:20:30
 0:49:08
0:49:08
 0:51:16
0:51:16
 0:36:49
0:36:49
 0:35:57
0:35:57
 0:09:18
0:09:18
 0:13:11
0:13:11
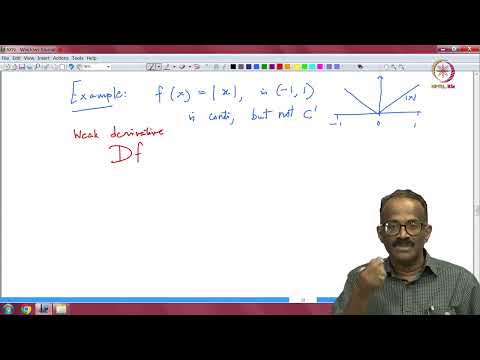 0:35:07
0:35:07
 0:25:57
0:25:57
 0:10:39
0:10:39
 0:49:25
0:49:25
 0:09:27
0:09:27
 1:22:07
1:22:07
 0:54:18
0:54:18
 0:36:28
0:36:28
 0:27:37
0:27:37
 0:30:35
0:30:35