Worked example: Approximation with local linearity | AP Calculus AB | Khan Academy

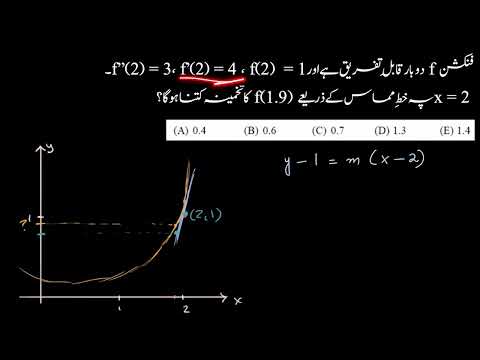
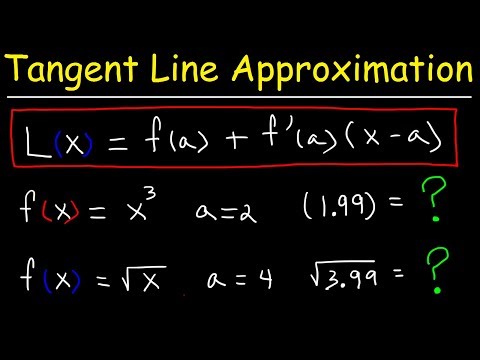
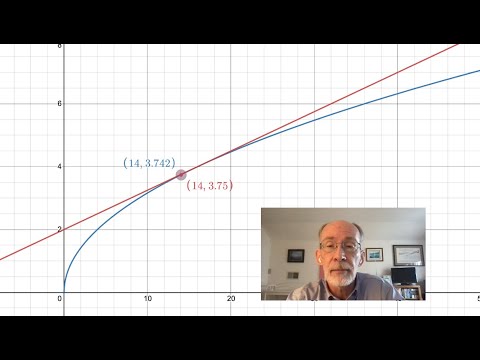
Finding the equation of a tangent line at a point of a curve by knowing the derivative at that point. Then using that equation to approximate the value of the function at close by x-values.

AP Calculus AB on Khan Academy: Bill Scott uses Khan Academy to teach AP¨_ Calculus at Phillips Academy in Andover, Massachusetts, and heÕs part of the teaching team that helped develop Khan AcademyÕs AP¨_ lessons. Phillips Academy was one of the first schools to teach AP¨_ nearly 60 years ago.

Khan Academy is a nonprofit organization with the mission of providing a free, world-class education for anyone, anywhere. We offer quizzes, questions, instructional videos, and articles on a range of academic subjects, including math, biology, chemistry, physics, history, economics, finance, grammar, preschool learning, and more. We provide teachers with tools and data so they can help their students develop the skills, habits, and mindsets for success in school and beyond. Khan Academy has been translated into dozens of languages, and 15 million people around the globe learn on Khan Academy every month. As a 501(c)(3) nonprofit organization, we would love your help! Donate or volunteer today!

[axelmoreno]

I just want to say a short but sweet thank you to you and the people of khan academy. I'm mostly planning to major in mathematics and use math in any field In the work place, but also learn how to effectively teach it too. Your app has helped me so much, not only that, but I always take notes too when I watch these videos and learn from them so I can help teach others. In general, I love learning and want to that you guys for helping me learn as I get closer to college

[aldolunabueno]

0, 615 is a better approximation using second derivative with Taylor polynomial, but for the exercise is fine. However, you should have indicated.

[enrianwicaksana]

Hello ! I'm a student from Indonesia. I think the right answer is 0.7
I find that f''(1, 9)≈3.7 and we know that f''(2)=3
So we can conclude that f'(x) is increasing from 1.9<x<2
From the approximation with the same way as you did we get that f(1.9)≈0.6
Since we know that the magnitude of the tangent line is increasing so point (1.9, 0.6) is below the f(1.9)
So f(1.9) is slightly greater than 0.6 and from the answer is 0.7

That's just my idea. Please review it !!!

[CossZt]

sin(x) = tan(x) = x

*Waits to be exiled*

[samlawler]

At 2:26, how do you know the slope of the tangent line is 4?

[andylaw]

Something coming up from my mind with a T at the