filmov
tv
03 Rayleigh-Schroedinger Perturbation theory Part 3

Показать описание
Degenerate perturbation theory
03 Rayleigh-Schroedinger Perturbation theory Part 3
2 Rayleigh-Schroedinger perturbation theory Part 2
CompChem: Correlation: 26. Rayleigh Schrödinger Perturbation Theory: 3. nth-Order Wavefunction
04 Rayleigh-Schroedinger Perturbation theory Part 4
Perturbation Theory in Quantum Mechanics - Cheat Sheet
1 Rayleigh-Schroedinger perturbation theory Part 1
Lecture14 Nondegenerate Rayleigh-Schroedinger Perturbation Theory
CompChem: Correlation: 24. Rayleigh Schrödinger Perturbation Theory: 1 Ansatz
CompChem: Correlation: 25. Rayleigh Schrödinger Perturbation Theory: 2. Energies
Many-body perturbation theory, October 28, 2022. Rayleigh-Schrödinger and Brillouin-Wigner
Oct23 Physics 151 time-dependent perturbation theory, Reyleigh scattering
PH3203 - Lecture 3
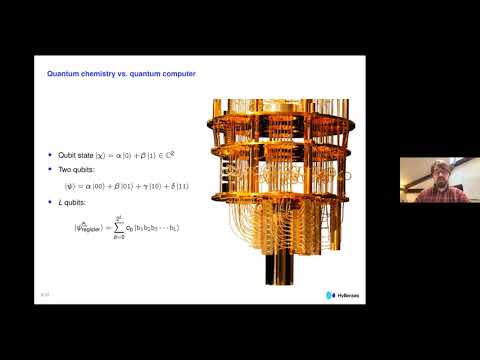
Multireference quantum chemistry on NISQ devices
OHM2013: Introduction to Quantum Perturbation
PERTURBATION THEORY FOR QUANTUM MECHANICS (INTRODUCTION)
Mod-04 Lec-28 Reyleigh-Schrodinger perturbation methods and adiabatic switching
PH3203 - Lecture 7
Perturbation methods Video 9 of 10.
Many-body lectures, perturbation theory, part 2
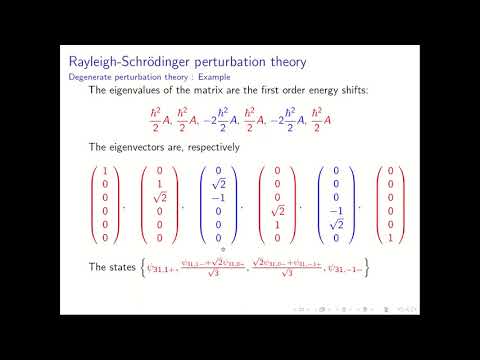
Degenarate Perturbation Theory : Part 2
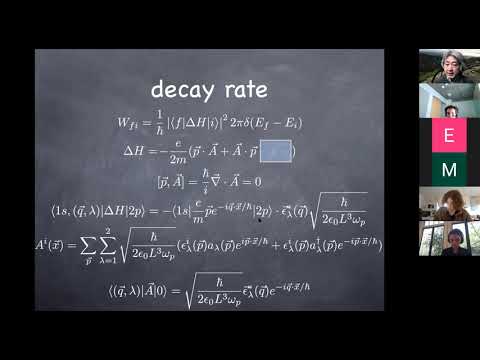
Mar17 emission of photon, selection rules, time-dependent perturbation theory
PH3203 - Lecture 5a
Episode-2 for perturbation theory (Time independent &Non-degenerate)
05 Dalgarno-Stewart Perturbation theory
Комментарии
 0:33:36
0:33:36
 0:42:36
0:42:36
 0:17:25
0:17:25
 0:41:36
0:41:36
 0:07:15
0:07:15
 0:42:03
0:42:03
 0:24:14
0:24:14
 0:11:59
0:11:59
 0:01:34
0:01:34
 1:18:40
1:18:40
 1:19:15
1:19:15
 0:53:50
0:53:50
 0:50:37
0:50:37
 0:23:48
0:23:48
 0:14:05
0:14:05
 0:53:30
0:53:30
 0:41:29
0:41:29
 0:46:12
0:46:12
 1:29:35
1:29:35
 0:38:58
0:38:58
 1:23:03
1:23:03
 0:33:34
0:33:34
 0:17:39
0:17:39
 0:35:26
0:35:26