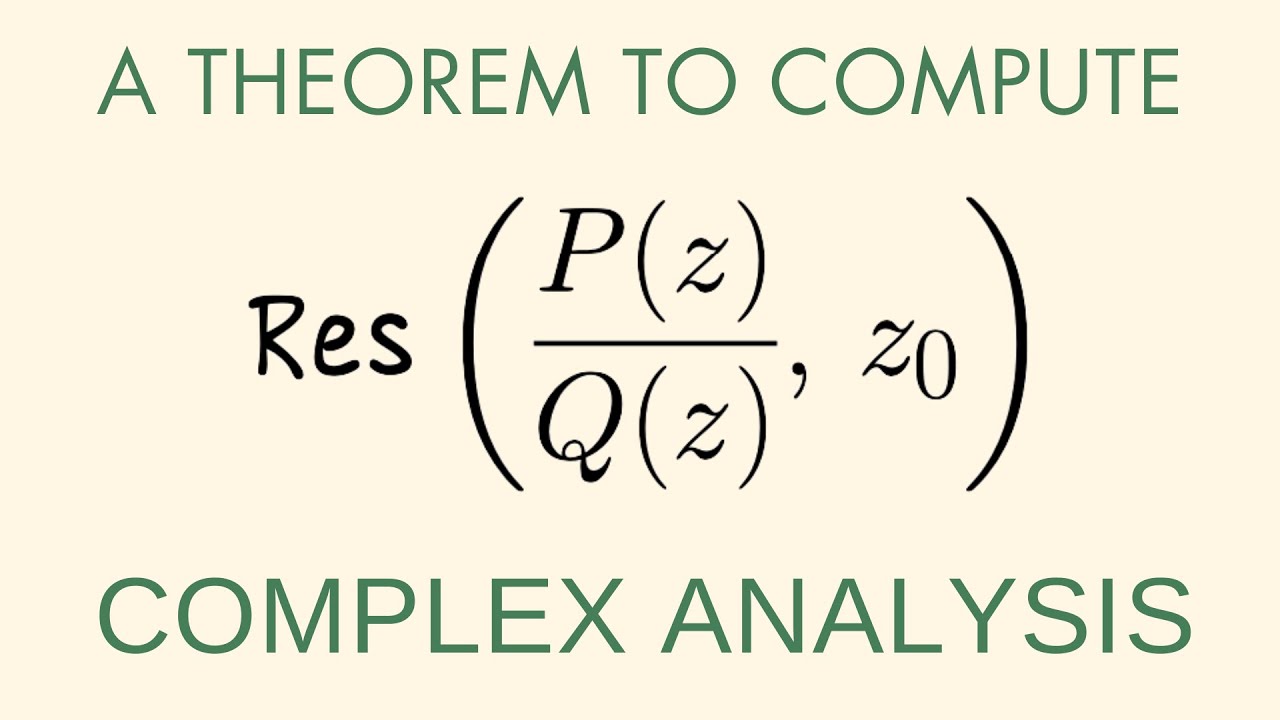
Calculating residues at simple poles, Complex Analysis

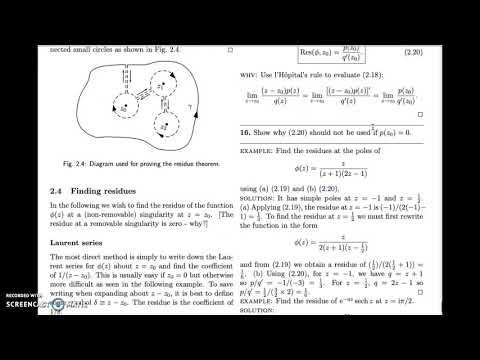
I show and prove a quick result from complex analysis that gives a nice way to compute the residue of a rational function with a simple pole. I start by stating the problem: given two polynomials, P(z) and Q(z) (with no common zero) with Q(z) having a simple zero at z0, we want to find the residue of the function f(z) = P(z)/Q(z) at z0. This scenario is typical when performing contour integrals involving simple singularities inside the contour (for, say, Cauchy's residue formula).

The proof is straightforward and requires us to manipulate the expression P(z)/Q(z) to bring in the difference quotient for Q at z0.

Then we do two quick computational examples.

#complexanalysis #mathematics #Contourintegration #integration #CauchysResidueTheorem #residuecalculus #mathtutorial ##ResidueComputation