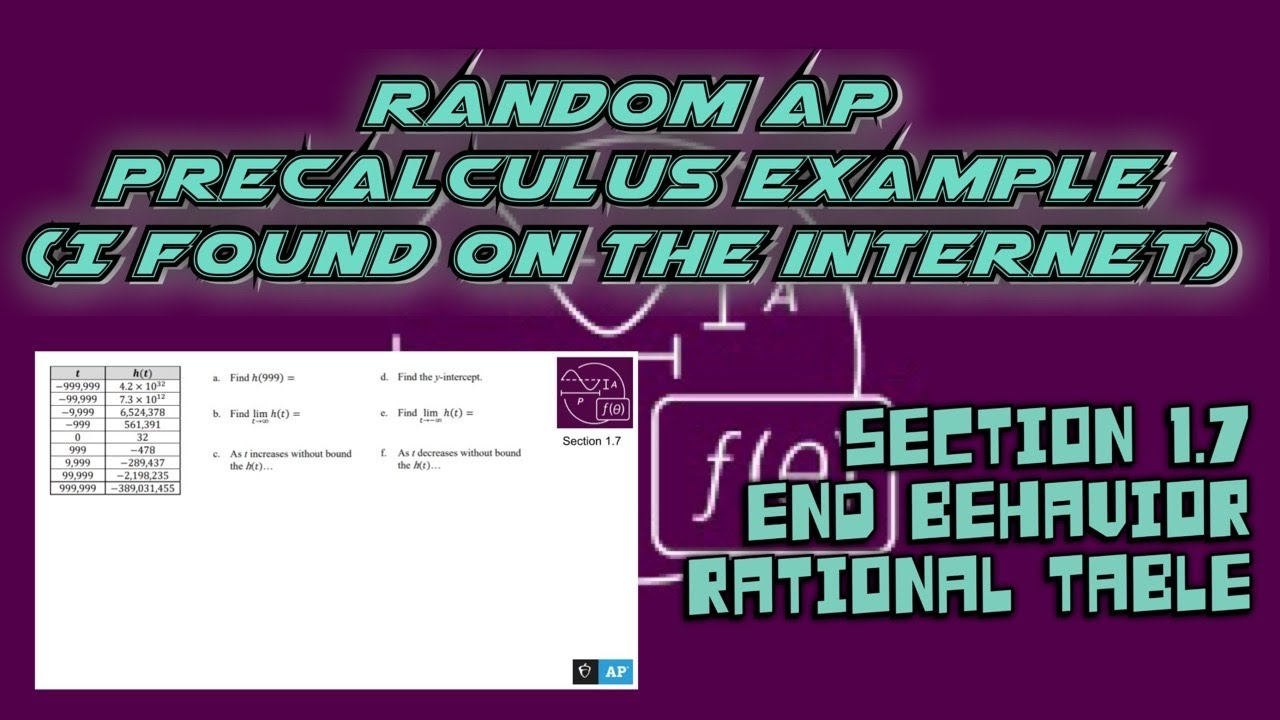
AP Precalculus Section 1.7 Example: End Behavior of a Rational Function from a Table

Random AP Precalculus Problems (I found on the Internet). These are not official AP Collegeboard examples, but they will definitely get the job done!

A table of values for a function can provide insights into its behavior, including predicting the end behavior. While tables don't give a comprehensive view like graphs do, they can still offer clues about how the function behaves as \(x\) approaches positive or negative infinity.

### Steps to Predict End Behavior from a Table of Values:

1. **Examine \(x\) values:** Look at the \(x\) values provided in the table. If the table includes values of \(x\) approaching positive and negative infinity (e.g., very large positive and negative values), focus on those.

2. **Analyze corresponding \(y\) values:** Corresponding \(y\) values for the extreme \(x\) values will reveal the trend in the function's behavior.

- If the \(y\) values for very large positive \(x\) values approach a certain number, it suggests a horizontal asymptote at that value. For instance, if \(y\) values for large positive \(x\) tend towards 4, it indicates a horizontal asymptote at \(y = 4\).

- If the \(y\) values for very large positive \(x\) grow without bound (increasing or decreasing without limit), it suggests that the function has no horizontal asymptote and its end behavior leads to positive or negative infinity.

3. **Look for patterns:** Consider the trend of the \(y\) values. Are they getting closer to a certain value as \(x\) increases or decreases without bound? Is there a consistent pattern in the values that indicates convergence towards a particular number or divergence towards infinity?

### Example:

Let's say we have a function and its corresponding table of values:

| \(x\) | \(y\) |
|------|------|
| 10 | 2 |
| 100 | 1.8 |
| 1000 | 1.7 |
| -10 | -2 |
| -100 | -1.8 |
| -1000| -1.7 |

From the table:

- As \(x\) approaches positive infinity, the \(y\) values decrease but seem to approach 1.7. This suggests a horizontal asymptote at \(y = 1.7\) for \(x\) approaching positive infinity.
- As \(x\) approaches negative infinity, the \(y\) values also decrease but tend toward -1.7. This suggests a horizontal asymptote at \(y = -1.7\) for \(x\) approaching negative infinity.

By analyzing the trend in the table, you can predict the behavior of the function as \(x\) moves towards positive or negative infinity.

The Topics covered in AP Precalculus are...

1.1 Change in Tandem
1.2 Rates of Change
1.3 Rates of Change in Linear and Quadratic Functions
1.4 Polynomial Functions and Rates of Change
1.5 Polynomial Functions and Complex Zeros
1.6 Polynomial Functions and End Behavior
1.7 Rational Functions and End Behavior
1.8 Rational Functions and Zeros
1.9 Rational Functions and Vertical Asymptotes
1.10 Rational Functions and Holes
1.11 Equivalent Representations of Polynomial and Rational Expressions
1.12 Transformations of Functions
1.13 Function Model Selection and Assumption Articulation
1.14 Function Model Construction and Application
2.1 Change in Arithmetic and Geometric Sequences
2.2 Change in Linear and Exponential Functions
2.3 Exponential Functions
2.4 Exponential Function Manipulation
2.5 Exponential Function Context and Data Modeling
2.6 Competing Function Model Validation
2.7 Composition of Functions
2.8 Inverse Functions
2.9 Logarithmic Expressions
2.10 Inverses of Exponential Functions
2.11 Logarithmic Functions
2.12 Logarithmic Function Manipulation
2.13 Exponential and Logarithmic Equations and Inequalities
2.14 Logarithmic Function Context and Data Modeling
2.15 Semi-log Plots
3.1 Periodic Phenomena
3.2 Sine, Cosine, and Tangent
3.3 Sine and Cosine Function Values
3.4 Sine and Cosine Function Graphs
3.5 Sinusoidal Functions
3.6 Sinusoidal Function Transformations
3.7 Sinusoidal Function Context and Data Modeling
3.8 The Tangent Function
3.9 Inverse Trigonometric Functions
3.10 Trigonometric Equations and Inequalities
3.11 The Secant, Cosecant, and Cotangent Functions
3.12 Equivalent Representations of Trigonometric Functions
3.13 Trigonometry and Polar Coordinates
3.14 Polar Function Graphs
3.15 Rates of Change in Polar Functions

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#APPrecalculus
#PreCalcProblems
#MathMinds
#AdvancedPreCalc
#TrigTales
#PrecalcPuzzles
#FunctionFiesta
#GraphGoals
#CalcReady
#PreCalcLife
#AlgebraicAdventures
#DerivativeDreams
#IntegrationInsights
#MathematicsMagic
#PreCalcReview
#PrecalcConcepts
#LogarithmLove
#TrigonometryTips
#MathMastermind
#APCalcPrep
#Mathematics
#MathMinds
#Math
#Maths