filmov
tv
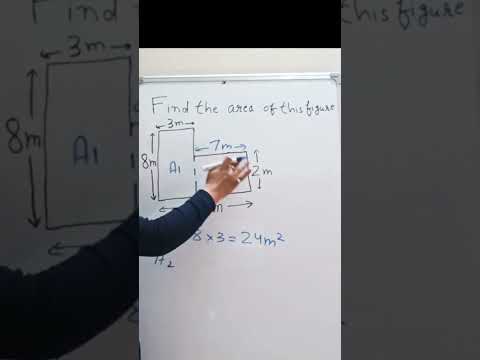
Calculate the Area of the Red Rectangle

Показать описание
In today's video, we'll tackle an intriguing geometry problem: calculating the area of the red rectangle. This problem might seem simple at first, but it requires a good understanding of geometric principles.
🔍 In this video, you will learn:
- How to identify key elements of the given shape.
- Step-by-step instructions to calculate the area of the red rectangle.
- Useful tips and tricks to solve similar geometry problems.
📐 Topics Covered:
- Basic geometric shapes and properties
- Area calculation techniques
- Problem-solving strategies in geometry
Don't forget to:
- Like the video if you found it helpful 👍.
- Subscribe to our channel for more math tutorials and problem-solving tips.
- Hit the bell icon 🔔 to get notified whenever we post new content.
Have questions or suggestions? Drop them in the comments below. I love hearing from you!
-------------------------------------------------------------------
📧 Contact Me:
------------------------------------------------------------------
Happy learning and see you in the video!
🔍 In this video, you will learn:
- How to identify key elements of the given shape.
- Step-by-step instructions to calculate the area of the red rectangle.
- Useful tips and tricks to solve similar geometry problems.
📐 Topics Covered:
- Basic geometric shapes and properties
- Area calculation techniques
- Problem-solving strategies in geometry
Don't forget to:
- Like the video if you found it helpful 👍.
- Subscribe to our channel for more math tutorials and problem-solving tips.
- Hit the bell icon 🔔 to get notified whenever we post new content.
Have questions or suggestions? Drop them in the comments below. I love hearing from you!
-------------------------------------------------------------------
📧 Contact Me:
------------------------------------------------------------------
Happy learning and see you in the video!
Area of a triangle #area #maths #mathsrevision
✨ AREA Calculation in AutoCAD #autocadtutorial #autocadtips #area #autocadtipsandtricks
Can you calculate area of the Yellow Square? | (Triangle) | #math #maths | #geometry
How to Find the Area of a Triangle | Calculate the Area of a Triangle
How to Calculate Area of Irregular Land or Plot Step by Step
Area of square | How to find area of the square #shorts
AutoCAD 2D - Calculate areas (2 methods - easy and fast way)
Finding the Perimeter
Can you afford a dream house? #downpayment #creditscore #homebuyers #homebuyingtips #homebuyerguide
Geography Mapwork: How to calculate Area on a map
Area of the figure | Area of the rectangle | #mathstricks #shorts
How to Calculate Area | Learn how to calculate area and apply it in the real world
How to Calculate the Area of an Ellipse
How To Calculate The Area of a Semicircle
Floor Tiles Calculation for a room #short #shorts 📝🏗️🏨
What’s the area?
calculate area for shapes|| autocad shorts
Area and perimeter of square | Ayushi teacher | Area and perimeter #maths #area #perimeter
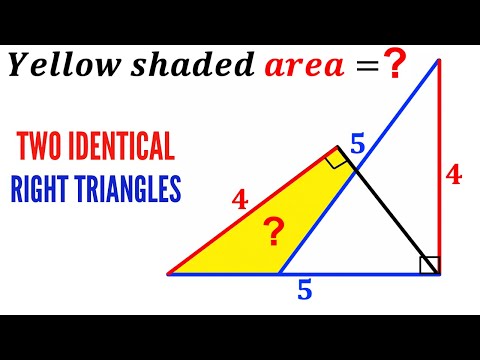
Can you calculate area of the Yellow shaded region? | (Triangles) | #math #maths | #geometry
Area of a Circle | Beat the Calculator #shorts
4 methods to calculate area
How to automatically calculate area from floor plans. #shorts #architect
how to calculate the plot area how to convert square feet of a plot into square yards#shorts
Area of a Rectangle | How to Calculate Area of a Rectangle | Math Help with Mr. J
Комментарии
 0:00:33
0:00:33
 0:00:32
0:00:32
 0:10:58
0:10:58
 0:06:37
0:06:37
 0:07:20
0:07:20
 0:00:30
0:00:30
 0:01:53
0:01:53
 0:00:40
0:00:40
 0:00:33
0:00:33
 0:07:40
0:07:40
 0:00:48
0:00:48
 0:12:59
0:12:59
 0:00:28
0:00:28
 0:05:07
0:05:07
 0:00:16
0:00:16
 0:00:42
0:00:42
 0:00:26
0:00:26
 0:00:16
0:00:16
 0:06:39
0:06:39
 0:00:52
0:00:52
 0:01:02
0:01:02
 0:00:23
0:00:23
 0:00:16
0:00:16
 0:05:28
0:05:28