filmov
tv
An ingenious & unexpected proof of the Binomial Theorem (2 of 2: Proof)

Показать описание
An ingenious & unexpected proof of the Binomial Theorem (1 of 2: Prologue)
An ingenious & unexpected proof of the Binomial Theorem (2 of 2: Proof)
The Man Who Took LSD and Changed The World
Do you know Leonardo da Vinci's circle area proof?
Iwan Baan: Ingenious homes in unexpected places
The Ingenious Gas-Resistant Pram That Could Save Lives During War
Have You Ever Been Provoked? Churchill’s Ingenious Response to Lady Astor #history #amazinghistory...
One of his name's was, he who makes ingenious things out of the combat! - Jordan Peterson #shor...
Ingenious construction workers building brick tunnel in rural
Ingenious Camping Inventions That Are On The Next Level | Best Of All Time
Aliens Stumble Upon Ancient Human Fleet Remains Amazed by Ingenious Engineering |Galactic HFY Sci-Fi
Horrible Histories Ingenious Inventors 🔭 Science Milestones Through the Ages | CBBC
Cruise Ships – Ingenious Engineering – Big Bigger Biggest
10 INGENIOUS INVENTIONS YOU SHOULD SEE
D-Day’s Forgotten Critical Secret Weapon
Secrets Hidden Inside Mountains - Part 1
Cauchy's Proof of the Basel Problem | Pi Squared Over Six (3blue1brown SoME1 Entry)
The Strangest Mathematician in History
Escape from Alcatraz: Ingenious or Impossible? #shorts
12 INGENIOUS INVENTIONS YOU'VE NEVER SEEN BEFORE
Building the Worst WW2 Air Force - Terrible Aircraft and How to Sell Them (feat. @AnimarchyHistory )
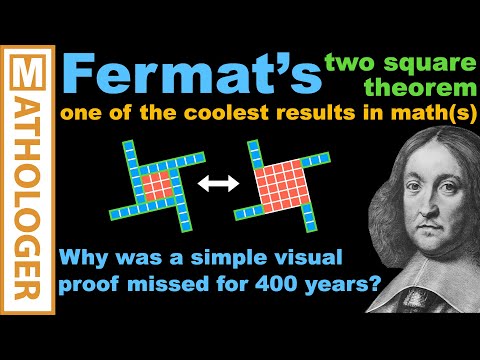
Why was this visual proof missed for 400 years? (Fermat's two square theorem)
The Sharks Are Amazed When Ketchup Rolls Right Off Of Baobab's White Shirts | Shark Tank Global
45 Ingenious Tools That Are At Another Level on Amazon 2025
Комментарии
 0:06:55
0:06:55
 0:16:16
0:16:16
 0:33:40
0:33:40
 0:00:54
0:00:54
 0:16:59
0:16:59
 0:00:45
0:00:45
 0:00:36
0:00:36
 0:00:57
0:00:57
 0:00:12
0:00:12
 1:10:40
1:10:40
 0:14:50
0:14:50
 0:14:26
0:14:26
 0:45:35
0:45:35
 0:12:09
0:12:09
 0:35:43
0:35:43
 0:26:21
0:26:21
 0:09:59
0:09:59
 0:10:48
0:10:48
 0:01:00
0:01:00
 0:11:19
0:11:19
 1:06:15
1:06:15
 0:33:59
0:33:59
 0:09:34
0:09:34
 0:35:18
0:35:18