filmov
tv
Response of an LTI System: Convolution
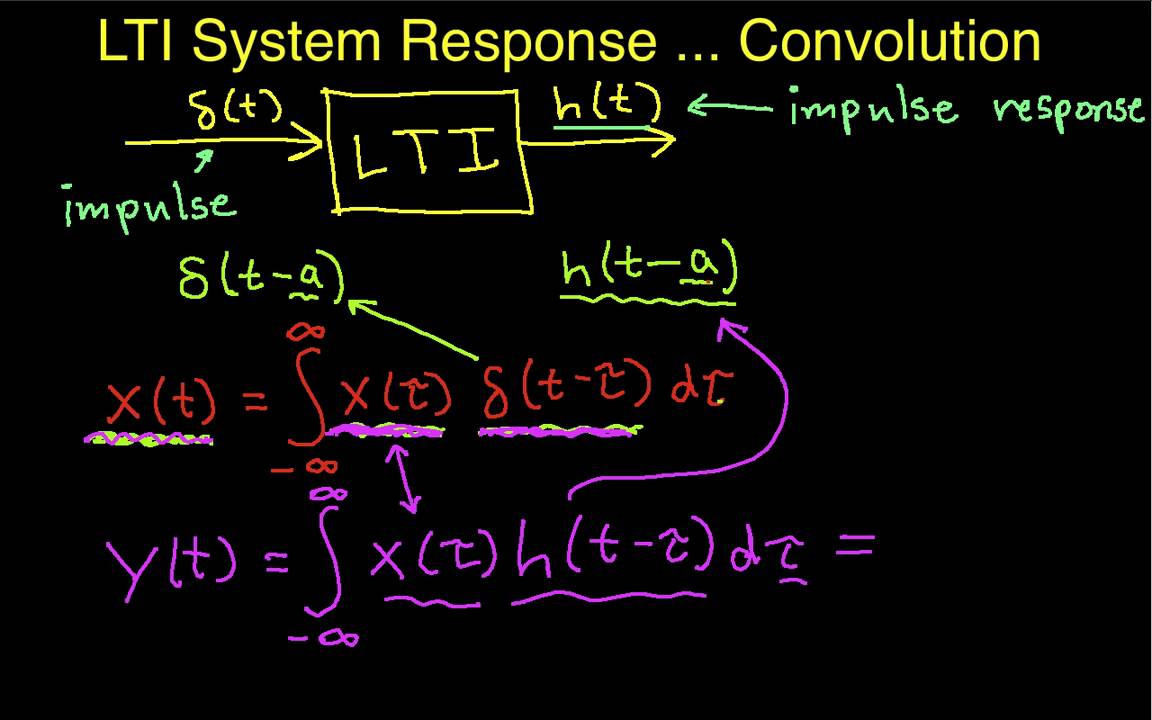
Показать описание
Shows how the response of an LTI system to an arbitrary input is obtained as the convolution of the impulse response of the system with the input.
Impulse Response and Convolution
What is a Linear Time Invariant (LTI) System?
Linear Time-Invariant (LTI) Systems
step response of LTI system in signals and systems || EC Academy
Convolution and Unit Impulse Response
The impulse response of a linear time invariant system is h[𝒏]. find the response of the system.
Linear Time-Invariant(LTI) system- concept, convolution, properties, deconvolution, identity system
Impulse Response of an LTI System #math #maths #engineering #electricalengineering
Time domain - tutorial 8: LTI systems, impulse response & convolution
Example: Output by convolution of input with impulse response
Response of an LTI System: Convolution
Impulse Response & Transfer Function of a System
Review of Linear Time-Invariant (LTI) Systems
Chapter 02 Part 1: Impulse Response and Convolution for Discrete Time Systems
Frequency Response Descriptions for LTI Systems
#113 Properties of LTI System in terms of impulse response and SLOVED PROBLEM // EC Academy
Stable LTI System
DSP Topic 4: Impulse Response of LTI Systems (V8)
Causal LTI System
M3L2 - Defining and Deriving Step Response of a LTI system
Response of an lti system : convolution
IMPULSE RESPONSE & TRANSFER FUNCTION of LTI system
Step Response of a System
Step response of an LTI system.
Комментарии
 0:09:49
0:09:49
 0:06:17
0:06:17
 0:06:37
0:06:37
 0:04:30
0:04:30
 0:09:22
0:09:22
 0:16:14
0:16:14
 0:06:58
0:06:58
 0:00:37
0:00:37
 0:13:19
0:13:19
 0:04:53
0:04:53
 0:11:59
0:11:59
 0:05:06
0:05:06
 0:10:41
0:10:41
 0:29:04
0:29:04
 0:15:17
0:15:17
 0:06:42
0:06:42
 0:05:29
0:05:29
 0:18:27
0:18:27
 0:08:00
0:08:00
 0:12:23
0:12:23
 0:11:58
0:11:58
 0:12:51
0:12:51
 0:11:29
0:11:29
 0:08:23
0:08:23