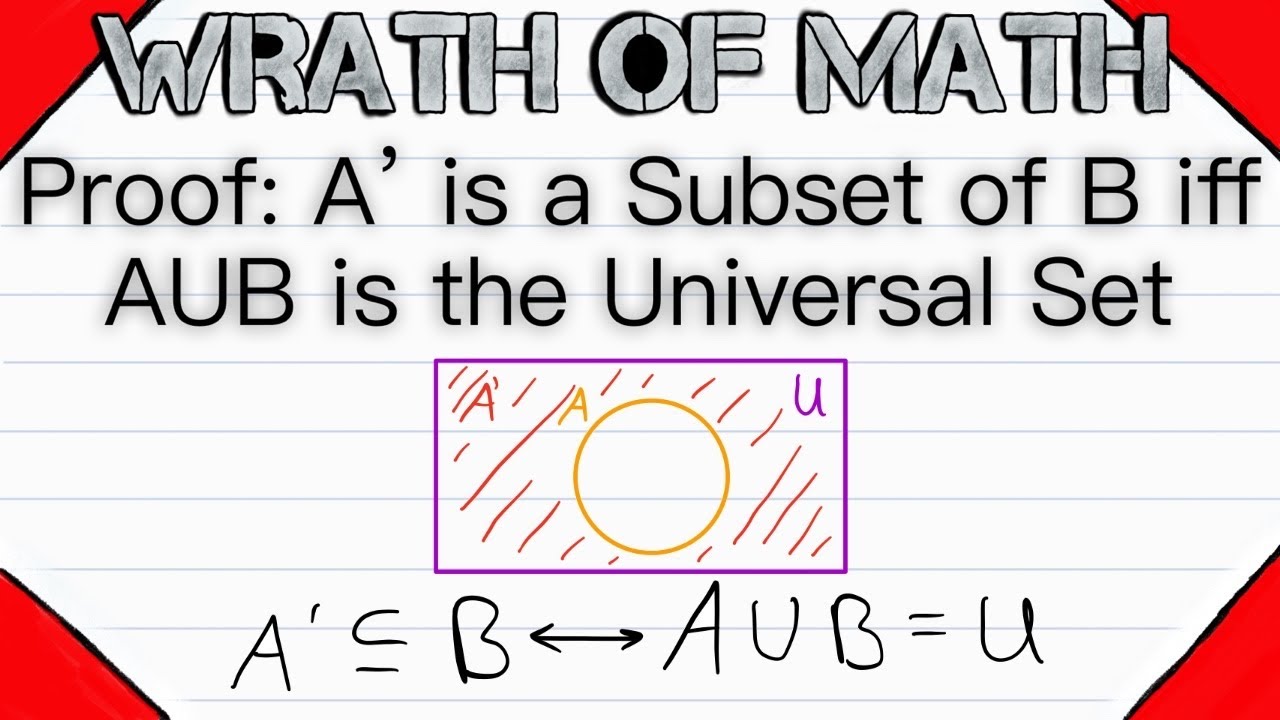
Proof: A' is a Subset of B iff AUB is Universal Set | Set Theory

Показать описание

Let A and B be subsets of a universal set U. Then the complement of A, written A', is a subset of B if and only if A union B is the universal set! We'll go over this set theory proof in today's set theory lesson. All we need to do is use our definitions of set complement, set equality, subset, and set union, and we'll be able to prove this biconditional set theory result.

I hope you find this video helpful, and be sure to ask any questions down in the comments!

********************************************************************
The outro music is by a favorite musician of mine named Vallow, who, upon my request, kindly gave me permission to use his music in my outros. I usually put my own music in the outros, but I love Vallow's music, and wanted to share it with those of you watching. Please check out all of his wonderful work.

********************************************************************

+WRATH OF MATH+

Follow Wrath of Math on...

Рекомендации по теме

Комментарии

You are always great with your lectures, they are super useful

WhenCatPlayz

Can you pls do it in Identity base not in definition

Akitchen

bro you're saving my life, thank you

anonymousvevo

1/ A is a subset of the universal set S means, by definition of the universal set, A U A' = S. As S = A U B, we should have A U A' = A U B. By definition of set union, this means A' = B, and therefore A' is a subset of B.
2/ On one hand, A and B be are subsets of a universal set S. This implies, by definition of set subset, that A U B is a subset of S.
On the other, if s is an element of the universal set S then, by definition of the universal set, S = A U A' and s is of course an element of A or A'. As A' is a subset of B then s is an element of A or B, that is s is an element of A U B and the universal set S is a subset of A U B. So A U B and S are subsets of each other, which is a way to say A U B = S.

azizhani

Proof: A' is a Subset of B iff AUB is Universal Set | Set Theory

Prove A is a subset of B with the ELEMENT METHOD

Set Theory Proof A is a subset of B if and only if A intersect B = A

Proof: A is a Subset of B iff A intersect B Equals A | Set Theory, Subsets

Proof: A is a Subset of B iff B' is Subset of A' | Set Theory, Subsets, Set Complement

Proof: If A is a Subset of B then P(A) is a Subset of P(B) | Power Sets, Set Theory

Proving subsets

Set Theory Subset Proof

How to do a PROOF in SET THEORY - Discrete Mathematics

Prove that set A is subset of set B. A intersects B = A. Abstract Algebra. Set Theory

The Empty Set is a Subset of Every Set Proof

Proving A is a subset of B

Proof: If P(A) is a Subset of P(B) then A is a Subset of B | Set Theory

Proof: Subset is a Transitive Relation | Set Theory, Subsets, Transitivity

Proof: A is a Subset of B iff A Union B Equals B | Set Theory, Subsets

How To Prove A Set Is A Subset Of Another Set

Proof: A-B is a Subset of A union B | Set Theory

An empty set is a subset of every set (Proof) [ILIEKMATHPHYSICS]

Why is the Empty Set a Subset of Every Set? | Set Theory, Subsets, Subset Definition

Proof: A is a Subset of B' iff B is a subset of A' | Set Theory, Set Complements

Set Theory Proof: A x C is a Subset of B x C if and only if A is a Subset of B

Prove that A is a subset of A union B for any sets A and B.

A Set Is A Subset Of Itself

An Intro to Proof Based Math: Proving A is a Subset of B

Can You Prove a subset relation with symmetric difference?