Climb to Infinity!

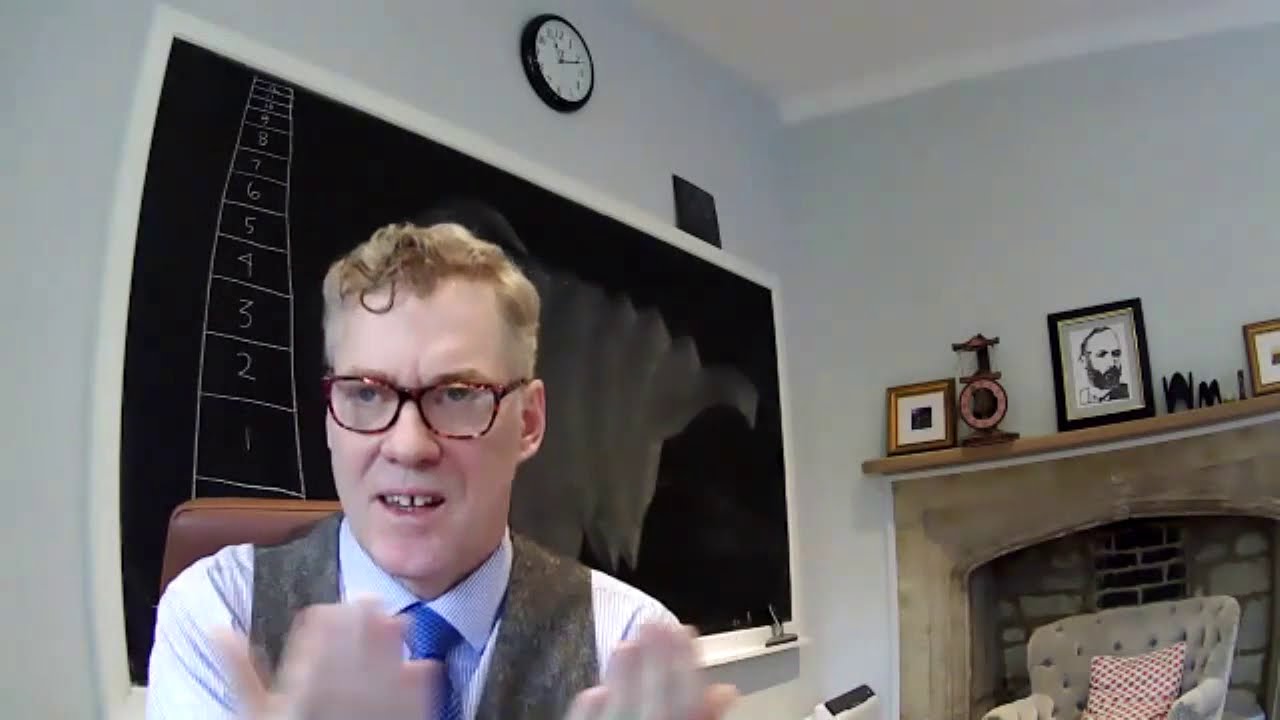
Joel David Hamkins, Professor of Logic, Oxford University

Lecture 3. Infinity

We shall follow the allegory of Hilbert’s hotel and the paradox of Galileo to the equinumerosity relation and the notion of countability. Cantor’s diagonal arguments, meanwhile, reveal uncountability and a vast hierarchy of different orders of infinity; some arguments give rise to the distinction between constructive and nonconstructive proof. Zeno’s paradox highlights classical ideas on potential versus actual infinity. Furthermore, we shall count into the transfinite ordinals.

[minch]

This has been great so far! Can't wait for next week, thanks for putting these up to the public

[Achrononmaster]

@59:40 yeah, but you need to be very careful about educating economists. They can be a bit nerdy and autistic in a dangerous way. In economics the Pareto principle is often grossly violated. It goes under various fallacies of composition. Such as the paradox of thrift: everyone is at least slightly better off if they net save. But if everyone net saves then everyone starves and dies (in a monetary economy). In a monetary economy with no counterfeit (so strict accounting laws) some agency has to net dissave. That is typically government, and government can _always_ net spend, since they are the monopoly currency issuer. It is called "running a deficit".
Most Europeans think this is a shockingly appalling bad thing to not have people begging for the state currency (hence they introduce insane "fiscal rules" in the EMU which cause needless austerity and mass unemployment), but they've got basic accounting 101 all wrong, it is a *_good thing_* for government to run a deficit --- no ones "tax dollars" are paying for this --- although the government deficit need not be targeted, the supply can float in order to accommodate a stable price level at full employment --- by which I mean zero involuntary unemployment, not the NAIRU myth, the reality is a NAIBER not a nairu, or "non accelerating inflation buffer employment ratio". Employment and price level are all about buffer stocks, which mainstream macroeconomists never seem to consider when theorizing about inflation, being the idiots they are, they are pretty evil really. (Bet you didn't think a few words about reflexive and partial orderings would lead to life & death morality in the real world. But this comment is just basic math for state money accounting systems.)
I think it can also be viewed as a comment about directed acyclic graphs? So from DAG's you get an understanding about the source of the price level, which no mainstream economist understands (I am willing to bet).
Government deficits accommodate non-government savings desires (to the penny). (Inflation has nothing to do with this.) But try telling this to a Neoclassical or New Keynesian "professional" and they'll wet their pants.

[Achrononmaster]

@38:50 what I like is that you "construct" all algebraic numbers by "listing" all the polynomials over rationals --- surely countable, and they're bijective with the strictly finite sub-lists of rationals or integers that are the coefficients. But you never _actually_ construct this list. You only "know you could" if you were a god. This presumption of humble god-like powers is what finitists often object to, but the humility is beautiful. It is "well, if _only_ I had infinite paper and infinite time, or could do the proverbial ω many tasks in 2 seconds by working twice as fast each task step. While I am against tying mathematics to "physical tasks" (or even ω −Turing machine tasks) like this, since it leads to seeming pathologies like Banach-Tarski, it does cry out for a better verb than "construct". It is "mental construction". But what's the shorthand for that? It is "conceivability" or "computability" is it not? But _strong conceivability_ --- one must have a means of computation available _as if_ one were a humble god who merely has vast resources (infinite time or time steps).
Which brings me to the question: has anyone got some of these proofs (involving the reals that use such god-like constructive arguments) in the form of computations involving tasks that could be completed in finite time and finite time steps? (i.e., non god-like.) Not that I care, I am fine with the nonconstructive proofs, and infinite tasks. But I'd like to have the occasion now and then to throw old Norman Wildberger some scraps.

[EsatBargan]

Martin Maria Martin Melissa Lopez Timothy

[Achrononmaster]

@1:25:00 another answer is that historically people did not understand "=" does not mean "the same" or "identically equal". So _x_ + _x_ = 2 _x_ = _x_ 2, is fine for finite numbers "up to isomorphism". But Cantor, yeah, he's the GOAT.

[Achrononmaster]

@1:17:00 not having a clear concept of ℕ, and thinking Dedekind-Peano is "murky" is fine. It gives mathematicians things to do. It does not force one to adopt pluralism. Pluralism is fine too, as a framework. But it is fragile, right? If you could get a non-murky definition of ℕ, then that kills pluralism for ℕ (a damn good thing in my opinion). However, Gödelman always flies to the rescue, no? There's always some structure you can validly desire to be pluralist about, like today the CH. Or "The Continuum" in general --- hyperreal continuua, Surreal continuum (the Surreals are non-murky?), or Cantor-Rucker "The Absolute" continuum (cardinality of Ω = the absolute infinite.)
However, does not a lot of this boil down to words? With finer grain knowledge of mathematical structure we can name things that were once thought the same differently, provided we do not run out of words. Like 1960's particle physicists. 🤣 (that's a joke). I honestly think this game can go on forever, the question is whether mathematicians will last for _more than forever_ so the game terminates! 😜

[Achrononmaster]

@1:14:00 I am pretty sure, gut instinct, that what Ahmed meant was whether there is a problem with mathematical induction when we allow transfinite numbers, like ω +1, or ω·ω. Maybe? The answer is no (I think). The issue he might have had in mind was that if we can "go to" ω+1 then how come the induction over ℕ is valid? The answer is that it is valid because the proof using induction is induction only over ℕ. But the same idea extends to validity of transfinite induction.
For these things I find the category theoretic style better than the indexing style. The CT folks tend to write an "index set" and try to never use an index subscript. It is just conceptually clearer that then one is staying in ℕ (or whatever index set one is using). Although I think I am not expressing this well. I got it off Misha Gromov ("I hate subscripts." He is also known to have said, "I don't know what a matrix is, a matrix is stupid." --- which being a Clifford algebra user I fully agree with.... not just for the laughs! Although mostly for the laughs.).

[wasifsultani]

Great talk on Infinity! Thanks for sharing.

[rath]

binary expansions would have the unique representation problem

[erincarmody]

Thanks for a great talk! Maybe we can't know if Goldbach's conjecture is true or false since not all the evens have been created yet haha :)! Thanks for your comments on my comment and excellent talk on Infinity!

[namelastname]

A better microphone would go a long way

[martinepstein]

1:24:20 In his chapter on order type arithmetic Enderton says r+s as "r, then s" and r*s as "r, s times" which I find helpful.

[wholesomeinfj]

I am enjoying your videos! Thanks so much for sharing your knowledge with the world

One note, the f(p, q) = 3^p * 5^q argument needs more precision since that isn't technically a bijection to the natural numbers, as it isn't surjective (i.e. there are odd natural numbers that will not be mapped to by f)

[roberthvistendahl]

I would suppose there is a discrepancy between the plethora of uses for infinity in mathematics and physics/ and the practical world. If the hotel 'had infinite guests', they would still be BEING CHECKED IN!!

[bob.x]

Regarding the cruise ship .. How can there be enough people onboard to take all the real number tickets? Since people would be a countable infinity and so smaller than the number or real numbers. Having said that can there even be enough tickets to print all the real numbers on? Ha! enjoying this series !!

[jalepezo]

Thank u for shaing, I am a math undergraduate now working with infinite sets and measure theory, really a mess,

[Zineas]

Cantor's original argument seems to me suspiciously tooo similar to Aristotle's argument about magnitudes and how he refutes Zeno's paradox.

[benhbr]

36:35 Its’s Liouville, not Louisville :)

[glebpolevoy]

You say aleph one is the smallest cardinal larger than aleph zero and so on, regardless whether aleph 1 is equal to beta 1, i.e. regardless whether the cont. hypothesis holds. How do you know there exists the smallest such cardinal? Are the cardinals known to be well-ordered?

[АндрейДенькевич]

12:00 absolutely no difference how to count by rows or by diagonals.