filmov
tv
Finding lower and upper bounds for sin(x)/x on [pi/4,pi/2]
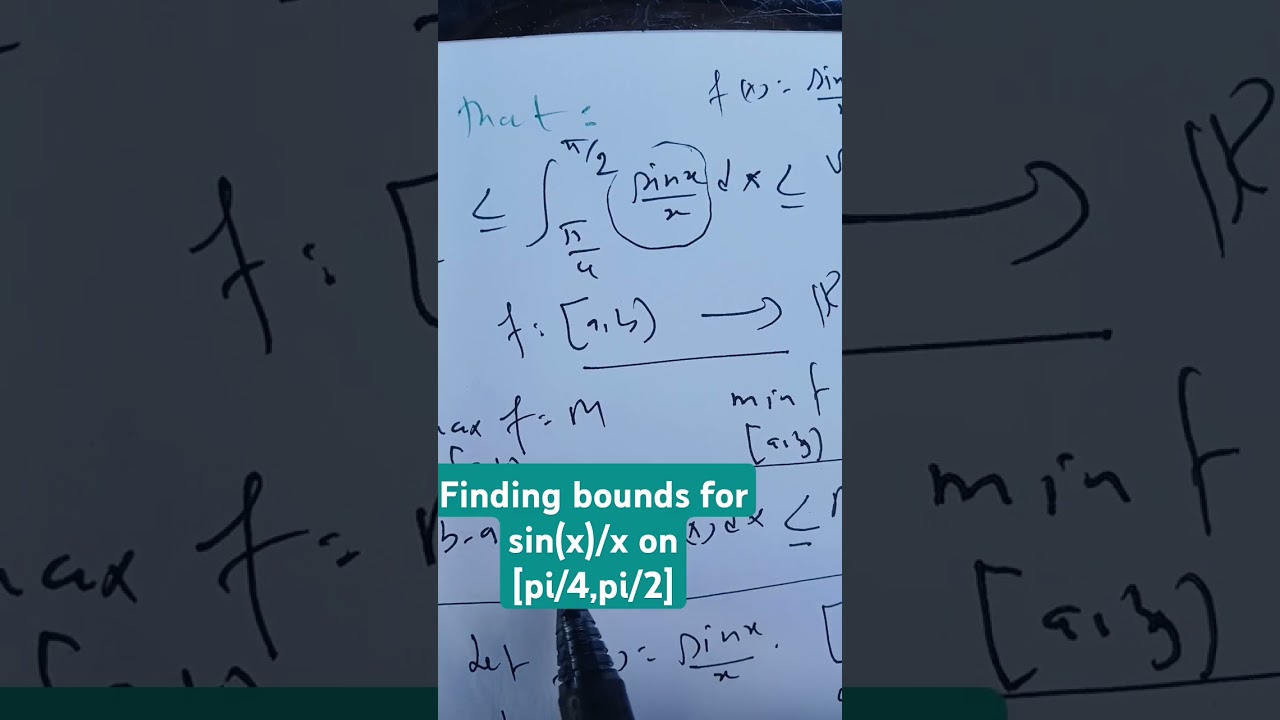
Показать описание
We're going to talk about the properties of integral in general. So assume in this case that we have a function that's continuous on some interval[a,b,].We Define the integral of a Off f, over [a,b] As the limit as n goes to Infinity of f of x, Delta X. So this is known as the Rieman sum. We can prove that the integral Is linear. That means The one we integrate Two functions that we add. We're gonna get the integral of the two functions that we have to add. If we multiply a function by a scalar and we integrate, we're going to get the scalars time, the integral of that function. If we have a function that's zero, It's integral is gonna be zero, We can't have a function that has integral zero but it can not be zero Or it can be easier. Not in general, not all the time. We also got a Define the mean value For integrals. We also can prove, the fundamental theorem of calculus for integral the first form. And the second form. We also going to use these theorems that you compute many results, concerning the properties of integral. We're gonna see that we can use the disk method and the cylindrical, or the shells method to compute the volume of some Objects, Our case. But anyways, we can show that the integral is linear. The integral Of an even function is twice, the integral between 0 and a of that function and the integral of an an odd. Function Is zero. If we integrate between - aand a .
khanacademy,zack star ,cybermath, michael penn,blackpenredpen, ap calculus, praxis math5161 , olympiad, putnam, india cometition math,china competition math, mindyourdecisions, how to be good in math , how to overcome math fear, math sorcerer, differential equation, cal1, calc2,cal3
learn hard problems, you can make it if you do a bit of work daily.
#python #calculus #olympiad
khanacademy,zack star ,cybermath, michael penn,blackpenredpen, ap calculus, praxis math5161 , olympiad, putnam, india cometition math,china competition math, mindyourdecisions, how to be good in math , how to overcome math fear, math sorcerer, differential equation, cal1, calc2,cal3
learn hard problems, you can make it if you do a bit of work daily.
#python #calculus #olympiad
 0:08:16
0:08:16
 0:03:14
0:03:14
 0:04:32
0:04:32
 0:18:35
0:18:35
 0:05:38
0:05:38
 0:02:06
0:02:06
 0:06:38
0:06:38
 0:15:39
0:15:39
 3:06:37
3:06:37
 0:10:34
0:10:34
 0:13:37
0:13:37
 0:00:16
0:00:16
 0:27:13
0:27:13
 0:15:25
0:15:25
 0:03:23
0:03:23
 0:02:30
0:02:30
 0:14:16
0:14:16
 0:03:10
0:03:10
 0:07:09
0:07:09
 0:06:08
0:06:08
 0:02:22
0:02:22
 0:15:55
0:15:55
 0:23:16
0:23:16
 0:05:21
0:05:21