How To Prove Bernoulli's Inequality

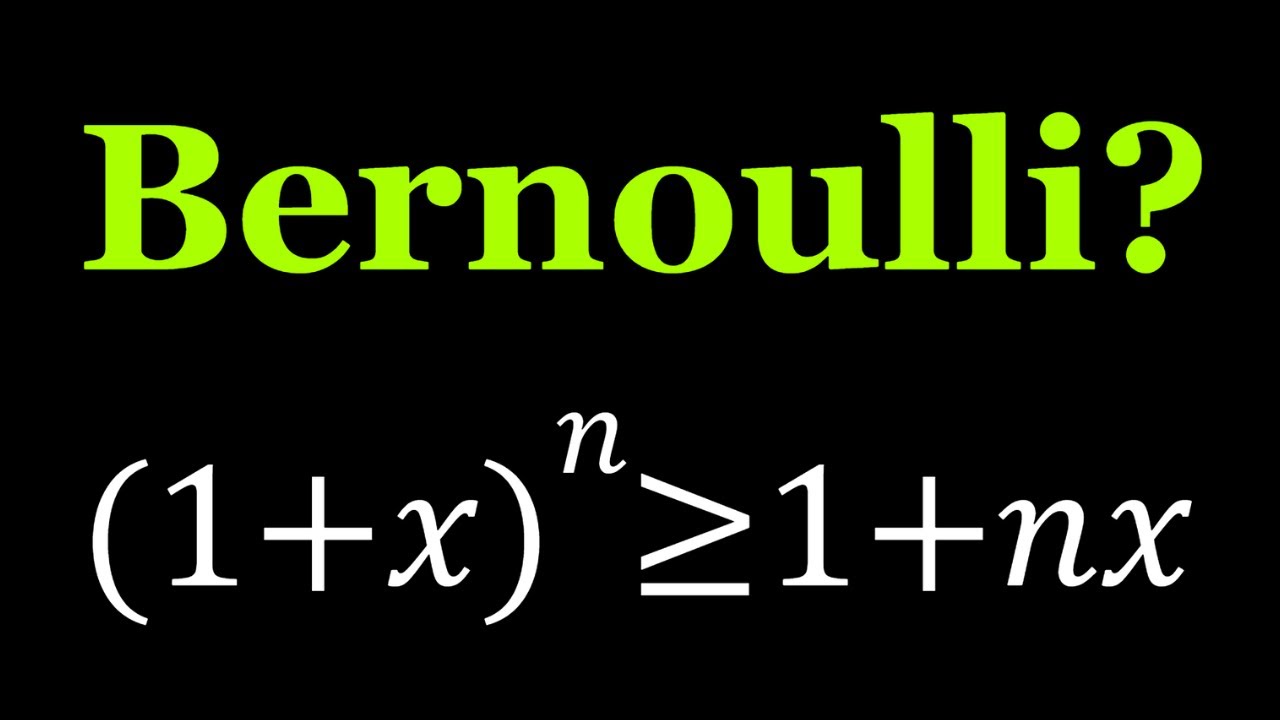
preview_player
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡

If you need to post a picture of your solution or idea:

#inequalities #algebra
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva

PLAYLISTS 🎵 :
  1. SyberMath Shorts
Рекомендации по теме
How To Prove 0:06:58

How To Prove Bernoulli's Inequality

Proof of Bernoulli's 0:05:42

Proof of Bernoulli's Inequality using Mathematical Induction

Using induction to 0:04:33

Using induction to prove Bernoulli's inequality

Bernoulli's Inequality 0:12:13

Bernoulli's Inequality

Bernoulli's Inequality (two 0:04:35

Bernoulli's Inequality (two visual proofs via calculus)

Bernoulli's Inequality: Proof 0:10:05

Bernoulli's Inequality: Proof by Induction

Bernoulli's Inequality Proof 0:03:04

Bernoulli's Inequality Proof using Mathematical Induction | Maths |Mad Teacher

⏺️2. proving Bernoulli's 0:05:10

⏺️2. proving Bernoulli's inequality by mathematical induction

Bernoulli's Inequality 0:02:30

Bernoulli's Inequality

bernoulli inequality proof 0:06:35

bernoulli inequality proof by induction

Proving Bernoulli's Inequality 0:08:22

Proving Bernoulli's Inequality using Mathematical induction

Proof of Bernoulli's 0:04:08

Proof of Bernoulli's inequality

Proof by induction 0:01:16

Proof by induction of Bernoulli's inequality

Bernoulli's Inequality | 0:04:30

Bernoulli's Inequality | Urdu/Hindi

Bernoulli Inequality 0:11:03

Bernoulli Inequality

Induction with inequalities 0:04:07

Induction with inequalities

Application of Bernoulli's 0:03:31

Application of Bernoulli's inequality part 2.mp4

State and proof 0:09:12

State and proof Bernoulli's inequality | in urdu/hindi

Bernoulli's Inequality 0:08:21

Bernoulli's Inequality

Bernoulli Inequality | 0:17:18

Bernoulli Inequality | Inequality EP3

Prove that Bernoulli 0:04:10

Prove that Bernoulli inequality and e^x \geq (1+x)

Cosine: The exact 0:02:21

Cosine: The exact moment Jeff Bezos decided not to become a physicist

BERNOULLI'S INEQUALITY MATHEMATICS 0:03:35

BERNOULLI'S INEQUALITY MATHEMATICS ISI ,DSE ,JNU ,IGIDR ,CSIR NET ,NPTEL ,MIT ,IIT JAM ,UPSC ,...

Albert Einstein doing 0:00:13

Albert Einstein doing physics | very rare video footage #shorts

Комментарии
Автор

Third method:

The two sides if the inequality are equal at x=0. The derivative of the RHS is n, and the derivative of the LHS is n(1+x)^(n-1) >= n, since 1+x > 1. So the LHS is increasing at a faster rate than the RHS, so the LHS must always be at least as large as the RHS

seanfraser
Автор

You could have taken n=0 as your base case

neuralwarp
Автор

How do you use this to prove a sequence is growing sequence

EpsilonDelta-ky
Автор

Why set x > 0 here rather than > -1 like the principal seems to originally state? is it just a simpler approach and the full proof would have a separate case for between -1 and 0?

sammyasher
Автор

Nice." I hope you enjoyed it... don't forget to comment, like..." and you syber don't forget the please let me know after the i hope you enjoyed it!😃💯

yoav
Автор

Thanks 🙏
I'm from the lraq
طالبة علوم رياضيات

Sarahmathematician