filmov
tv
Differentiable implies continuous proof
Показать описание
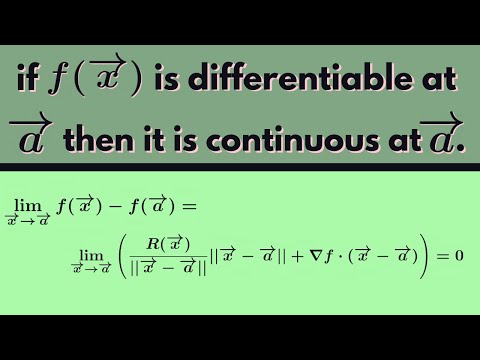
I give a proof that a differentiable function implies we have a continuous function. It is important to note that the converse does not hold; a continuous function does not imply a function is differentiable everywhere. Consider as an example g(x) = | x - 5 | ; it is not differentiable at the point (5,0), but it is continuous.
🧮 Cheerful Calculations! 📐
References:
[1] J.A. Fridy, Introductory analysis: The theory of calculus, 2nd ed., Harcourt Academic Press, San Diego, CA, 2000.
[2] Steven A. Gaal, Point set topology, Dover Publications Inc., Mineola, NY, 2009.
[3] Edward D. Gaughan, Introduction to analysis, 5th ed., American Mathematical Society, Providence, RI, 2009.
Some products I used (I am not sponsored or endorsed by these products):
Samsung Galaxy A10e
TexMaker
Microsoft Paint
#realanalysis
🧮 Cheerful Calculations! 📐
References:
[1] J.A. Fridy, Introductory analysis: The theory of calculus, 2nd ed., Harcourt Academic Press, San Diego, CA, 2000.
[2] Steven A. Gaal, Point set topology, Dover Publications Inc., Mineola, NY, 2009.
[3] Edward D. Gaughan, Introduction to analysis, 5th ed., American Mathematical Society, Providence, RI, 2009.
Some products I used (I am not sponsored or endorsed by these products):
Samsung Galaxy A10e
TexMaker
Microsoft Paint
#realanalysis
0:11:39
Proof: Differentiability implies continuity | Derivative rules | AP Calculus AB | Khan Academy
0:08:21
Proving differentiability implies continuity
0:03:13
Differentiability implies continuity
0:13:48
Differentiable implies continuous proof
0:03:25
Proof that Differentiable Implies Continuous
0:03:28
lec#20.Calc.Theorem#Prove Differentiability implies continuity but the converse is not always true .
0:05:12
Differentiability Implies Continuity
0:05:50
differentiable implies continuous
0:11:24
Differentiable implies continuous
0:03:56
3.5 Differentiable implies continuous
0:05:40
Differentiable Implies Continuous
0:09:11
Continuity vs Partial Derivatives vs Differentiability | My Favorite Multivariable Function
0:03:51
Differentiable then Continuous Proof
0:14:04
Multivariable Calculus | Differentiable implies continuous.
0:32:48
Continuity and Differentiability
0:05:33
Continuity and Differentiability EXPLAINED with Examples
0:08:48
Derivatives: Intro - 10. Differentiable Implies Continuous
0:04:04
27 Differentiable Implies Continuity
0:06:34
3.9 Continuous but not differentiable functions
0:03:55
A Proof that Differentiable Functions are Continuous
0:04:12
Continuous DOES NOT imply Differentiable!
1:15:36
Lecture 18: Weierstrass's Example of a Continuous and Nowhere Differentiable Function
0:48:28
Calculus: derivative as a function, differentiable implies continuous, examples, graphs, 2-2-24
0:07:05
A Differentiable Function is Continuous: Proof
Комментарии