filmov
tv
Dr. Dionyssis Mantzavinos | Long-time asymptotics for the integrable focusing nonlinear Schrödinger

Показать описание
Speaker: Dr Dionyssis Mantzavinos (University of Kansas)
Date: 1st Aug 2024
Title: Long-time asymptotics for the integrable focusing nonlinear Schrödinger equation and proximity of its solutions with those of its non-integrable generalizations
Event: (PDWW01) Frontiers of Dispersive Hydrodynamics
Abstract: Dynamics of ideal fluid with free surface can be effectively solved by perturbing the Hamiltonian in weak nonlinearity limit. However it is shown that perturbation theory for standard variables (Zakharov, 1968), which includes third and fourth order terms in the Hamiltonian, results in the ill-posed equations because of short wavelength instability. Instability growth rate increases with increase of wavenumber so that small scales blow up in arbitrary small time. To fix that problem we introduce the canonical Hamiltonian transform from original physical variables to new variables for which instability is absent and dynamical equations are well-posed.
👉 Want to learn more about the talks in this stream?
-------------------
FOLLOW US
SEMINAR ROOMS
ABOUT
The Isaac Newton Institute is a national and international visitor research institute. It runs research programmes on selected themes in mathematics and the mathematical sciences with applications over a wide range of science and technology. It attracts leading mathematical scientists from the UK and overseas to interact in research over an extended period.
Date: 1st Aug 2024
Title: Long-time asymptotics for the integrable focusing nonlinear Schrödinger equation and proximity of its solutions with those of its non-integrable generalizations
Event: (PDWW01) Frontiers of Dispersive Hydrodynamics
Abstract: Dynamics of ideal fluid with free surface can be effectively solved by perturbing the Hamiltonian in weak nonlinearity limit. However it is shown that perturbation theory for standard variables (Zakharov, 1968), which includes third and fourth order terms in the Hamiltonian, results in the ill-posed equations because of short wavelength instability. Instability growth rate increases with increase of wavenumber so that small scales blow up in arbitrary small time. To fix that problem we introduce the canonical Hamiltonian transform from original physical variables to new variables for which instability is absent and dynamical equations are well-posed.
👉 Want to learn more about the talks in this stream?
-------------------
FOLLOW US
SEMINAR ROOMS
ABOUT
The Isaac Newton Institute is a national and international visitor research institute. It runs research programmes on selected themes in mathematics and the mathematical sciences with applications over a wide range of science and technology. It attracts leading mathematical scientists from the UK and overseas to interact in research over an extended period.
 0:33:54
0:33:54
 0:57:41
0:57:41
 0:46:06
0:46:06
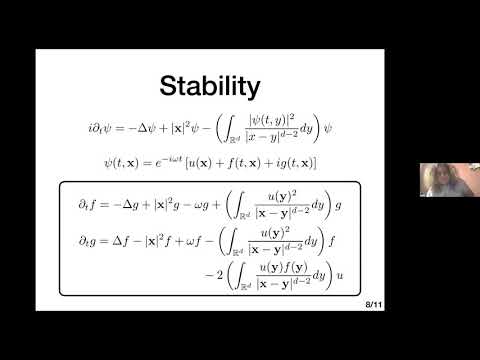 0:25:33
0:25:33
 0:36:10
0:36:10
 0:50:59
0:50:59
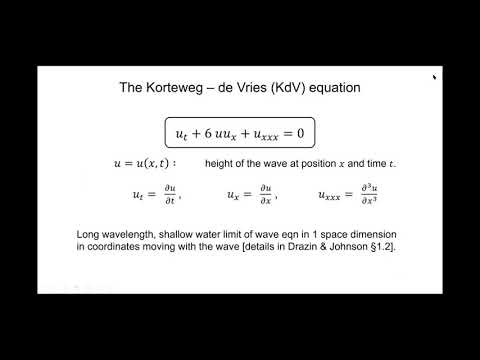 0:37:25
0:37:25
 0:31:31
0:31:31
 0:00:31
0:00:31
 0:52:23
0:52:23
 0:44:18
0:44:18
 0:48:32
0:48:32
 0:49:47
0:49:47
 0:06:33
0:06:33
 0:44:36
0:44:36
 1:22:05
1:22:05
 1:34:04
1:34:04
 0:42:22
0:42:22
 0:52:50
0:52:50
 1:38:27
1:38:27
 1:21:42
1:21:42
 1:18:09
1:18:09
 1:17:51
1:17:51
 0:45:29
0:45:29