Singapore Math - Solving word problems using models : Grade 6 - Percentage, Ratio & Fraction

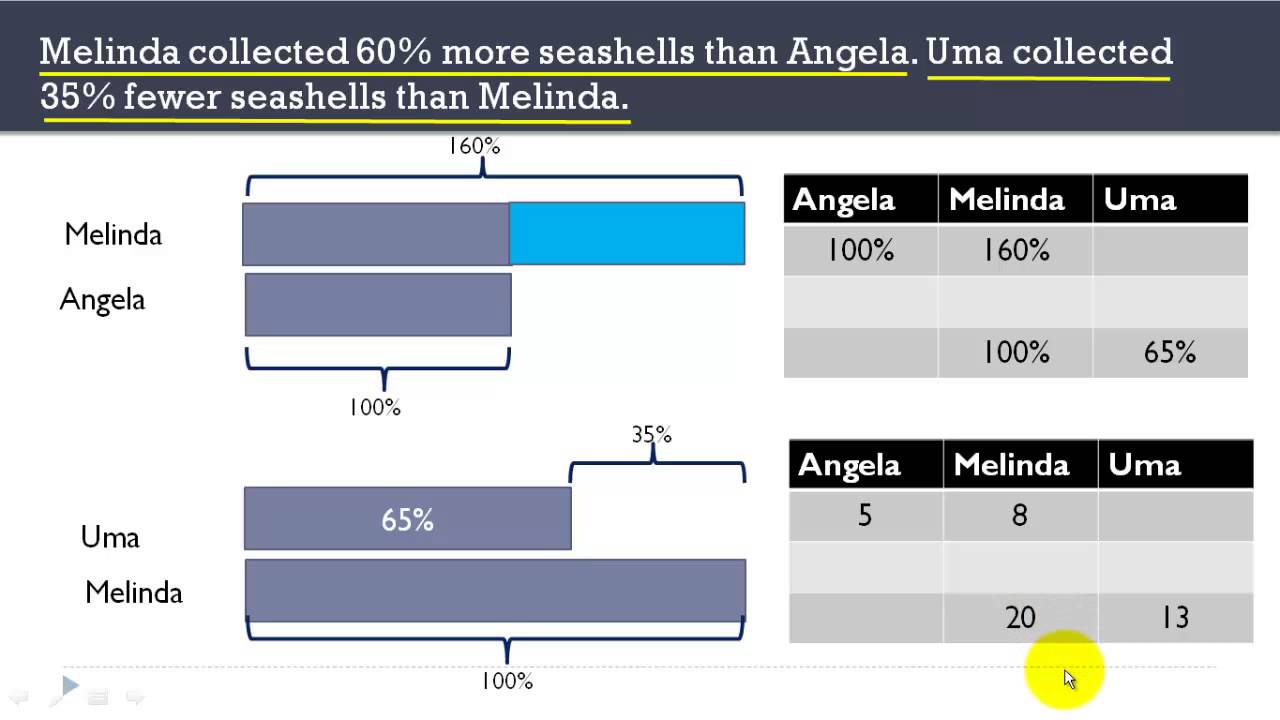
This video covers solving word problems using models. The problem selected includes percentage, ratio and fractions in one problem. A question that seems like a complex problem, is solved by dividing the problem into three small parts and then solving them step by step.

The problem being solved in this video is:
Melinda collected 60% more seashells than Angela. Uma collected 35% fewer seashells than Melinda.
Melinda and Angela gave Uma some seashells in the ratio 3:1. Uma then had 1-1/2 times as many seashells as she had at first.
Given that Melinda had 306 more seashells than Angela in the end, how many seashells did Melinda give Uma?

[blanchesnow]

Informative way to solve this problem. Appreciate your devotion to math. ❤❤❤Please create video teaching us how to solve geometry problem with ratio just like the way you solve this problem

[AronsonDavid]

Thank you for your explanation. I tried this problem using a technique that I have developed, and would appreciate your critique. IMHO, my way is more concise and more straightforward to learn.

I create a three column and two row table with the following column headers: Angela, Melinda and Uma, and the row labels of Initial and Final.

In the first row I filled in the cells as follows: X 1.6X 1.04X

X represents Angela's number. 1.6X represents Melinda's number (60% more than Angela). and 1.04X represents Uma's number, which is 35% less than Melinda which is also equivalent to 65% of Melinda's.

Next I multiplied Uma's 1.04X by the factor 1.5 to represent her final value of 1.56X (50% more than what she initially had).
1.56X minus 1.04X = .52X, the amount gained.

If Melinda and Angela gave in the ratio of 3:1, then Melinda contributed 75% of .52x (or .39X) and Angela contributed 25% of .52X (or .13X).

I then subtracted .39X from 1.6X to arrive at a final value of 1.21X for Melinda.
I subtracted .13X from 1.0X to arrive at a final value of .87X for Angela.

Next I related these final values in the equation: 1.21X = .87X + 306 and solved for X. arriving at X = 900

If X = 900, then .39X, the amount that Melinda contributed is .39 * (900) = 351.

Thanks for your review.

David Aronson

[williamtli]

The Singapore method uses bar models to translate each given statement in the question. It is easy to understand. By doing so, you can see the whole picture of the problem. Then you can break down the question into smaller parts and solve each part step by step. Of course, you can use algebra to solve it, which is pretty easy. But the Grade 6 students may or may not learn enough algebra to solve the problem in North America. Personally, I really like the Singapore method.

[diembui]

Thank you! Your math problem videos are very helpful. I hope you make more videos for grade 6

[bedrockredstone]

I need help :( ;-; could you please tell me where my first problem was? Thanks in advance.

A= Angela
U= Uma
M= Melinda
x=global unknown seashell variable (percentage)

We know that:
Angela has 100% seashells
Melinda has 60% more seashells than Angela
Uma has 35% less seashells than Melinda.

M=100+60%x
=160%x

U=M-35%M=65%M
=65%x*160%x

=10400/10000=104/100=104%x
U=104%x


After collecting

Both Melinda and Angela give Uma y shells. Melinda gives Uma 75%y shells, and Angela gives Uma 25%y shells. (This means that Melinda lost 75%y, and Uma 25%y)

Uma now has 1½U, which means that she got ½U more than she had originally, which means that y=½U

½U=104/2%x=51%x
Therefore, y=51%x

Therefore, Melinda gave Uma 75%*51%x shells, which is 75*51/100*100, which is 3825/10000, which is 38.25/100, which is 38.25%x. Therefore, Melinda lost 38.25%x shells, which is equal to 160-38.25%x, which is equal to 121.75%x shells. Therefore, Melinda now has 121.75%x shells.

Angela gave Uma 25%*51%x shells, which is 25*51/100*100, which is 1275/10000, which is 12.75/100, which means that Angela gave Uma 12.75%x shells. This means that Angela lost 12.75%x shells, which means that her total is 100-12.75%x, which is 87.25%x. This shows that Angela's new balance of seashells is 87.25%x.

Considering that now, Melinda has 306 more shells than Angela, this shows that the difference in the two would also equal 306, which we can express as 121.75-87.25%x=306 shells, which we can further simplify as 34.50%x=306 shells. Now, x can be isolated by dividing both parts of the equation by 34.50, giving us (34.50/34.50)%x=306/34.50 shells, which can be simplified as 1%x=204/23 shells, which is about 9.

If Melinda gave Uma 38.25%x shells, that means that Melinda gave Uma (204/23)*38.25 shells, which is equal to (204*38.25)/23 shells, which is equal to 7803/23 shells, which is not 36. Where did I go wrong??

[sf]

Great video. How would this be solved algebraically?

[alexischeong]

Hi. Thanks so much for your sharing. It's so helpful. I have a word problem and would appreciate if you could share your solution - Sara's age is 3/7 of Tom's age. In 18 year's time, her age will be 3/5 of Tom's age. How old will Tom be by then?

[anxious]

This helps me soooo much bc I suck at math :/ thanks for making this

[buttercoconut]

I ummm sorry sir I am very confused 😕 ahh why in the where there is the simplified bumbers of the percentage (of how much sea shells they have) how can it happen that the 1st row we gonna divide it to 5
and in the second row why we are gonna divide it to 2???? Please answer my question!!!! I need to know this thing quickly!!!! 😔

[Kino-Imsureq]

"how many seashells did Melinda give Uma"
Makes me feel uncomfortable ._.

[buttercoconut]

I love this video can you make more you are very ♥♥

[Kino-Imsureq]

M = A + 60% "Although ratio 3:1 is technichally 3 xD" "Melinda has 306 more seashells than Angela"
U = M - 35% = A + 60% - 35% = A + 25%

A [ 100 ]
M [ 100 ] [ 60 ]
U [ 100 ] [ 25 ] | 35 |

dU = U * 1.5 = (A+35%)*1.5 = 1.5A+52.5%
R = 3:1 "Melinda gave 3 times more than Angela?"
T = dU - U = U/2 = A/2 + 12.5%
M1 = 3T/4 = (3A/2 + 37.5%)/4 = 3A/8 + 9.375% " A = 100% " = 300%/8 + 9.375% = 37.5% + 9.375% = 46.875%

Melinda gave 46.875% of her seashells.
M - 46.875% = 306
160% - 46.875% = 306
160% = 306 + 46.875%
0 = 306 + 46.875% - 160% = 306 - 113.125%
113.125% = 306 "113.125% of Melinda's seashells is 306"
46.875% = ?

[lorizizza]

This is fantastic! Please help me with the following problem. Would love to use the methods you use to solve the problem in the video.
The ratio of the number of boys to the number of girls in a hall was 3:2 at first. After 30 boys left the hall, the ratio became 2:3. How many boys were there in the hall at first?

[lhian_min]

Ummm sir can i ask you a question ummm can you give me the whole formula to solve that problem thank you sir

By the way i am your new subscriber 😁😁