filmov
tv
Induction: Fibonacci Sequence

Показать описание
Induction: Fibonacci Sequence
Proof by strong induction example: Fibonacci numbers
Proofing a Sum of the Fibonacci Sequence by Induction
Prove with Induction and Fibonacci Numbers
Induction Fibonacci
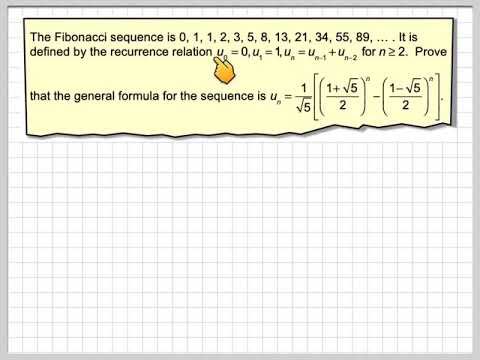
The general formula of Fibonacci sequence proved by induction
Proving the Sum of Odd Numbered Fibonacci Numbers with Mathematical Induction
Proofing 3 Different Equations About the Fibonacci Sequence by Induction
Proofing a Sum of the Fibonacci Sequence Without Induction
Mathematical induction | Appendix A | Fibonacci Numbers and the Golden Ratio
Example: Mathematical Induction (2)
Basic properties of Fibonacci Sequences using Mathematical Induction
Fib induction proof
Induction proof on Fibonacci sequence | Prof. Soborno Isaac Bari
Proof by induction | Sequences, series and induction | Precalculus | Khan Academy
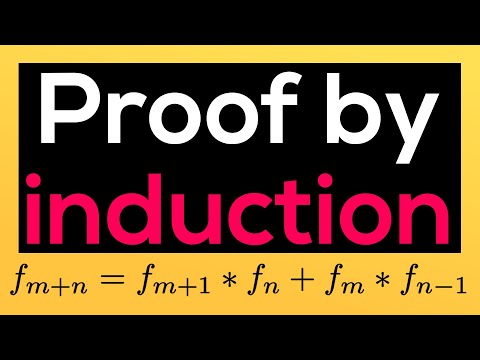
Proof F(m+n) = F(m+1) * F(n) + F(m) * F(n-1) by Induction (Fibonacci Sequence)
Golden Proof - Numberphile
Day 1 : Math Circle at Columbia | Proof by Induction | Fibonacci sequence
The magic of Fibonacci numbers | Arthur Benjamin | TED
Integers as Sums of Fibonacci Numbers
Sum of Fibonacci numbers | Lecture 9 | Fibonacci Numbers and the Golden Ratio
Mathematical Induction Practice Problems
The Fibonacci sequence
Number Theory: Fibonacci Sequence and Proof By Mathematical Induction
Комментарии
 0:21:20
0:21:20
 0:10:56
0:10:56
 0:02:25
0:02:25
 0:09:18
0:09:18
 0:09:32
0:09:32
 0:08:54
0:08:54
 0:03:39
0:03:39
 0:03:59
0:03:59
 0:02:25
0:02:25
 0:07:07
0:07:07
 0:08:14
0:08:14
 1:03:54
1:03:54
 0:10:35
0:10:35
 0:07:35
0:07:35
 0:09:23
0:09:23
 0:04:21
0:04:21
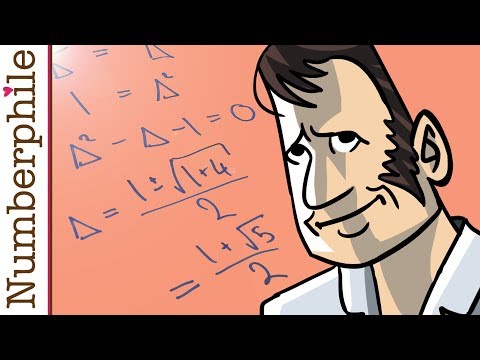 0:04:56
0:04:56
 0:03:58
0:03:58
 0:06:25
0:06:25
 0:10:24
0:10:24
 0:08:44
0:08:44
 0:18:08
0:18:08
 0:07:57
0:07:57
 0:11:55
0:11:55