filmov
tv
What's My Quantum Homework on This Week

Показать описание
Today I go over the topics that this weeks quantum homework is on, without going over specific problems (university policy). Mostly just talk about commutators tbh.
What's My Quantum Homework on This Week
How My Quantum Quiz Went!
Undergraduate Quantum Mechanics Homework | Perturbation Theory
What Will Be On My Quantum Final?
What My Quantum Field Theory Homework Looks Like
What Will My Graduate Quantum Final Be On
Schrodinger equation comment response and homework answers video
Special Relativity Homework For Quantum Field Theory
Arcturian Message of Love | Quantum Healing & High-Frequency Meditation
This is what my hw looks like as a math grad student 😅
Quantum Mechanics Video Solution Assignment
I'm busy studying, so here's my quantum computing assignment...
when you don't complete assignment on time😂 #shorts
Brian Cox explains quantum mechanics in 60 seconds - BBC News
How To Determine The 4 Quantum Numbers From an Element or a Valence Electron
Discussion on assignment 1 - Introduction to quantum mechanics
Griffith Quantum Mechanics Solutions 1.1
So Basically This Is Epic: Quantum Mechanics II Course Outline
Question -3 | Quantum Chemistry Assignment
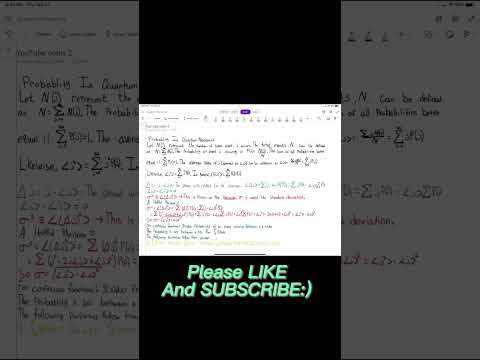
Quantum Mechanics Notes with Classical Music: Probability in Quantum Mechanics
Quantum Mechanics Lecture 05 Homework
How Stitches Work 😯
A Day in the Life of a Physics Major
I Have A Quantum Quiz Next Week
Комментарии
 0:04:51
0:04:51
 0:03:29
0:03:29
 0:07:01
0:07:01
 0:02:29
0:02:29
 0:04:55
0:04:55
 0:04:14
0:04:14
 0:03:21
0:03:21
 0:07:57
0:07:57
 1:02:53
1:02:53
 0:00:15
0:00:15
 0:09:43
0:09:43
 0:10:44
0:10:44
 0:00:15
0:00:15
 0:01:22
0:01:22
 0:04:25
0:04:25
 0:27:51
0:27:51
 0:07:06
0:07:06
 0:06:07
0:06:07
 0:00:57
0:00:57
 0:00:15
0:00:15
 0:58:19
0:58:19
 0:00:32
0:00:32
 0:00:28
0:00:28
 0:04:05
0:04:05