filmov
tv
Given, \( \mathbf{a}+\mathbf{b}+\mathbf{c}+\mathbf{d}=0 \), which of the following statements ar...

Показать описание
Given, \( \mathbf{a}+\mathbf{b}+\mathbf{c}+\mathbf{d}=0 \), which of the following statements are incorrect?
(a) \( \mathbf{a}, \mathbf{b}, \mathbf{c} \) and \( \mathbf{d} \) must each be a null vector.
(b) The magnitude of \( (\mathbf{a}+\mathbf{c}) \) equals the magnitude of \( (\mathbf{b}+\mathbf{d}) \)
(c) The magnitude of a can never be greater than the sum of the magnitudes of \( \mathbf{b}, \mathbf{c} \) and \( \mathbf{d} \).
(d) \( \mathbf{b}+\mathbf{c} \) must lie in the plane of \( \mathbf{a} \) and \( \mathbf{d} \), if \( \mathbf{a} \) and \( \mathbf{d} \) are not collinear and in the line of \( \mathbf{a} \) and \( \mathbf{d} \), if they are collinear.
(a) \( \mathbf{a}, \mathbf{b}, \mathbf{c} \) and \( \mathbf{d} \) must each be a null vector.
(b) The magnitude of \( (\mathbf{a}+\mathbf{c}) \) equals the magnitude of \( (\mathbf{b}+\mathbf{d}) \)
(c) The magnitude of a can never be greater than the sum of the magnitudes of \( \mathbf{b}, \mathbf{c} \) and \( \mathbf{d} \).
(d) \( \mathbf{b}+\mathbf{c} \) must lie in the plane of \( \mathbf{a} \) and \( \mathbf{d} \), if \( \mathbf{a} \) and \( \mathbf{d} \) are not collinear and in the line of \( \mathbf{a} \) and \( \mathbf{d} \), if they are collinear.
 0:06:20
0:06:20
 0:07:48
0:07:48
 0:07:48
0:07:48
 0:00:10
0:00:10
 0:02:00
0:02:00
 0:06:06
0:06:06
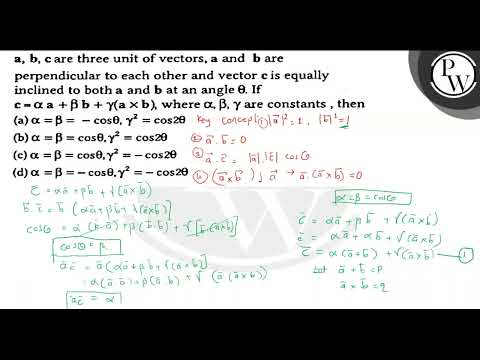 0:12:10
0:12:10
 0:09:39
0:09:39
 0:03:06
0:03:06
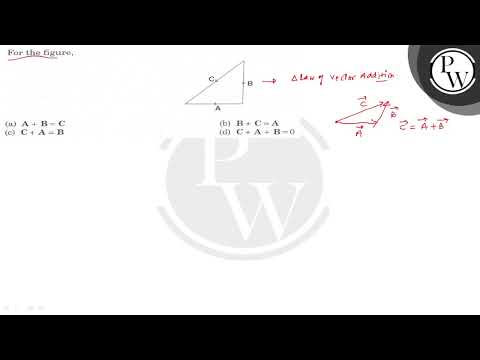 0:01:39
0:01:39
 0:03:07
0:03:07
 0:05:10
0:05:10
 0:05:21
0:05:21
 0:06:38
0:06:38
 0:05:15
0:05:15
 0:05:00
0:05:00
 0:03:04
0:03:04
 0:11:44
0:11:44
 0:11:44
0:11:44
 0:06:48
0:06:48
 0:07:40
0:07:40
 0:02:47
0:02:47
 0:06:18
0:06:18
 0:00:21
0:00:21