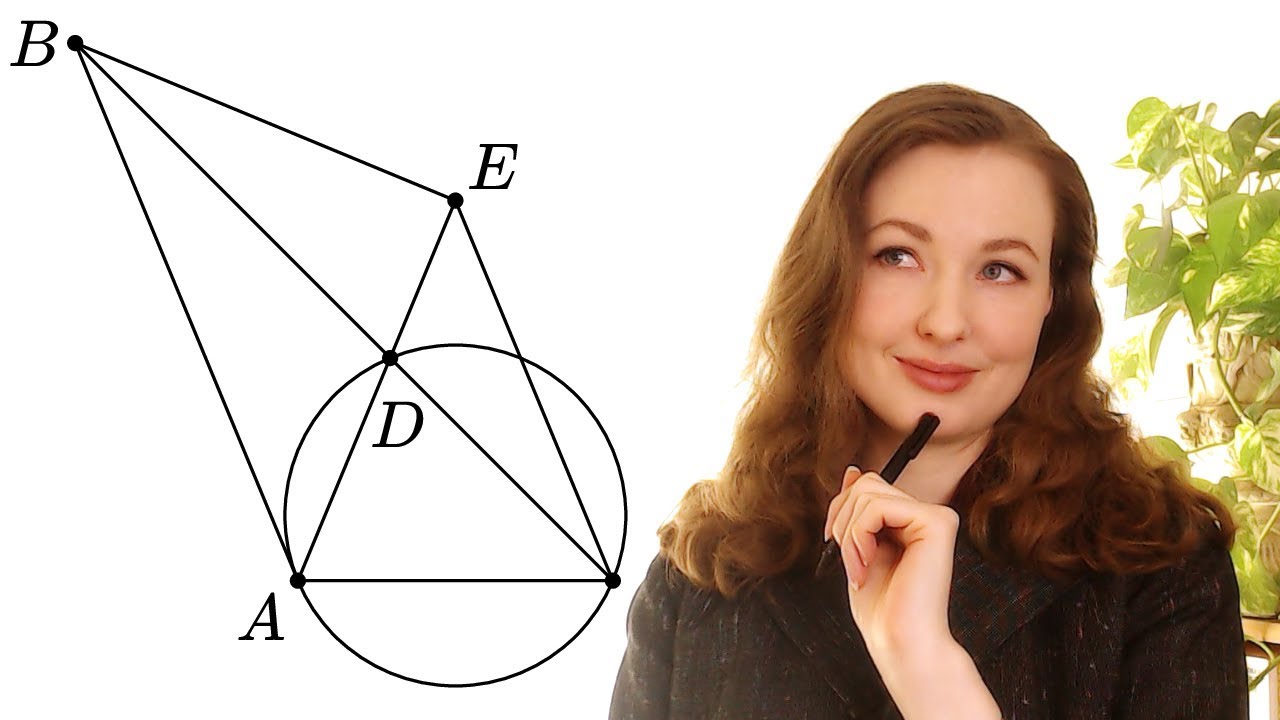
Maths Olympiad Questions - 2019 INMO Q1

A circle geometry problem from the 2019 Indian National Mathematical Olympiad. I'll go through two solutions, the first an elegant classical/synthetic proof, and the second a messy (but straightforward to come up with) modern solution using the sine rule and lots of algebra.

[uxinox]

I think your videos are destined to become classics and the ones for younger students will launch many a mathematical career. So Congratulations!  I love your videos. They do not labour the obvious. Ideas are conveyed succinctly. The graphics are great. I loved those bubbles that decompose and reassemble numbers, but I love the other stuff too. The neat writing, the freehand diagrams that somehow manage to look like they are supposed to. Not too big or too small and lines that are just straight enough! I am not forgetting your prodigious mathematical ability, but there are so many ways to be a mathematical genius and still make videos that are a disaster! The internet is flooded with them. So, keep up the good work. You are a pearl of great price!

[ishikameher]

I found her voice so calming, and I had a test the day after. Completely cleared my questions. Thank you!

[srinivasadireddi]

OMG. I came across your channel today and oh my! I genuinely understand the whole process, your making math more intuitive!

[beanhwak]

Draw a perpendicular from E to AB. Call the foot of this perpendicular F. AFE and EFB are congruent triangles.(EF is common side, <AFE = <BFE = 90, <BEF = 90 - <FEA= <EFA). Hence BE = AE. But BE=AE=CE, so E is the circum-center. By angle at center = 2 x angle circumference, BCA = (1/2) x <BEA= (1/2)x90 = 45. Thank you for the problem, I used your solution for teaching the trigonometry.

[wesleysuen]

Just past 6:00, we see that triangle BFA is right-angled and isosceles, and so angle BFA is 45 degrees, and hence so is angle BCA, and we are done.

[tthtlc]

your series was one of my dream: to work out some olympiad problems a video. i wish you will continue doing that.

[abhipriyeshukla]

Ma'am you are awesome thank you so much not many people solve these questions queen of math literally!!

[apratimghosh]

This was the question which messed up my paper, I didn't go on to the next round. But it's fine, I did make it through this year :)
EDIT: No, I didn't make it to IMO, check 'Apratim Ghosh' in IMOTC 2020 list. @Mohd Sufyan the cutoff was high, like ~50. I don't remember exactly, it was a while back.

[rhythmmandal]

me: I wonder what would happen if drew circle over ABC, nah wont work.
YouTube video: Draws a circle around ABC.
me: Lesson Learned never doubt instinct.

[sondo]

The way to convey the solution of the problem is slow and easy to understand. Explore the problem, we will see many interesting results!. Thank you

[bachirblackers]

There are more than simple solutions connecting C to the center of circle ADC and solve for x in quicker way for example draw the bisector of Angle C passing by the center and intersecting AB At point let say M then connect M to D also construct AO and belive me you ll find 1/2 angle C and betta every where however the most beautiful key is the angle made by BC cross AO IF O is the center of circle ACD

[matejcataric]

I just saw your channel and I am lovin it!

[sandy]

I am from India but I like the way you solve the problem

[adityachakrabarty]

BE=AE=EF B, E and f are collinear bef is the diameter angle abf is 90° angle eba is thus 45 and so is dab and hence bca

[narendrayeole]

Thanks dear respected madam God bless you and your family....Tc...lol....

[aryanrawat]

Really true. These constructions are actually impossible. Leave alone the exam you wont be able to think about any construction working on the question for days at home

[mathswithrbsingh]

Really, it's a great problem from IMO & u've also provided a good idea.I'm really impressed... & I've pressed like & subscribe button together without taking a few second😉😃

[waiphyoemg]

can anyone help me? Factorise (p-q)r^3 - (p-r)q^3 + (q-r)p^3.

[zeldner]

Seems to me that the first solution doesn't include the fact that AC=DC. Is that possible?

[aiseop]

Tell me a book that can guide me from beginning level geometry to imo level geometry.