filmov
tv
SURFACE AREA of a SPHERE SECTION

Показать описание
If you're here to read some WACKY nonsense involving one of the three main characters, you're in luck. The WILDEST text EVER will reveal itself in a dream to you IF AND ONLY IF you want this video without skipping the ad and watching all the way till the end.
🌟Support the channel🌟
🌟my other channels🌟
🌟My Links🌟
🌟How I make Thumbnails🌟
🌟Suggest a problem🌟
But why is a sphere's surface area four times its shadow?
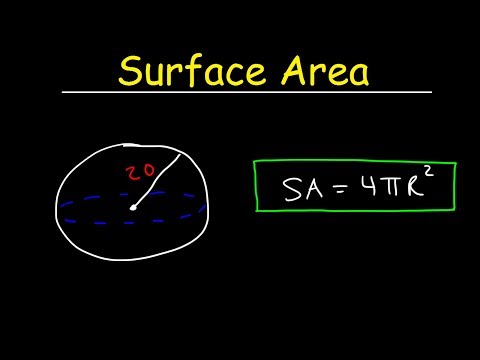
Surface Area of a Sphere
Sphere surface area proof sketch
Volume and Surface Area of a Sphere Formula, Examples, Word Problems, Geometry
Surface Area of a Sphere explained in easy way | no need to memorise
Visual proof of:Surface area of Sphere and Volume of sphere#maths #mathematics
Surface Area of Sphere | Beat the Calculator #shorts
Surface Area of a Sphere - Nerdstudy
Mensuration Part 2: Surface Area & Volume | Master Tricks by Maneesh Sir 🔥
How do you find the surface area of a sphere
Surface Area Of A Sphere | Geometry | Math | Letstute
🫡Why a sphere's surface area is 4πR^2 #how #ytshorts #proof
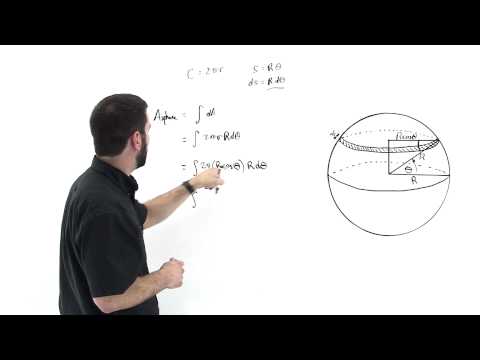
Surface Area of a Sphere (equation derived with calculus)
Surface Area of a Sphere - Visual Explanation and Example (Mastering Geometry)
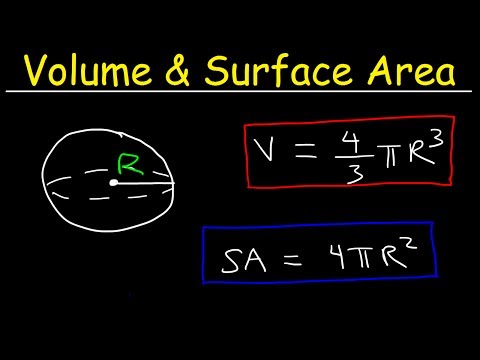
Sphere Surface Area and Volume
Sphere Surface Area
Surface Area of Spheres (1 of 2: Finding the Surface area of spheres and hemispheres)
Geometry#competitive #Surface area and volume #cube#cuboid#Cylinder#Cone#Sphere#Hemispheres#shorts
calculating the surface area of sphere.
How to Use Surface Area Sphere Formula
Sphere and Hemisphere: Diagram, volume, surface area.... Important formulae #shorts
Volume and Surface Area of Spheres (GMAT/GRE/CAT/Bank PO/SSC CGL) | Don't Memorise
Finding the Surface Area of a Sphere
Volume and Surface Area of Spheres - GCSE Maths
Комментарии
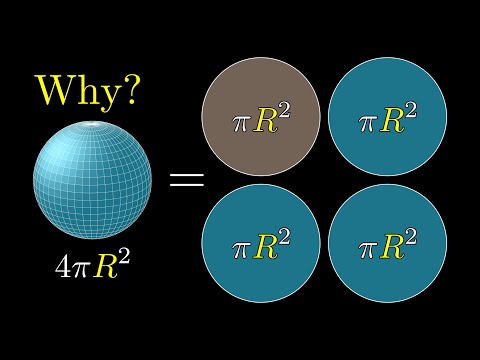 0:15:51
0:15:51
 0:01:20
0:01:20
 0:01:25
0:01:25
 0:11:09
0:11:09
 0:03:45
0:03:45
 0:00:15
0:00:15
 0:00:46
0:00:46
 0:02:39
0:02:39
 0:59:15
0:59:15
 0:01:47
0:01:47
 0:07:40
0:07:40
 0:00:59
0:00:59
 0:05:14
0:05:14
 0:05:32
0:05:32
 0:01:55
0:01:55
 0:00:20
0:00:20
 0:07:23
0:07:23
 0:00:05
0:00:05
 0:00:10
0:00:10
 0:03:53
0:03:53
 0:00:32
0:00:32
 0:03:20
0:03:20
 0:07:50
0:07:50
 0:10:26
0:10:26