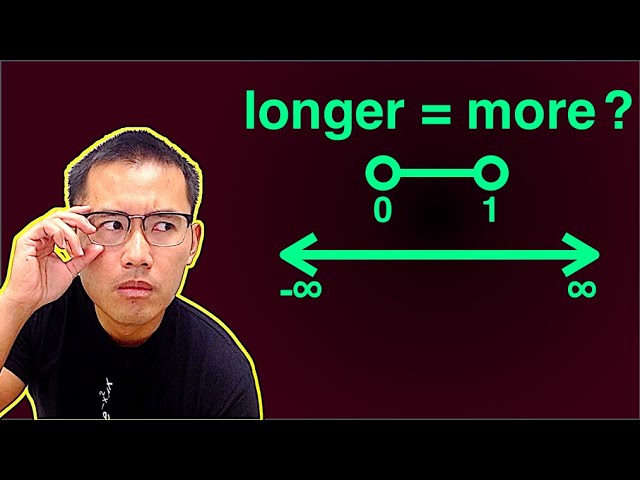
Bijection between (0,1) and (-inf, inf)

Which interval contains more real numbers: (0, 1) or (-inf, inf)?



blackpenredpen,
math for fun

[blackpenredpen]

Which one do you like: longer or more?

[natealbatros]

it's so weird, every element between 0 to 1 is inside the other interval and even more, but somehow they have the same amount of elements.

[majkgmajkg]

When I was younger I have read about alef zero and continuum and then I found out that (-inf, +inf) contains the same number of elements as (0, 1). I explained it to myself like this:
when you have (-inf, inf) you can add infinity to both ends and you get (0, inf). then you divide by infinity you get (0, 1). I knew that wasn't correct but for me sounded like pretty good explanation xD.


Great Video!

[WarmongerGandhi]

There's a geometric construction for this problem I really like.

Draw the interval (0, 1) vertically along the y axis. Draw the line y=1/2. Pick a point P with coordinate (p, 1) where p > 0 and a point Q with coordinate (-p, 0). Now for any x >= 0, we draw a line connecting Q and (x, 1/2). For x < 0 we draw a line connecting P and and (x, 1/2. Thus for each x in (-inf, inf) we have a corresponding y in (0, 1).

[BrainGainzOfficial]

One interesting thing I've noticed about the past few videos you've posted that have to do with infinite sets is that there always seems to be a few people in the comments rejecting the idea and claiming it is impossible / incomplete / incorrect, purely based on the fact that it goes against their intuition. I'm not saying it's a bad idea to be a skeptic or question things. In fact, it's probably a productive habit for a mathematician. But some people stop there and miss out on the most important part, which is researching, digging, and trying to find truth and clarity. This is what makes a great mathematician. It's not about intelligence. It's about a willingness / desire to search for truth. I hope this comment encourages someone who is skeptical / confused to do some digging. Infinite sets can be mind - boggling, but that's also what makes them so interesting to learn about. Anyways, great video as always BPRP.

[paulamigotka]

Hello Blackpenredpen!
For a long time I have a big problem with that eguation:
"Solve the equation

This problem comes from math olympiad (Russia, 95). I know that it doesn't have any solutions, but the problem is that we have to proof it. I saw solution to this but I don't unterstand it.

I am your fan, best regards!

[MaksymCzech]

Let me guess, both sets have same *cardinality?*

[absoluterefusal]

Here is the deal: your channel is excellent! Thanks!

[nanakokuroi]

When I studied at 1 year my calculus teacher asked me about this and I was so confident that longer means more... can never forget his sad face 😔

[Albkiller]

Wow when I did discrete math we didn't use these kind of functions for the bijection lol

[johannbauer]

Every neural net programmer: Sigmoid function! (B -> A), but still bijective!

[raijinvolante]

Hey bprp, I thought of f(x)= 1/x - 1/(1-x), and using derivatives you can show that on (0, 1) the function is strictly decreasing, which means it is injective. Then you take the limits at the endpoints, which evaluate to -inf and +inf. If someone has trouble with trig functions, I think this is a little easier. Anyway great vid as always. Love from Italy

[egillandersson]

I remember thatI read about this incredible fact when I was 12, in a book titled "Les nombres et leurs mystères" by André Warusfel. I thing this is one of reason of my interest in mathematics.
Thank you for this video.

[krischan]

I think a lot of the confusion and disagreement comes from the use of words like "more" or "less". These terms are only useful for other applications, like their elements.

For example, if matter didn't consist of atoms, but of uncountably infinitely many particles, two kilograms of it would be more than one kilogram, but you cannot make such a statement about their amounts of particles

[ahmadkalaoun]

Cot(πx) is the same as tan(π(x-1/2)) because
tan(θ-π/2)=cot(θ)

[samuelgunter]

I prefer the Sigmoid Squishification Function, which is 1 / (1 + e^-x)

[JuanMataCFC]

i used y = cot(πx) as my function from A to B. i found this easier than using tan because the domain of cot is (0, π) which is much easier to get from (0, 1) than when negative numbers are involved.

[LiuyingHuang]

omg this is awesome! thanks for explaining this clearer

[milocarteret]

Definitely stumps me. A is a subset of B, where B is not a subset of A. So, any element of A is an element of B, but not all elements of B are also elements of A. And yet, through the bijection, for any element of B there exists a singular element of A to pair it with. Turns my brain to pudding

[travishayes]

I came up with a piecewise function. For domain (0, 1), if x \neq \frac{1}{2}, f(x) = tan(pi x), otherwise f(x) = 0.