filmov
tv
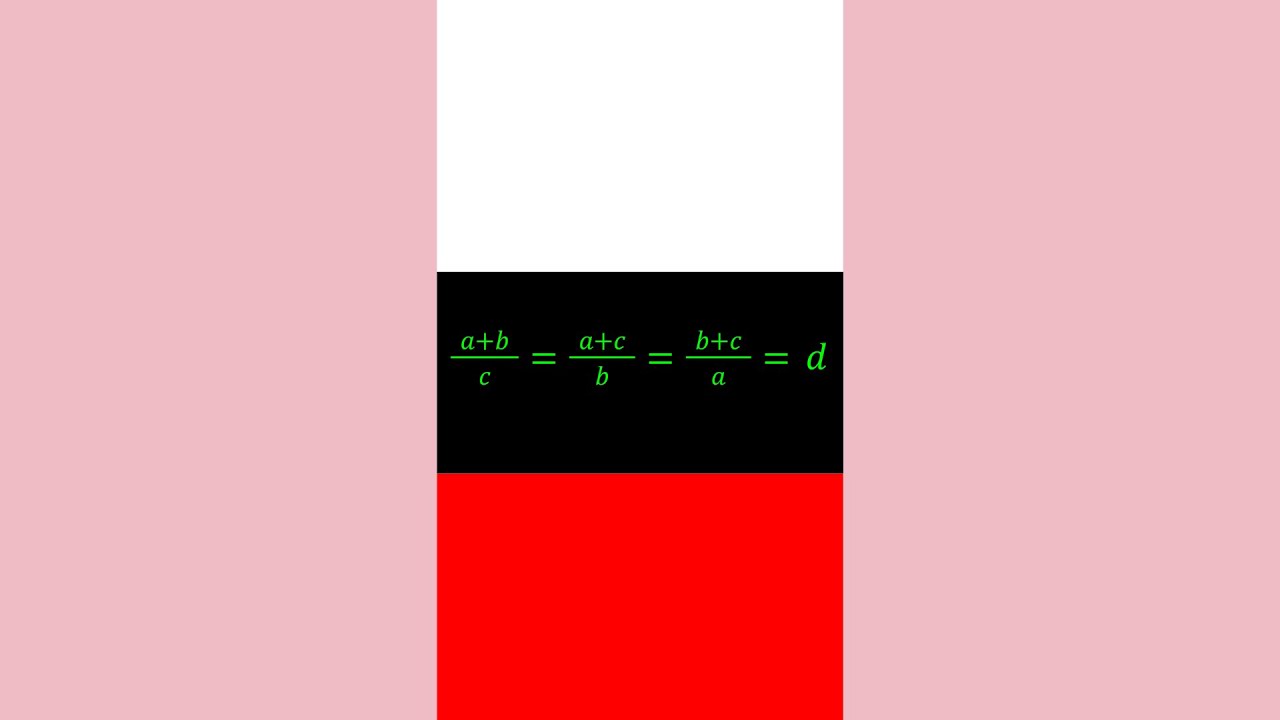
Working with Ratios and Proportions
Показать описание
#Shorts #RatiosAndProportions via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
Ratio and Proportion Word Problems - Math
Word Problems Ratios
Math Antics - Ratios And Rates
Proportions | Solving Proportions with Variables
How to Solve Proportions | Math with Mr. J
Ratios | All About Ratios
Math Antics - Proportions
RATIOS, RATES OF WORK AND PROPORTIONS.
➗ Ratio and Proportion | Mathematics Simplified | Defence Education Academy
Ratios Rates and Proportions
Visualizing Proportional Reasoning: Working With Ratios and Proportions
Working with Ratios and Proportions
Introduction to Ratios (What Are Ratios?) | Ratio Examples and Answers
Fractions & Proportions : Ratios & Proportions in Math
Aptitude Made Easy | Ratio & Proportions -1| Basics and Methods, Shortcuts, Tricks by Pavan Jais...
Art of Problem Solving: Introducing Ratios
Let’s PRACTICE Ratios, Rates and Proportions…step-by-step…
How to calculate ratio - sharing money GCSE question
Ratio and Proportion Nursing Dosage Calculations Made Easy
Ratio of Boys to Girls C8
What is a Ratio in Math? Understand Ratio & Proportion - [6-3-1]
Ratios Maths literacy | Simplify
Ratio, Proportion & Rates.
Advanced n Sharing in ratios converted
Комментарии
 0:13:27
0:13:27
 0:00:35
0:00:35
 0:08:49
0:08:49
 0:03:46
0:03:46
 0:08:36
0:08:36
 0:05:57
0:05:57
 0:10:30
0:10:30
 0:03:20
0:03:20
 0:20:50
0:20:50
 0:15:15
0:15:15
 0:06:16
0:06:16
 0:00:53
0:00:53
 0:05:06
0:05:06
 0:02:02
0:02:02
 0:04:49
0:04:49
 0:05:56
0:05:56
 0:17:21
0:17:21
 0:04:25
0:04:25
 0:10:05
0:10:05
 0:02:47
0:02:47
 0:20:59
0:20:59
 0:09:37
0:09:37
 0:30:11
0:30:11
 0:01:31
0:01:31