Emergence of Chaos in Damped-Driven Nonlinear Pendulum

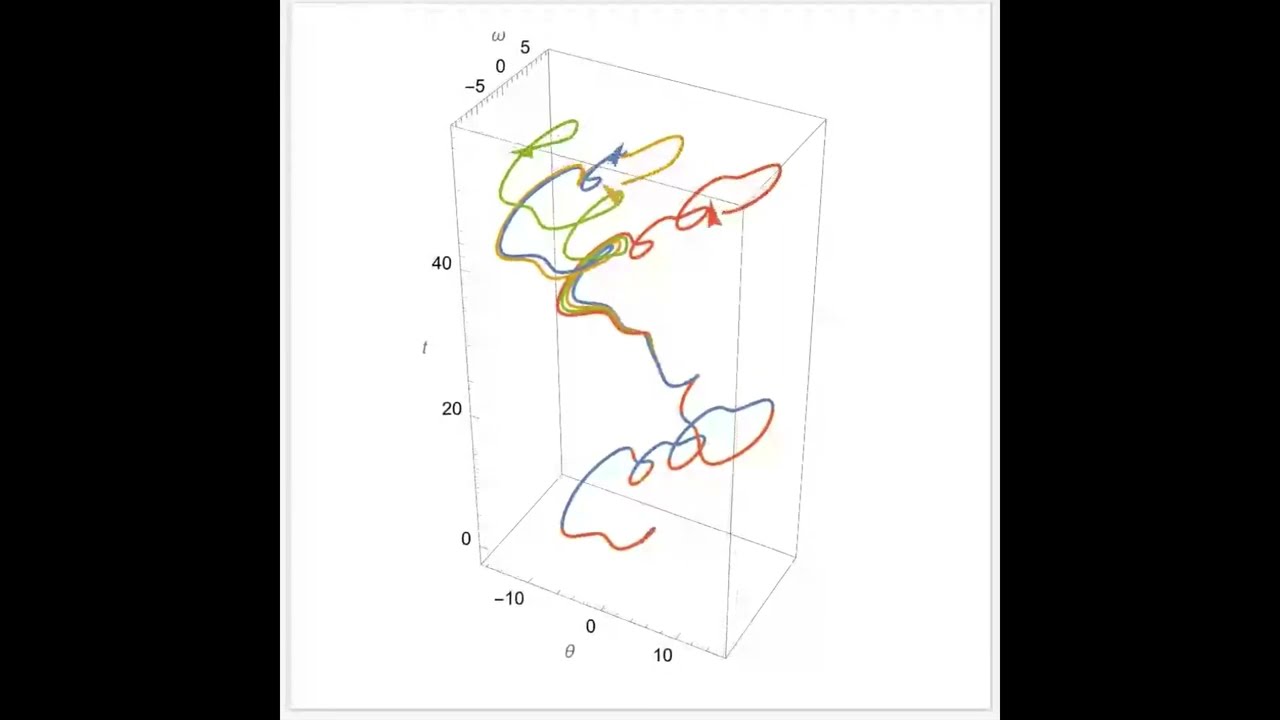
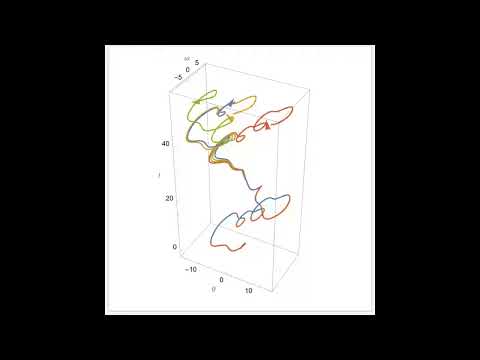
This animation was made in Mathematica and it shows the deviation in phase trajectory for damped-driven nonlinear pendulums with similar initial conditions ($0.147\leq\theta(0)\leq 0.150$). This demonstrates the Poincaré–Bendixson theorem, showing that chaos emerges when the phase space is 3-dimensional and there is at least 1 nonlinear coupling. Here $t$ is the time elapsed, $\theta$ is the angular displacement, and $\omega$ is the angular velocity.