Knorrer periodicity in curve counting

Young-Hoon Kiem speaks at the CMSA Simons Collaboration Workshop on Homological Mirror Symmetry and Hodge Theory, held January 10-13.

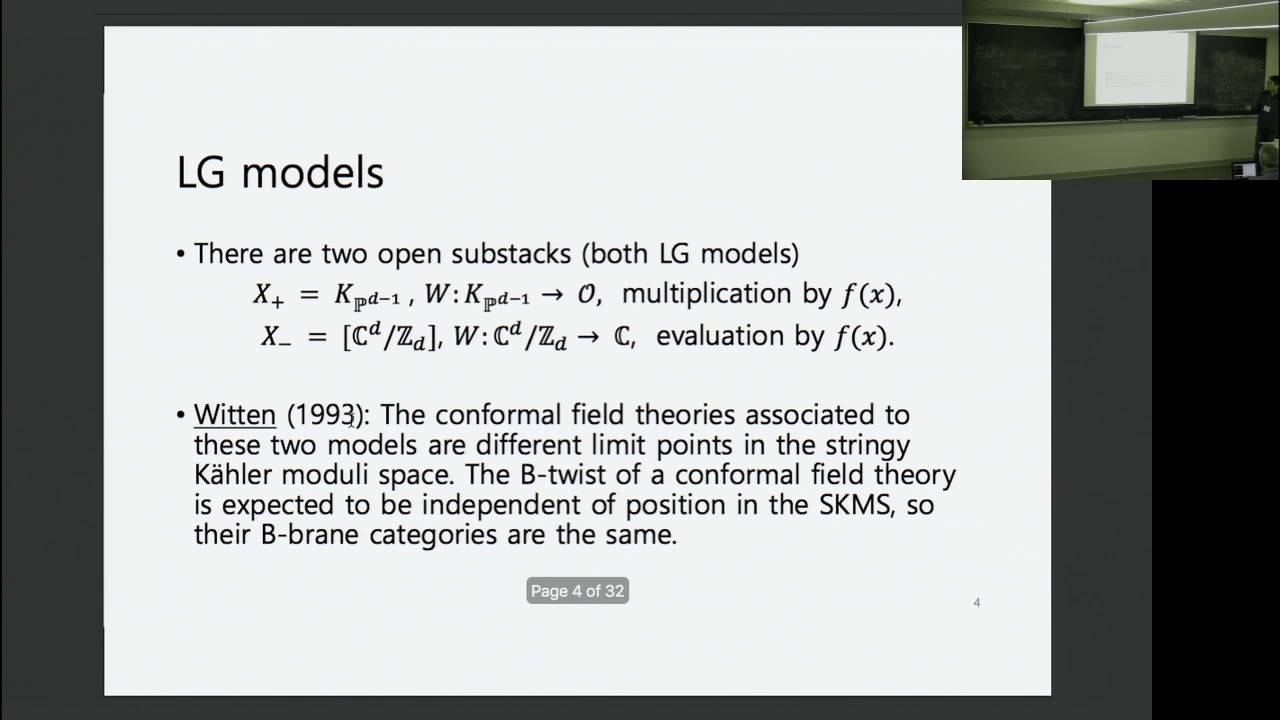
Abstract: The derived Knorrer periodicity compares the derived category of coherent sheaves on a projective hypersurface with that of matrix factorizations of its defining equation. I’d like to talk about a parallel development in curve counting, including Chang-Li’s p-field invariant, Chang-Li-Li’s algebraic theory of (narrow) FJRW invariant and Polishchuk-Vaintrob’s cohomological field theory, from the viewpoint of cosection localization.