filmov
tv
A System of Equations from Moscow | Incorrect Solution
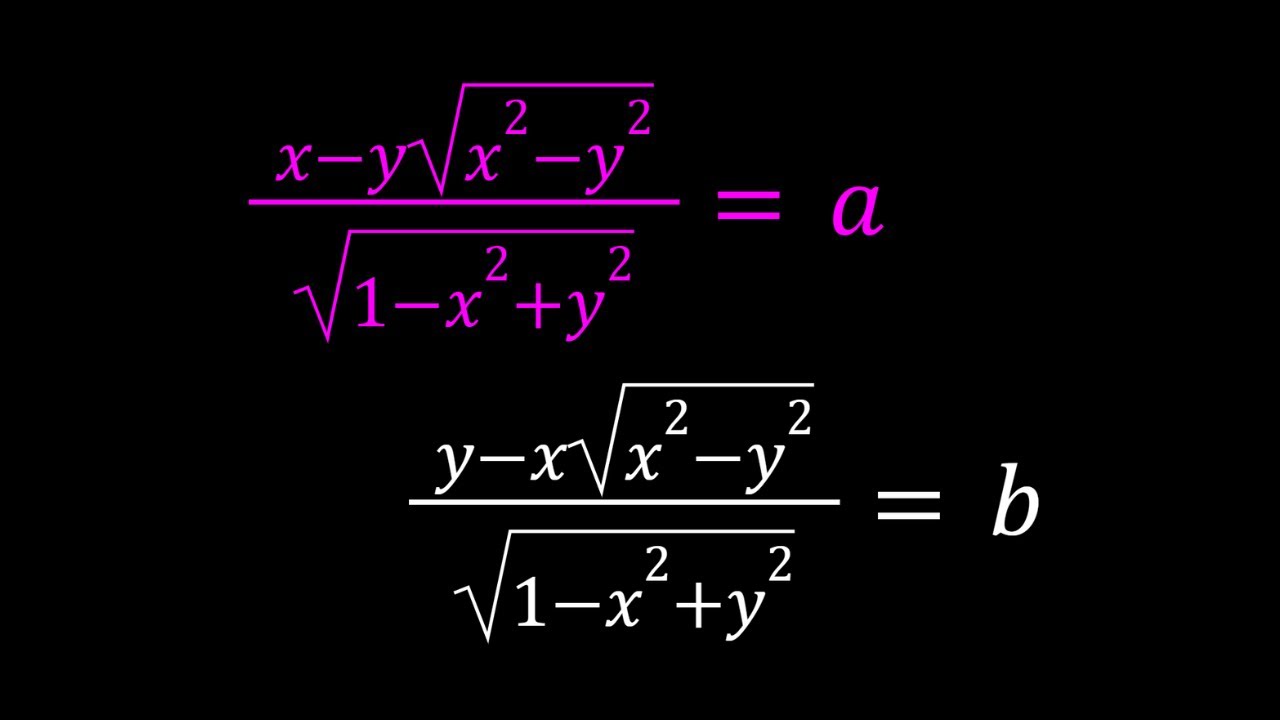
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts)
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #RadicalEquations #Radicals
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
#ChallengingMathProblems #RadicalEquations #Radicals
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Solving Systems of Equations By Elimination & Substitution With 2 Variables
How do we solve a system of linear equations using any method
Solving Systems of Equations By Graphing
SOLVING SYSTEMS OF EQUATIONS STEP-BY-STEP!
Solving systems of equations by elimination | Algebra Basics | Khan Academy
Linear Algebra - 27 - Algebraic Systems of Equations with Matrices
Substitution Method to Solve a System of Equations
Solving Systems of Equations With 3 Variables & Word Problems
Let's Get to 1000 Subs...Solving Simultaneous Equations 1 Linear 1 Quadratic #simulatneous Equa...
Learn to solve a system of equations using substitution
Solving a system of equations by substitution
Elimination Method For Solving Systems of Linear Equations Using Addition and Multiplication, Algebr
Solving Systems of Equations... Elimination Method (NancyPi)
Solve a system of linear equations using substitution
One Solution, No Solution, or Infinitely Many Solutions - Consistent & Inconsistent Systems
13 - Overview of Systems of Linear Equations (Simultaneous Equations & Systems of Equations)
Systems of Quadratic Equations
[November SAT Math] Systems Of Equations - TRICKS & SHORTCUTS + Matching Rule (Score Higher)
Method of Elimination Steps to Solve Simultaneous Equations
Solving Systems of Equations... Substitution Method (NancyPi)
Solve a system of three variables
Gaussian Elimination & Row Echelon Form
Introduction to Linear Algebra: Systems of Linear Equations
Linear Algebra - Solving Systems of Equations
Комментарии
 0:10:27
0:10:27
 0:04:34
0:04:34
 0:05:15
0:05:15
 0:04:49
0:04:49
 0:12:44
0:12:44
 0:07:18
0:07:18
 0:03:47
0:03:47
 0:12:44
0:12:44
 0:00:49
0:00:49
 0:05:17
0:05:17
 0:02:54
0:02:54
 0:10:21
0:10:21
 0:19:44
0:19:44
 0:05:03
0:05:03
 0:07:30
0:07:30
 0:28:36
0:28:36
 0:11:20
0:11:20
![[November SAT Math]](https://i.ytimg.com/vi/yYeIFsby4tQ/hqdefault.jpg) 0:15:06
0:15:06
 0:02:58
0:02:58
 0:18:30
0:18:30
 0:12:45
0:12:45
 0:18:40
0:18:40
 0:10:45
0:10:45
 0:05:59
0:05:59