filmov
tv
Math Olympiad | A Nice Algebra Problem | Integer Solutions...
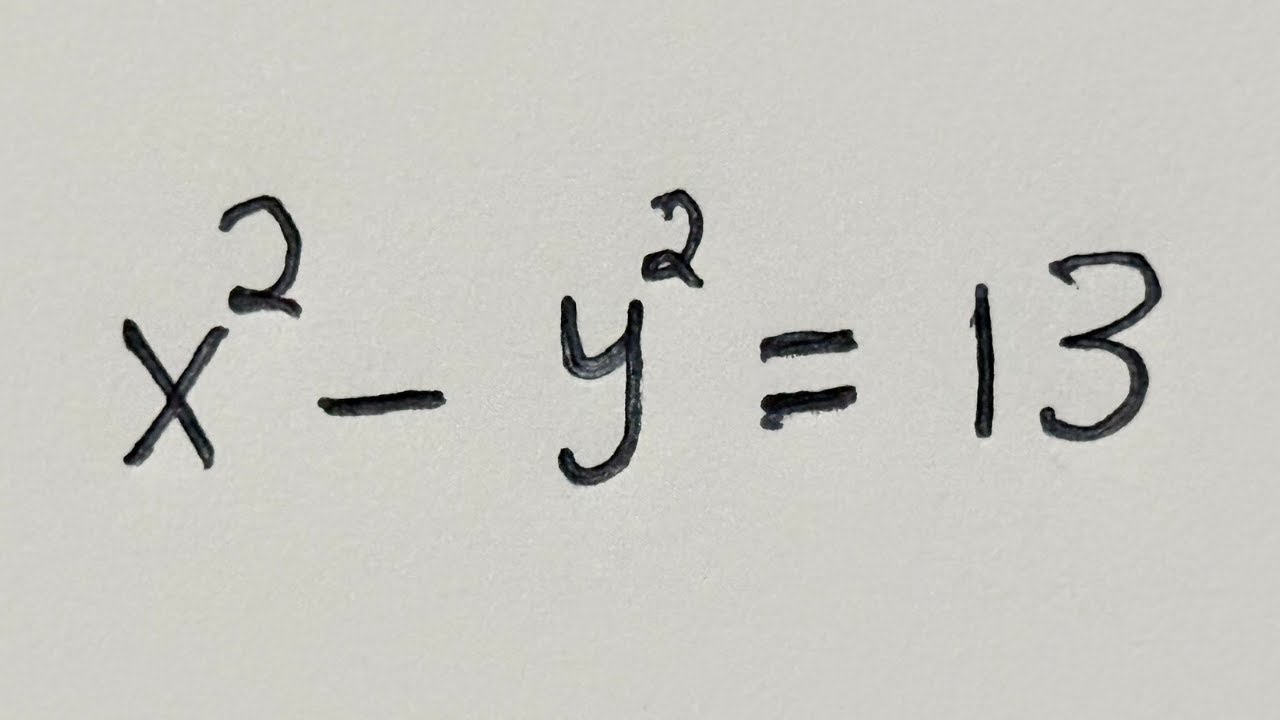
Показать описание
This equation represents a classic problem of solving for two variables in mathematics. Known as the difference of squares, this equation frequently appears in math Olympiads, challenging students to use factorization and number theory to find integer solutions for and .
#MathOlympiad #NumberTheory #DifferenceOfSquares #Algebra #MathChallenge #STEM #ProblemSolving
#MathOlympiad #NumberTheory #DifferenceOfSquares #Algebra #MathChallenge #STEM #ProblemSolving
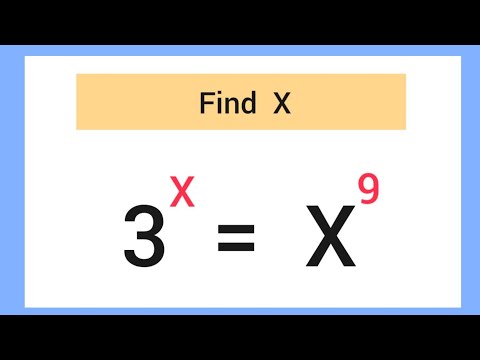
A Nice Math Olympiad Exponential Equation 3^x = X^9
A beautiful international math olympiad problem
Thailand | Math Olympiad Question | Nice Algebra Equation
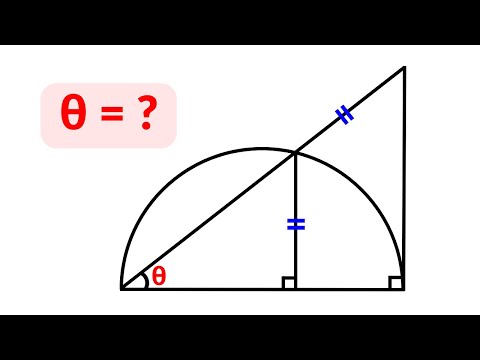
Japanese Math Olympiad | A Very Nice Geometry Problem
China | Math Olympiad | A Nice Algebra Problem 👇
Nice Olympiad Math | x^2–x^3=12 | Nice Math Olympiad Solution
A Nice Olympiad Exponential Multiplication Problem #short #olympiad #mathematics #maths #exponents
Mexico - A Nice Math Olympiad Exponential Problem
Nice Maths Olympiad | Find value of X | Can You Solve This ?
Français | Can you solve this ? | A nice Math Olympiad Exponential Simplification | Algebra Problem
Spainish | A Nice Algebra Problem | Math Olympiad
China | Math Olympiad | A Nice Algebra Problem 👇👇👇
Math Olympiad Question | Nice Algebra Equation | You should know this trick!!
Luxembourg - Math Olympiad Question | You should know this trick
Vietnam | A Nice Algebra Problem | Math Olympiad
Romania Math Olympiad | A Very Nice Geometry Problem
Math Olympiad | A Nice Algebra Problem | A Nice Radical Problem
A Nice Algebra Math Problem. Expansion Problem. #shorts #math #algebra #olympiad #expansion #viral
Japanese| A nice math Olympiad question #maths #matheolympiad
Norway Math Olympiad Question | You should be able to solve this!
Germany |A Nice Math Olympiad Problem👇
Japanese Math Olympiad Problem | A Nice Math Problem : Comparison
Portugal | A Nice Algebra Problem | Math Olympiad👇
Canada | A Nice Algebra Problem | Math Olympiad
Комментарии
 0:02:34
0:02:34
 0:01:00
0:01:00
 0:01:26
0:01:26
 0:09:58
0:09:58
 0:10:37
0:10:37
 0:15:05
0:15:05
 0:00:52
0:00:52
 0:08:36
0:08:36
 0:04:10
0:04:10
 0:10:49
0:10:49
 0:10:04
0:10:04
 0:15:05
0:15:05
 0:07:26
0:07:26
 0:02:51
0:02:51
 0:13:53
0:13:53
 0:10:52
0:10:52
 0:19:29
0:19:29
 0:00:59
0:00:59
 0:09:55
0:09:55
 0:03:21
0:03:21
 0:09:14
0:09:14
 0:04:15
0:04:15
 0:15:18
0:15:18
 0:12:49
0:12:49