filmov
tv
A beautiful math question for advanced students
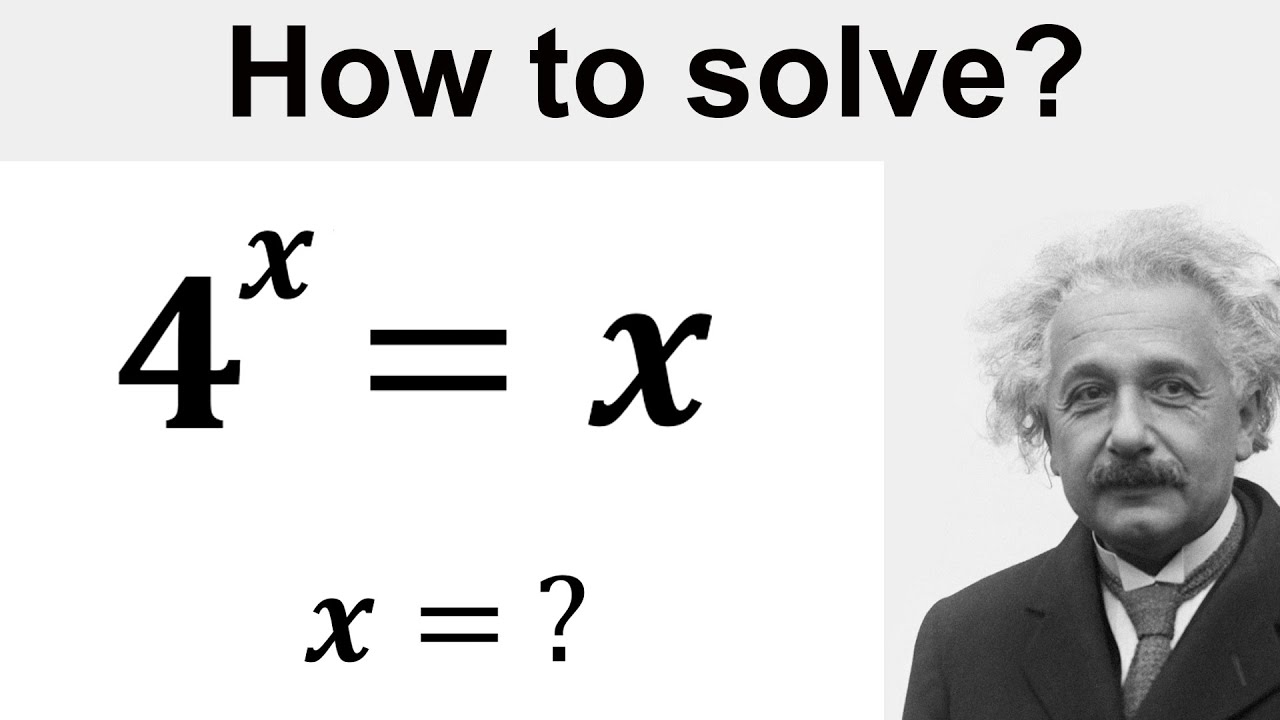
Показать описание
What do you think about this problem? If you're reading this ❤️.
Hello My Friend ! Welcome to my channel. I really appreciate it!
@higher_mathematics
#maths #math
Hello My Friend ! Welcome to my channel. I really appreciate it!
@higher_mathematics
#maths #math
A beautiful math question for advanced Students
A beautiful math question for advanced Students
A beautiful math question for advanced Students
A beautiful math question for advanced Students
A beautiful math question for advanced Students
A beautiful math question for advanced Students
A Beautiful Math Question for School Students | Can You Get This Math Question Correct?
A Beautiful Math Question for Advance Students | Only 12% Students Got This Math Question Correct
The Most Beautiful Equation in Math
The Beauty of Math - Zimmer [Motivational]
Anyone Can Be a Math Person Once They Know the Best Learning Techniques | Po-Shen Loh | Big Think
Luxembourg - Math Olympiad Question | You should know this trick
The Simplest Math Problem No One Can Solve - Collatz Conjecture
One Man's Mission to Unveil Math's Beauty
The Hardest Math Test
This Video Will Make You Better At Math
How to become a Math Genius.✔️ How do genius people See a math problem! by mathOgenius
The EASIEST SAT Math Question!
You're a physicist, so you're good at math, right? #Shorts
Math Olympiad Question | You should know this trick!!
The 7 Levels of Math
MIT Entrance Exam Problem from 1869 #Shorts #math #maths #mathematics #problem #MIT
Math trick for multiplying?
Mexico - A Nice Math Olympiad Exponential Problem
Комментарии
 0:10:35
0:10:35
 0:09:25
0:09:25
 0:10:26
0:10:26
 0:10:06
0:10:06
 0:09:24
0:09:24
 0:08:08
0:08:08
 0:04:27
0:04:27
 0:06:35
0:06:35
 0:03:50
0:03:50
 0:06:38
0:06:38
 0:03:53
0:03:53
 0:02:51
0:02:51
 0:22:09
0:22:09
 0:07:10
0:07:10
 0:00:28
0:00:28
 0:04:52
0:04:52
 0:15:24
0:15:24
 0:00:26
0:00:26
 0:00:09
0:00:09
 0:00:33
0:00:33
 0:08:44
0:08:44
 0:00:48
0:00:48
 0:00:16
0:00:16
 0:08:36
0:08:36