filmov
tv
This Video Will Make You Better At Math
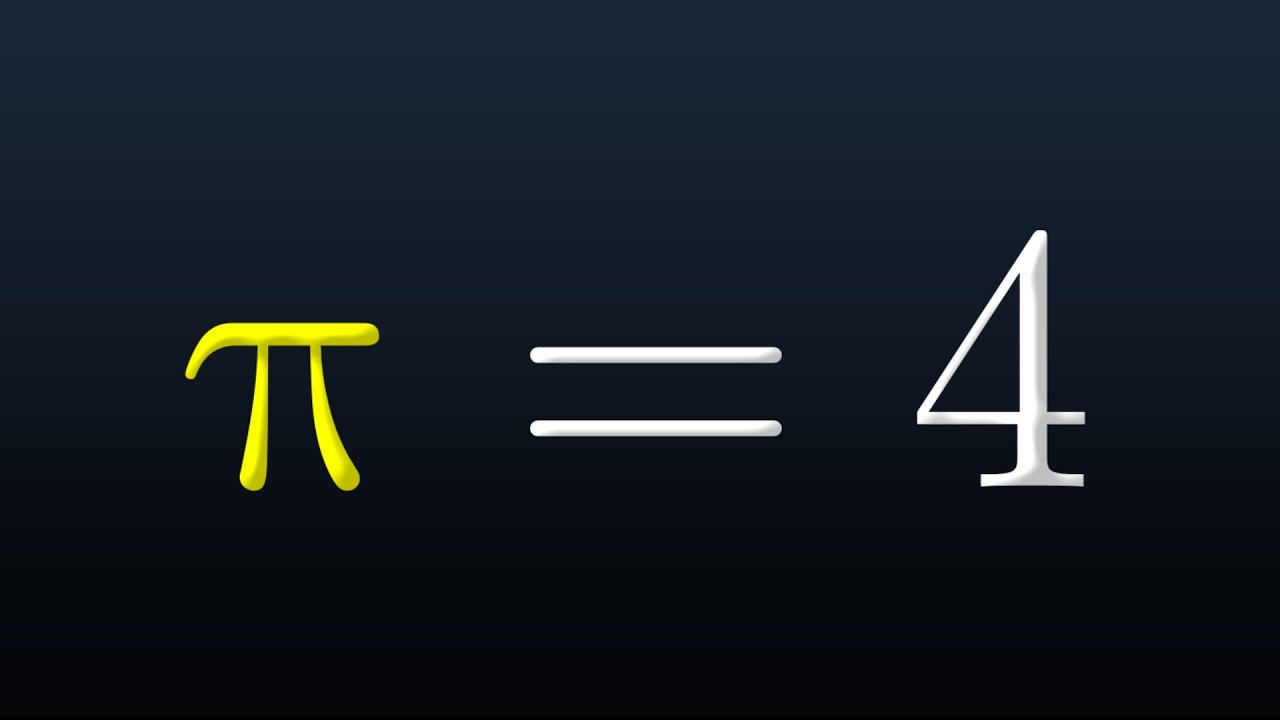
Показать описание
🎓Become a Math Master With My Intro To Proofs Course!
This is a (fairly) famous mathematical fallacy. Can you figure out what's going wrong? Does Pi really equal 4?
🛜 Connect with me on my Website
🙏Support me by becoming a channel member!
Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.
Pi=4
#math #brithemathguy #pi
This is a (fairly) famous mathematical fallacy. Can you figure out what's going wrong? Does Pi really equal 4?
🛜 Connect with me on my Website
🙏Support me by becoming a channel member!
Disclaimer: This video is for entertainment purposes only and should not be considered academic. Though all information is provided in good faith, no warranty of any kind, expressed or implied, is made with regards to the accuracy, validity, reliability, consistency, adequacy, or completeness of this information.
Pi=4
#math #brithemathguy #pi
This Video will Make You Pee in 5 Seconds! (100%) 😳
This video will make you see a Pikachu on your hands!🤯(REAL)
This Video will Make You Small for 5 Seconds!! 😱 (99% Real)
This Video will Make You Poop in 5 Seconds! 😳
This Video will Make You See Everything in Green Color! 😱🟢
This Video will Make You Pee In 5 Seconds!! 😱 (100% Real)
This Video will Magically Make You Fall...(REAL) 😱
This video will make you Angry!!
This Video Will Make You A Lockdown Cornerback!!
This Video will Make You See MARIO In Your Room!
This Video Will Make You Forget Your age! 🤯 (3 Seconds)
This Video will Make You Feel Like You are Flying! 🤯
This Video will Make Things Invisible...😱
This video will make you Blind for 5 seconds!!
This video will make you Fly (REAL)
This Video will Make You See SONIC In Your Room!
This Video will Magically Make Your Fingers Grow! 😱
This Video will Make You See PEACHES In Your Room!
this video will make you want to poop...
This Video will Make You Poop In 5 Seconds!! 😱 (100% Real)
I Will Make You Sleep In 10 Seconds...😴(100%Real)
This Video will Make You CRY! 😭 (100%)
This Video will Make Everything Flying Around You! 😱
This Video will Make Your Hands Colorful! 😱 (Real)
Комментарии
 0:00:53
0:00:53
 0:01:08
0:01:08
 0:00:50
0:00:50
 0:00:56
0:00:56
 0:01:31
0:01:31
 0:00:56
0:00:56
 0:01:02
0:01:02
 0:01:33
0:01:33
 0:13:06
0:13:06
 0:00:48
0:00:48
 0:01:17
0:01:17
 0:00:58
0:00:58
 0:01:25
0:01:25
 0:01:03
0:01:03
 0:00:55
0:00:55
 0:00:50
0:00:50
 0:01:01
0:01:01
 0:01:01
0:01:01
 0:00:42
0:00:42
 0:00:57
0:00:57
 0:00:58
0:00:58
 0:04:01
0:04:01
 0:01:10
0:01:10
 0:00:51
0:00:51