filmov
tv
Integrating Powers of tanx | Trigonometry
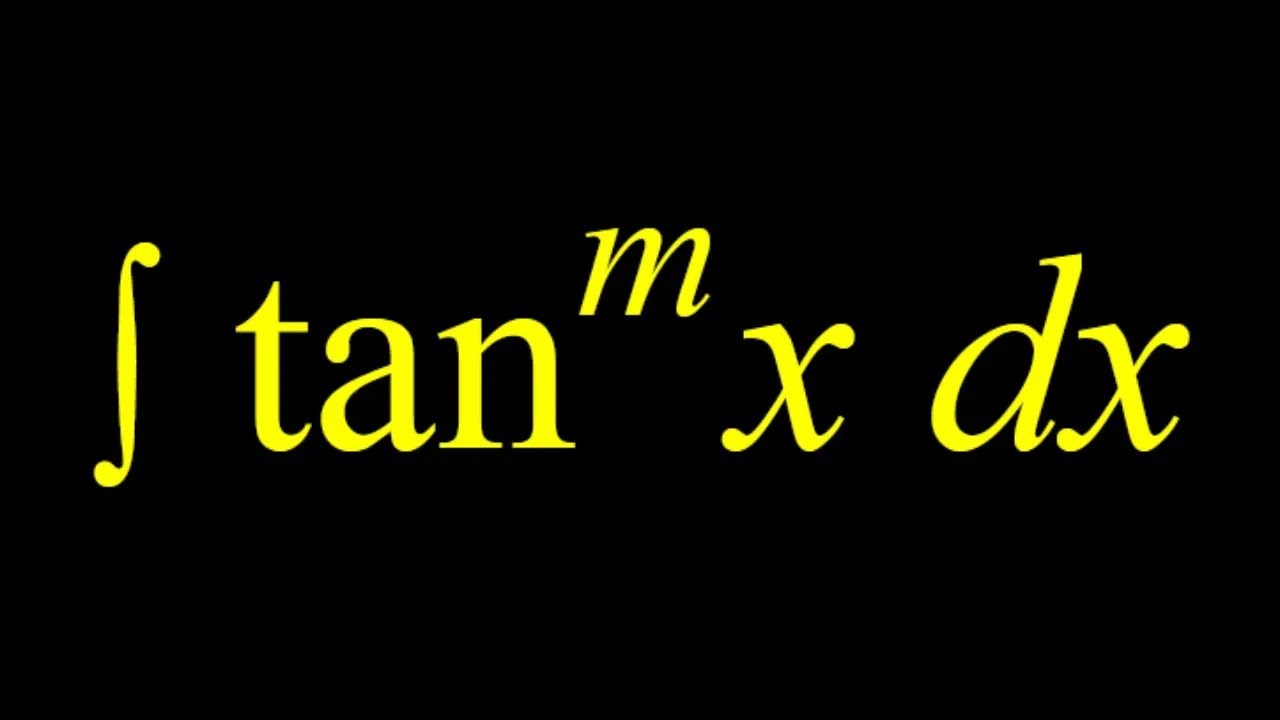
Показать описание
🤩 Hello everyone, I'm very excited to bring you a new channel (SyberMath Shorts).
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
x^3+2x^2=3
#ChallengingMathProblems #PolynomialEquations #Polynomials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Enjoy...and thank you for your support!!! 🧡🥰🎉🥳🧡
If you need to post a picture of your solution or idea:
x^3+2x^2=3
#ChallengingMathProblems #PolynomialEquations #Polynomials
via @YouTube @Apple @Desmos @NotabilityApp @googledocs @canva
PLAYLISTS 🎵 :
Integrating powers of tan x
Integrating powers of tan(x)
Integrating Powers of tanx | Trigonometry
Integrating Powers of tan(x) and sec(x) with an Even Power of sec(x)
STANDARD Integration of powers of tanx
trig integrals involving secant and tangent (calculus 2)
Trigonometric Integrals: Powers of Secant and Tangent
Integrating Powers of Tangent
Integral of tan^5(x)
Ma 126 Integrating powers of the tangent function.
Integral of tan^4x
Trig Integrals (Integrating Powers of Tangent)
Trigonometric Integrals Powers of Secant and Tangent tan^4(x)
Powers of tan(x) and sec(x)
Section 3.2 (Integrating Products of Powers of tan x and sec x)
How to Integrate Odd & Even Powers of Sine & Cosine : Math Problems & Trigonometry
Integration : - ( Integral of even powers of Tanx and Cotx ) - 23.
8.3.4 Integrating Products of Powers of Secant and Tangent
Integrating Powers of Trigonometric Functions--Power of the Tangent is Odd and Positive
Trig Integrals Involving Even Powers of Tan and no Factors of Sec
INTEGRALS OF POWERS OF TANGENTS AND SECANTS: CASE 1
Integrating Even Powers of sin(x) and cos(x)
Integrating the Products of the Powers of Secant and Tangent | Odd and Even Powers
integral of tan^2(x)*sec(x), calculus 2 tutorial
Комментарии
 0:03:28
0:03:28
 0:13:16
0:13:16
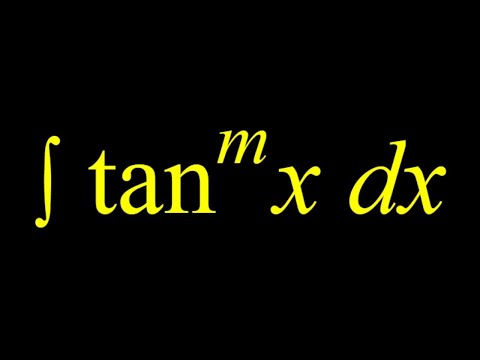 0:13:16
0:13:16
 0:03:27
0:03:27
 0:14:59
0:14:59
 0:16:51
0:16:51
 0:08:32
0:08:32
 0:06:36
0:06:36
 0:06:43
0:06:43
 0:04:32
0:04:32
 0:04:09
0:04:09
 0:07:43
0:07:43
 0:03:21
0:03:21
 0:09:28
0:09:28
 0:09:53
0:09:53
 0:08:06
0:08:06
 0:13:15
0:13:15
 0:19:08
0:19:08
 0:05:24
0:05:24
 0:05:33
0:05:33
 0:14:03
0:14:03
 0:04:28
0:04:28
 0:07:12
0:07:12
 0:04:10
0:04:10