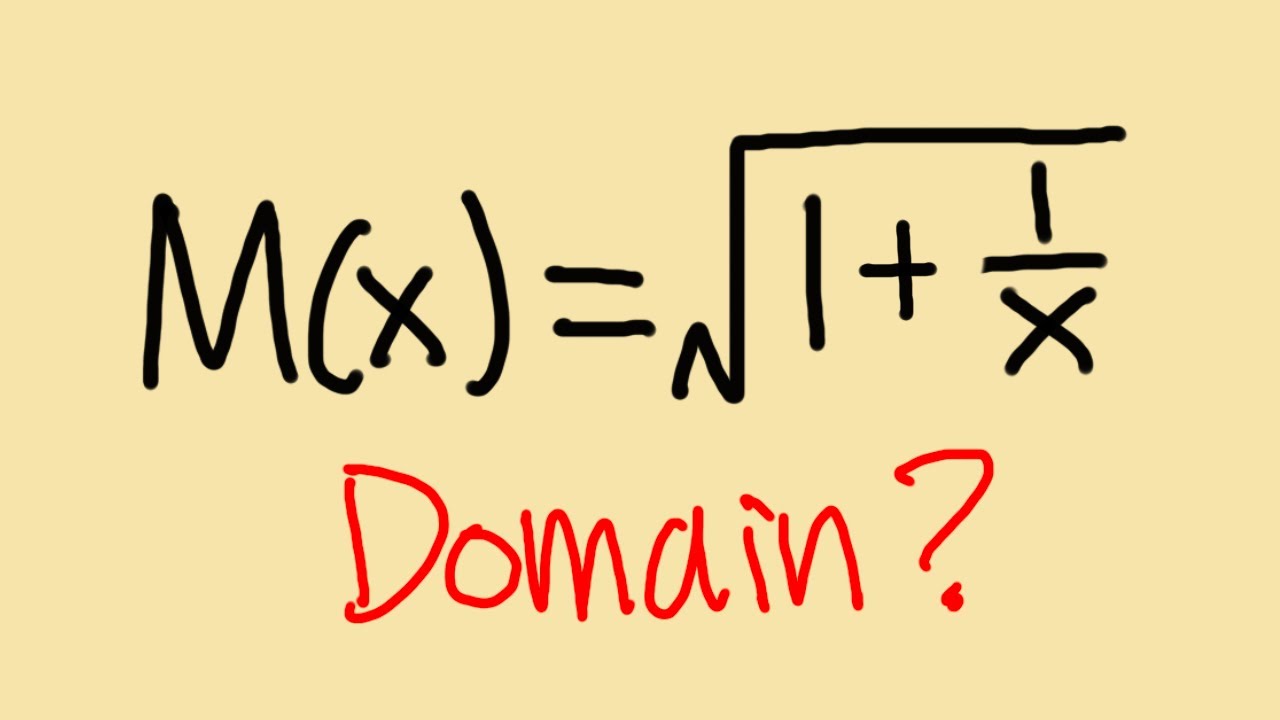
How to find the domain of M(x)=sqrt(1+1/x)

This question is from Single Variable Calculus by James Stewart. Although it's from a calculus textbook, we just have to do algebra and precalculus for this. We have to find the domain of M(x)=sqrt(1+1/x) and how do we set up the restrictions? Subscribe to @bprpmathbasics for more math tutorials!

-----------------------------
-----------------------------
#math #algebra #mathbasics

[justaspeedcuber]

i dont even understand what this is about but i enjoy your videos, keep up the good work sir

[jskelton]

At about 1:50, just times both sides by x^2 (guaranteed positive) to get x(x+1)>=0 hence (by considering graph) x<=-1 or x>=0
Then make the second inequality strict: x>0 (since we know x=/=0). Done in moments.

[o_pao.z]

interesting the way you solved the inequality, I learned to analyze the sign of the equation of the numerator and the denominator using a line for each, and then make the 'product table' of the intervals

[m.h.]

The domain depends on the number type that you look for. If you look for complex numbers or more, you only have x ≠ 0.
For real and easier number types you also have:
1 + 1/x ≥ 0 |-1
1/x ≥ -1 |*x
Case x > 0: (x ≠ 0 is already given)
1 ≥ -x |*-1
-1 ≤ x
But x > 0 is already more restrictive, So x > 0 is a valid range
Case x < 0:
1 ≤ -x |*-1
-1 ≥ x
So x ≤ -1 is a valid range

So - for real numbers or simpler - we have a domain of (-∞, -1] and (0, ∞)

[ForStudying-wq]

hey as this is a math channel can u help me with something. Can u tell me all the different names of the three main nature of roots like bsquared -4ac>0 and b^2-4ac<0 and bsquared-4ac=0. Sometimes they are called real and distinct, sometimes real and equal, sometimes complex, not real, repeating, tangent to the curve, intersecting the curve and other stuff. can u pls tell me all names and explain which one each is. plsss

[Negan_]

Hello, why have you taken open interval at ∞ although 1+ 1/∞=1

[okaro]

Wouldn't it be simpler to just check where the nominator and denominator have the same sign. We gdt

x>0 and x+1>=0 i.e. x>0, or x<0 and x+1<0 i.e. X<=-1

[elmeralvarado]

Why is the (x+1)=0. X=-1 "important" I've only been taught to evaluate the bottom x can not equal zero

[cyrusyeung]

Why not solve 1 + 1/x ≥ 0 by:
1 + 1/x ≥ 0
1/x ≥ -1
-1/x ≤ 1
(x ≥ -1 and x > 0) or (x ≤ -1 and x < 0)
(x > 0) or (x ≤ -1)

[-wx--]

How it happened that lovely fish ∝ eventually become “inside”?! 😉