The SAT Question Everyone Got WRONG - An Alternative Proof

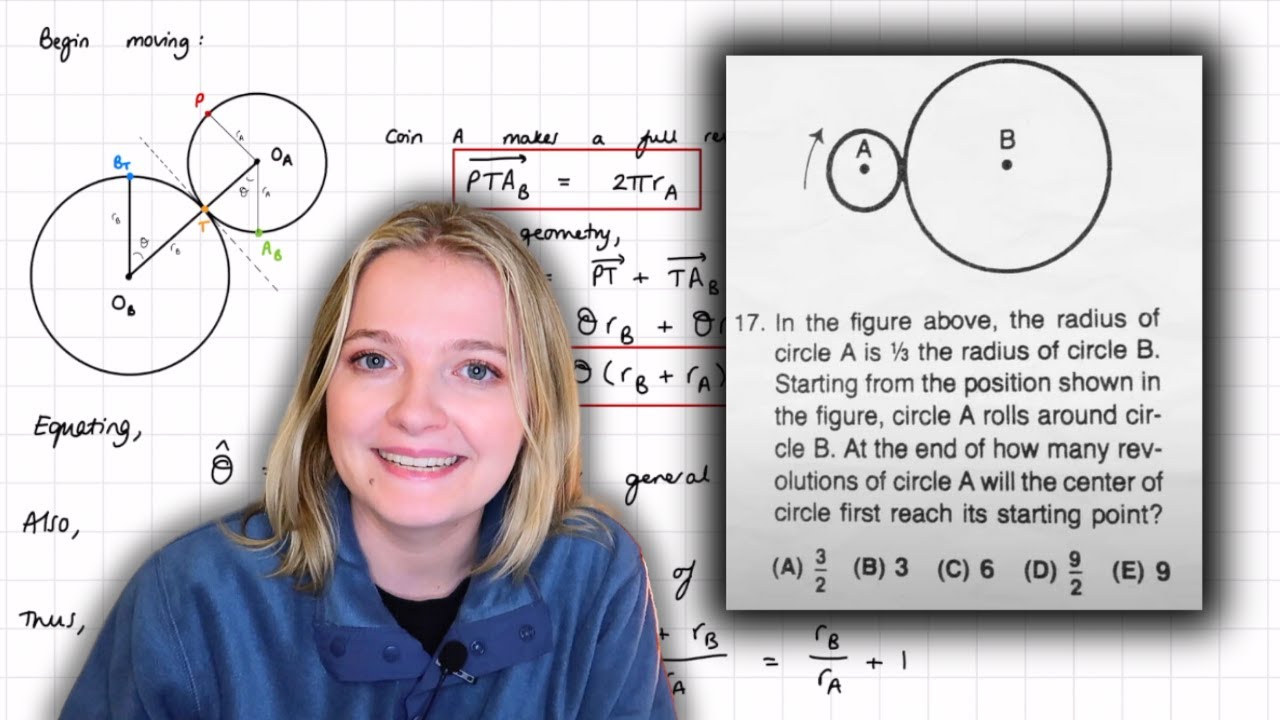
Let's look at the viral SAT problem that everyone got wrong! Showing you the basics of the problem as well as an alternative proof!

🔗 Social Media 🔗

My CV & Personal Statement (+ other documents):

✨Support my channel✨

For those of you that are new here, hi there 🌞 my name is Ellie and I'm a Part III Mathematics Graduate from the University of Cambridge and current Astrodynamics Software Engineer! This channel is where I nerd out about maths, physics, space and coding so if that sounds like something you're interested in, click the subscribe button to follow along ☺️

This video was sponsored by Brilliant 🚀

[maisonbishop]

As someone who just graduated with a math degree, I felt like an idiot for not getting this right at first😂

[superball]

great question! Thanks for covering.
My initial intuition was: 3 would be how many rotations we get if we spin the big coin under the small coin once. But relative to each other, we're seeing an additional rotation of the big coin in opposite direction.
great to see how geometry touches into algebraic topology

[mharouny]

Brilliant explanation! As an extension to what you were saying, you can say that you have to take a larger "step" to meet each point on the circle than you would need to meet on the flat line.

[DownhillAllTheWay]

The example with the triangle makes it immediately intuitive, but like most people, I got it wrong in the first instance - however, when I say "like most people", that includes the examiner who set the question, who also got it wrong - and we know this because the correct answer did not figure among the multi-choice answers.

[tomasgarza]

Circle A is subject to both a rotation motion (about its center) and a translation motion (around the circumference of Circle B). In order to prevent slippage, the rotation motion must conveniently adjust its speed to the translation speed, depending on the relative sizes of the two circles. Now, suppose we stop the rotation and let Circle A simply glide around Circle B., glueing, as it were, the point of tangency. This means that the relative positions of the point of tangency and the center of Circle A change in such a way that, at the end of the process, they are back at its original state. Thus, Circle A will have given one whole turn, due only to the translation motion. This, I hope, explains why there is one more turn in the end. The rotation motion contributes whatever number of revolutions result from the ratio between the radii of the circles, and the translation motion contributes one additional turn.

[armanavagyan]

PROF i pray God every day that THIS CHANNEL continue to exist😘

[MATHSNISHANTVERMA]

@EllieSleightholm which site or app are you using for the platform where you are solving it 8:41

[zarraz]

in the last demonstration, something comes out of the blue: line between Oa center and green point Ab. It helps for alternate angles calculation, but what is its justification, construction and angle value (parallelism and same theta angle?) ?

[jim]

Number of small coin rotations around large coin. Formula: (R + r)/r, where R = large coin radius, r = small coin radius.
For example: R = 3 and r = 1. (3 + 1)/1 = 4. Muy facil.

[lousleightholm]

Great question & answer! I was lost initially but you explain everything so well! Thank you

[elyaelovenom]

the art of mathematics
ellie I appreciate this channel and tricking math questions that u post here
10Q a lot

[VarunAstro]

Wow you actually full-filled my wish ❤❤

[Melatmeshesha-erfb]

Wow you motivated me to study maths lovely thanks

[IsaacNewton-edoj]

If the inner circle was a dot then then the number of revolutions would be 1 .
And if the outer circle was a dot then it would be rb/ra (just removing the +1 from your formula as the radius of the smaller circle would become 0)

[Jms]

How we would solve it if the center of the smaller circle would be at the top?

[marshian__mallow]

Would you be able to make a video talking about your job position more? I can’t find much information about what exactly an Astrodynamics software engineer does and how much it pays. Also to get a job like that would you have to specialise in applied maths as opposed to pure maths?

[jimf]

I like ur proof. Very smart. I think you may have slipped up near the end. At 15 minutes and 40 seconds where you say 2 pi = N x theta hat. If theta hat = 4 pi, then doesn’t N = 1/2? I’d think 2 pi N = theta hat. I’ve made some videos and it’s extremely hard to get everything right in one take. It gave me newfound respect for politicians who have to answer questions on the fly. My respect for them went from zero to epsilon. 😂 Anyway, good luck with your channel.❤🌈🤓💃🥳🥁

[isobar]

Clear as mud for me! Still can't explain it when you substitute the circles for gears that can't slip. i'm so confused...oh well, go to the back of the class!

[DalyHenry-nq]

I think that's sure, best intervalle geometric🤝

[virais]

You are brilliant! Your resolutions are incredible, I found your channel by chance and I'm grateful to God for that, I barely started exploring the enormous amount of content you have available on the platform, but I'm already impressed with the amount of skills you bring together. So is this what Cambridge graduates are capable of doing?