filmov
tv
Uncountability of the reals using their bijective correspondence with the subsets of natural numbers
Показать описание
In this video, using the famous Schroder-Bernstein Theorem, we show that there exists a bijective map from the power set of the set of natural numbers onto the set of real numbers. In the process we derive an alternative proof for the uncountability of the reals.
0:04:02
S01.9 Proof That a Set of Real Numbers is Uncountable
0:06:54
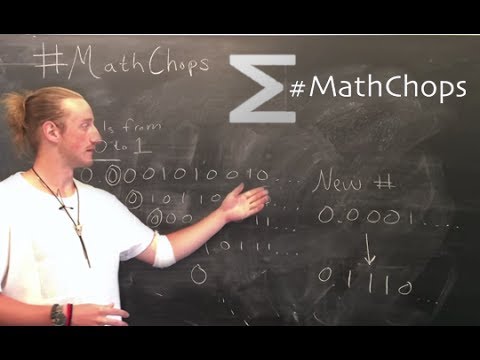
Episode 4: Uncountability of Real Numbers [#MathChops]
0:10:13
Real Analysis: Uncountability of reals using Nested Interval Theorem
0:08:20
The Set Of Real Numbers Is Uncountable
0:08:18
Real Analysis | The uncountability of ℝ
0:01:36
The Real Numbers. Uncountable.
0:07:20
An Alternative Proof That The Real Numbers Are Uncountable
0:04:34
The Real Numbers are Uncountable
0:10:04
The set of real numbers is uncountable (Proof) [ILIEKMATHPHYSICS]
0:50:11
MATH52: Lecture 30 - Uncountability of the Reals
0:04:47
6.3 Uncountability of the real numbers
0:05:24
Real Analysis Course #12 - (0,1) is Uncountable Using Diagonalization (Cantor Diagonalization)
0:27:46
Real Analysis-16: Set of Real Numbers is Uncountable (Via Nested Interval Property)
0:12:23
The Real Numbers are not listable/countable (Cantor's Diagonalisation Argument)
0:02:37
Real Numbers are Countable
0:00:57
prove that set r of real numbers is uncountable
0:12:04
Cantor's proof that the real numbers are uncountable
0:04:44
Set of Real Numbers is Uncountable Proof (by Cantor's Diagonal Argument)
1:02:00
Andrej Bauer: 'The countable reals'
0:06:59
Set of Real numbers is Uncountable | Cantor's diagonal argument | Discrete Mathematics
0:10:22
Uncountability of the reals using their bijective correspondence with the subsets of natural numbers
0:18:48
Reals are Uncountable
0:09:28
Reals are uncountable
0:07:27
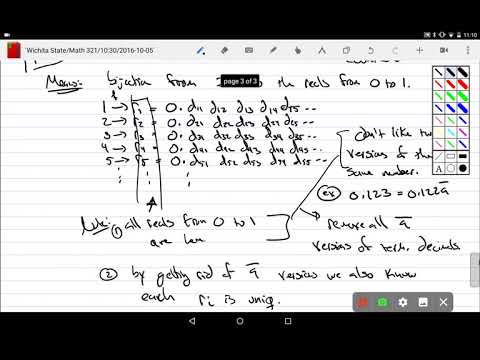
Countability: The Real Numbers are Uncountable - Part 1
Комментарии