filmov
tv
Calculate Area of the Blue shaded Circle | Learn these simple Geometry Tools fast | Math Olympiad
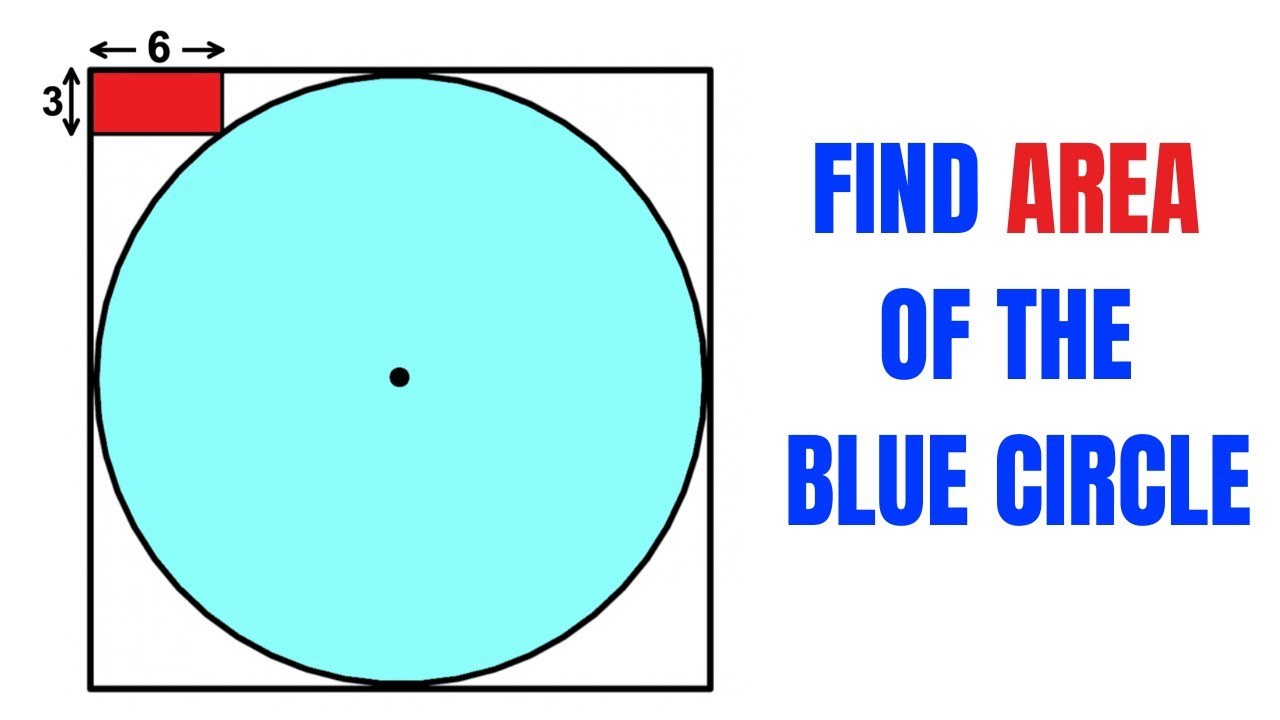
Показать описание
Need help with finding the area of a Semicircle? You're in the right place!
I have over 20 years of experience teaching Mathematics at American schools, colleges, and universities. Learn more about me at
Calculate Area of the Blue shaded Circle | Learn these simple Geometry Tools fast | Math Olympiad
#AreaOfCircle #FindAreaOfBlueShadedCircle #GeometryMath #OlympiadPreparation #Area
#CollegeEntranceExam #OlympiadMathematics #PythagoreanTheorem
#FindAreaOftheshadedSemicircle #HowtofindtheAreaof Semicircle #PythagoreanTheorem #ASA #AreaOfCircle #AreaofTheCircle #RightTriangle #TrigonometricRatios #Radius #Square#IsoscelesTriangle #IsoscelesTriangles #Rectangle #Circle #Radius
#blackpenredpen #OlympiadMathematics
How to Find the Area of a Circle
How to Find the Area of a Semicircle
How to Find the Area of a SemiCircle Step by Step
How to solve the hard geometry problems
How to find area of shaded region
blackpenredpen
Olympiad Mathematics
pre math
Po Shen Loh
Find area of the Blue shaded Circle
imo
Competitive exams
college entrance exam
Pythagorean Theorem
Subscribe Now as the ultimate shots of Math doses are on their way to fill your minds with the knowledge and wisdom once again.
Комментарии
 0:06:10
0:06:10
 0:08:36
0:08:36
 0:05:39
0:05:39
 0:09:17
0:09:17
 0:11:58
0:11:58
 0:01:54
0:01:54
 0:08:08
0:08:08
 0:05:44
0:05:44
 0:06:24
0:06:24
 0:03:00
0:03:00
 0:08:31
0:08:31
 0:04:48
0:04:48
 0:04:57
0:04:57
 0:08:06
0:08:06
 0:06:45
0:06:45
 0:02:38
0:02:38
 0:08:14
0:08:14
 0:04:03
0:04:03
 0:08:19
0:08:19
 0:11:11
0:11:11
 0:05:38
0:05:38
 0:15:51
0:15:51
 0:00:57
0:00:57
 0:07:56
0:07:56