filmov
tv
MCR3U - Simplifying Rational Expressions Part 1 - Grade 11 Functions

Показать описание
Course Site - Grade 11 Functions (MCR3U)
Other High School Courses
MHF4U Grade 12 Advanced Functions (Academic)
MCV4U Grade 12 Calculus & Vectors (Academic)
Social Media
key words: FIN300, FIN 300, FIN401, FIN 401, QMS 102, QMS 101, QMS10, ADMS 3530, ADMS3530, ADMS 4501, ADMS 4502, RYERSON UNIVERSITY, YORK UNIVERSITY, QUEENS UNIVERSITY, COMM 121, COMM121, COMM122, COMM 122, MAT133, MAT 133, MCV4U, MHF4U, MPM2D, MPM1D, MAT 134, MAT 135, calculus and linear algebra, MISSISSAUGA, TORONTO, calculus, advanced functions, grade 12, grade 11, high school, COMM 298, UBC, ACC 100, Ryerson, AMF 102, Waterloo university, STATS 1024, CALC 1000, Western University
#mcr3u #functions #grade11
Other High School Courses
MHF4U Grade 12 Advanced Functions (Academic)
MCV4U Grade 12 Calculus & Vectors (Academic)
Social Media
key words: FIN300, FIN 300, FIN401, FIN 401, QMS 102, QMS 101, QMS10, ADMS 3530, ADMS3530, ADMS 4501, ADMS 4502, RYERSON UNIVERSITY, YORK UNIVERSITY, QUEENS UNIVERSITY, COMM 121, COMM121, COMM122, COMM 122, MAT133, MAT 133, MCV4U, MHF4U, MPM2D, MPM1D, MAT 134, MAT 135, calculus and linear algebra, MISSISSAUGA, TORONTO, calculus, advanced functions, grade 12, grade 11, high school, COMM 298, UBC, ACC 100, Ryerson, AMF 102, Waterloo university, STATS 1024, CALC 1000, Western University
#mcr3u #functions #grade11
MCR3U - Simplifying Rational Expressions Part 1 - Grade 11 Functions
Simplifying Rational Expressions (MCR3U)
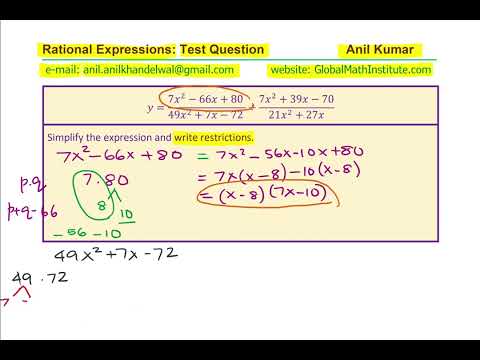
MCR3U Simplifying Rational Expressions - Test Solutions
MCR3U - Simplifying Rational Expressions Part 2 - Grade 11 Functions
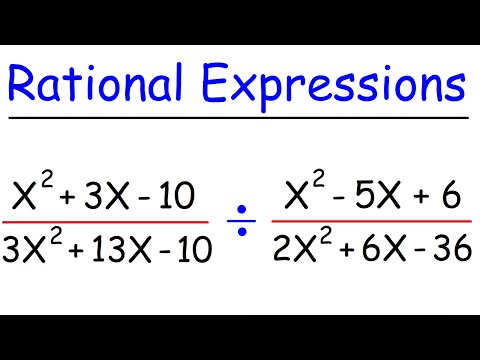
MCR3U 2.1/2.2 - Simplifying Rational Expression
Simplify Rational Expressions by Factoring
Simplify Rational Expressions
MCR3U - Simplifying Rational Expressions with Restrictions Part 3 - Grade 11 Functions
Simplifying Rational Expressions (MCR3U)
Rational Expressions - Basic Introduction
Simplify Rational Expression
Simplifying Rational Expressions
Mix of Operations with Simplifying Rational Expressions
Simplifying Rational Expressions
Simplify Rational Expressions and Write Restrictions Complete Review From Simple to Complex Cases
Simplify Rational Expressions With Division and Write Restrictions MCR3U
Simplifying Rational expressions
MCR3U - Simplifying Rational Expressions - Part 1 of 3
Simplifying Rational Expressions
Simplify Rational Expressions
Simplify and State Restrictions of Rational Expressions
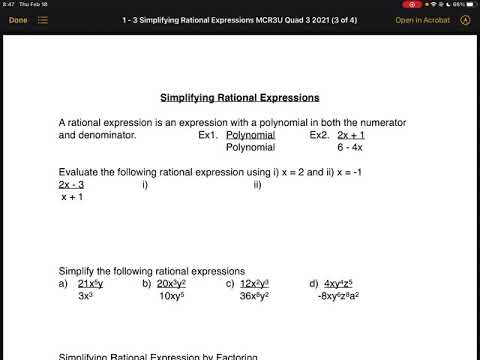
1-3 simplifying Rational expressions MCR3U
Simplifying Rational Expressions
Multiplying and Dividing Rational Expressions (Simplifying with Restrictions)
Комментарии
 0:07:54
0:07:54
 0:25:14
0:25:14
 0:29:27
0:29:27
 0:09:18
0:09:18
 0:19:32
0:19:32
 0:04:18
0:04:18
 0:03:28
0:03:28
 0:08:55
0:08:55
 0:24:28
0:24:28
 0:12:46
0:12:46
 0:03:18
0:03:18
 0:03:28
0:03:28
 0:09:26
0:09:26
 0:05:02
0:05:02
 0:19:36
0:19:36
 0:15:07
0:15:07
 0:03:29
0:03:29
 0:14:00
0:14:00
 0:22:19
0:22:19
 0:14:38
0:14:38
 0:04:31
0:04:31
 0:10:16
0:10:16
 0:06:13
0:06:13
 0:05:51
0:05:51