filmov
tv
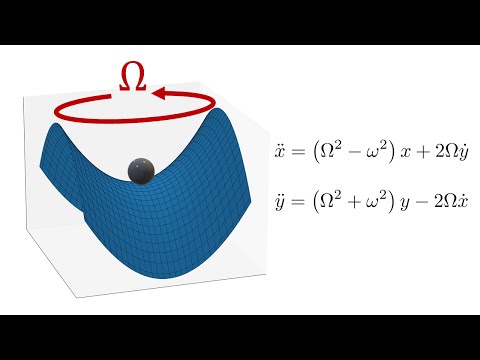
Particle dynamics on a rotating saddle

Показать описание
Particle dynamics on the surface of a rotating saddle simulated with high order symplectic integrators. The system is treated as a Hamiltonian system with a potential x^2 - y^2 rotated around the center. The Hamiltonian is non-autonomous in the Cartesian form because the rotation depends on explicitly on time. The Hamiltonian is generally not constant.
High rotation speed traps the particles with various motions around the center, whereas the particles may consistently roll down or up the surface if the rotation speed is too low.
The saddle potential, in spite of its simplicity, has numerous applications related to particle traps and mass filters albeit with the surface being associated with field potentials rather than height. The saddle shape potential field in particular can arise simply from the presence of four charged rods.
0:00 non-rotating saddle (particles not trapped)
0:07 very slow rotation (particles not trapped)
0:16 rapid rotation
0:22 moderate rotation
0:48 moderate rotation
0:56 slow rotation
1:04 phase space (q1,p2) of slow rotation
High rotation speed traps the particles with various motions around the center, whereas the particles may consistently roll down or up the surface if the rotation speed is too low.
The saddle potential, in spite of its simplicity, has numerous applications related to particle traps and mass filters albeit with the surface being associated with field potentials rather than height. The saddle shape potential field in particular can arise simply from the presence of four charged rods.
0:00 non-rotating saddle (particles not trapped)
0:07 very slow rotation (particles not trapped)
0:16 rapid rotation
0:22 moderate rotation
0:48 moderate rotation
0:56 slow rotation
1:04 phase space (q1,p2) of slow rotation
Moment of Inertia and Angular velocity Demonstration #physics
Rigid Bodies Relative Motion Analysis: Velocity Dynamics (Learn to solve any question step by step)
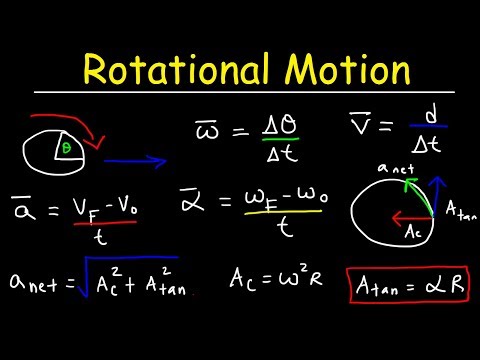
Rotational Motion Physics, Basic Introduction, Angular Velocity & Tangential Acceleration
Particle on a rotating saddle
Particle dynamics on a rotating saddle
Angular Motion and Torque
[2015] Dynamics 28: Relative Motion Analysis Using Rotating Axes [with closed caption]
System of Particles and Rotational Motion Class 11 All Formulas Short Notes
Angular Momentum Demo Arms IN vs OUT
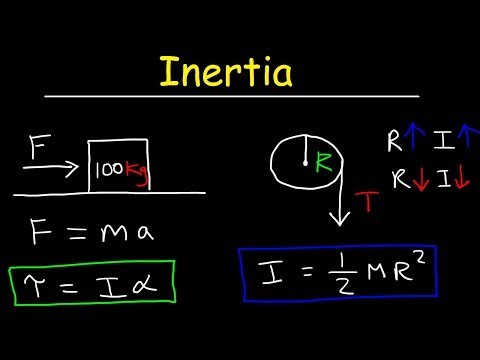
Inertia - Basic Introduction, Torque, Angular Acceleration, Newton's Second Law, Rotational Mot...
Rigid Bodies Work and Energy Dynamics (Learn to solve any question)
Rigid Bodies Impulse and Momentum Dynamics (Learn to solve any question)
Angular Momentum - Basic Introduction, Torque, Inertia, Conservation of Angular Momentum
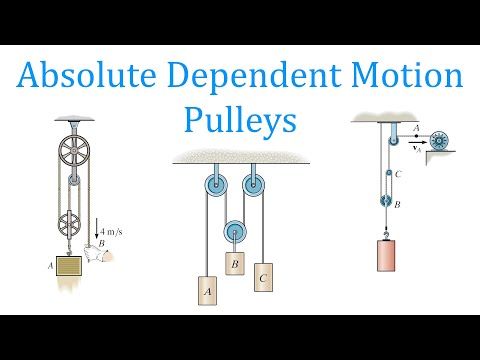
Absolute Dependent Motion: Pulleys (learn to solve any problem)
Magnetic particle dynamics in rotating magnetic field
Single Particle Dynamics | 1D and 2D Worked Examples
Rigid Bodies: Rotation About a Fixed Axis Dynamics (learn to solve any question)
Particle Dynamics Chapter 1, Lecture 6
Particle Dynamics 9.3 - Inertial Accelerations on Noncircular Paths
Relative Motion Analysis of Two Particles Using Translating Axes (learn to solve any problem)
V-2680950: Collective Particle Dynamics in Rotating Droplets Drying Under Acoustic Levitation
Rigid Bodies and Equations of Motion Translation (Learn to solve any question)
Principle of Angular Impulse and Momentum (Learn to solve any problem)
Puri physics laga di😯 (kinematics,NLM, Relative motion, Friction, Circular motion, Rotational M)
Комментарии
 0:00:33
0:00:33
 0:07:21
0:07:21
 0:11:28
0:11:28
 0:32:14
0:32:14
 0:01:34
0:01:34
 0:07:39
0:07:39
![[2015] Dynamics 28:](https://i.ytimg.com/vi/1Y-7jtoHy4g/hqdefault.jpg) 0:13:20
0:13:20
 0:00:09
0:00:09
 0:00:47
0:00:47
 0:11:58
0:11:58
 0:09:43
0:09:43
 0:13:59
0:13:59
 0:06:20
0:06:20
 0:08:01
0:08:01
 0:00:26
0:00:26
 0:57:28
0:57:28
 0:11:25
0:11:25
 0:10:47
0:10:47
 0:14:26
0:14:26
 0:11:28
0:11:28
 0:03:01
0:03:01
 0:13:36
0:13:36
 0:06:43
0:06:43
 0:00:15
0:00:15