AP Precalculus Practice Test: Unit 2 Question #16 Transformations in Composite Functions

My AP Precalculus Practice Tests are carefully designed to help students build confidence for in-class assessments, support their work on AP Classroom assignments, and thoroughly prepare them for the AP Precalculus exam in May.

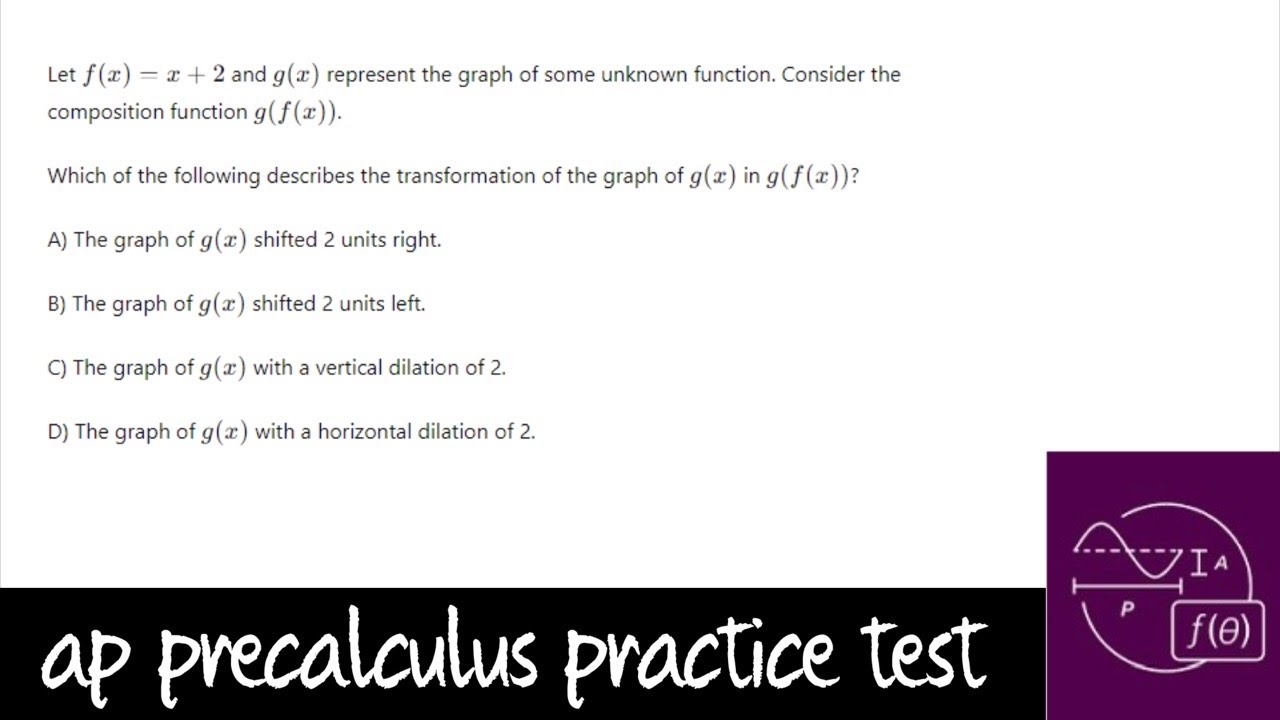
### AP Precalculus Practice Test: Unit 2, Question #16
**Topic:** Transformations in Composite Functions

This question involves understanding how transformations affect composite functions. A composite function combines two functions, where the output of one becomes the input of the other. Common transformations include shifts, stretches, compressions, and reflections.

---

### **Steps to Analyze Transformations in Composite Functions:**

#### 1. **Understanding Composite Functions:**
- A **composite function** is written as \( (f \circ g)(x) = f(g(x)) \), meaning you first apply \(g(x)\), then apply \(f(x)\) to the result of \(g(x)\).

#### 2. **Common Transformations:**
- **Shifts**:
- Horizontal shift: \( f(x-h) \) shifts the graph to the right by \( h \) units.
- Vertical shift: \( f(x) + k \) shifts the graph up by \( k \) units.
- **Reflections**:
- \( f(-x) \) reflects the graph across the \(y\)-axis.
- \( -f(x) \) reflects the graph across the \(x\)-axis.
- **Stretches and Compressions**:
- Vertical stretch: \( a \cdot f(x) \) stretches the graph by a factor of \( a \) when \( |a| is greater than 1 \).
- Vertical compression: \( a \cdot f(x) \) compresses the graph when \( |a| is less than 1 \).
- Horizontal stretch/compression: \( f(bx) \) compresses the graph when \( |b| is greater than 1 \) and stretches when \( |b| is less than 1 \).

#### 3. **Effect of Transformations in Composite Functions:**
- The order of transformations matters. In the composite function \( (f \circ g)(x) \), you first apply \(g(x)\), including its transformations, then apply \(f(x)\), with its transformations.
- For transformations such as \( f(g(x)) \), shifting \(g(x)\) affects the input to \(f(x)\), and the transformation of \(f(x)\) affects the final output.

---

### **Example Problem:**

Given two functions \( f(x) = 2x + 3 \) and \( g(x) = x^2 \), apply the following transformations:

1. Shift \( g(x) \) 2 units to the right.
2. Apply the transformation \( f(x) = 2x + 3 \) to the composite function.

#### Solution Steps:

1. **Shift \( g(x) \) by 2 units to the right:**
- The transformation becomes \( g(x - 2) = (x - 2)^2 \).

2. **Find the composite function:**
- Apply \( f(x) \) to \( g(x) \): \( (f \circ g)(x) = f((x - 2)^2) \).
- Substitute \( f(x) = 2x + 3 \): \( (f \circ g)(x) = 2(x - 2)^2 + 3 \).

Thus, the composite function is \( (f \circ g)(x) = 2(x - 2)^2 + 3 \).

---

### **Key Takeaways:**
- The **order of transformations** is important when working with composite functions.
- **Transformations applied to \( g(x) \)** affect the input to \( f(x) \), and **transformations applied to \( f(x) \)** affect the output.

I have many informative videos for Pre-Algebra, Algebra 1, Algebra 2, Geometry, Pre-Calculus, and Calculus. Please check it out:

/ nickperich

Nick Perich
Norristown Area High School
Norristown Area School District
Norristown, Pa

#math #algebra #algebra2 #maths #math #shorts #funny #help #onlineclasses #onlinelearning #online #study