filmov
tv
Revisiting 2024 AP Calculus AB FRQ #1 (Part D)

Показать описание
So this has been a HUGE discussion. I just want to give you my two cents on how I tend to view rates and interpretation, but it may not be the same as yours.
But this is probably easier to convey than the dozens of messages I've sent so far on it.
But this is probably easier to convey than the dozens of messages I've sent so far on it.
Revisiting 2024 AP Calculus AB FRQ #1 (Part D)
AP Calculus AB 2024: Most Difficult Parts to ALL 6 FRQs EXPLAINED
Use this to ace AP Calc!
2024 Fall AP Calculus AB/BC Open House
AP Calculus BC Unit Tier List In Under 1 Minute
How I Learned AP Calculus BC in 5 DAYS and got a 5 (Ultralearning HACKS)
AP Calculus AB/BC Review 2024 - Day 2 of 4: Units 6-10 LIVE
AP Night 2024
AB Calc 1.3a Continuity Conditions and Types of Discontinuity
AP Calculus AB Content Review Session #1
AB Calc Unit 1 Limits Morning Test Review WS 4
AB Calc Integrals Test Non Calculator Review Day 2
AB Calc 1.3c Intermediate Value Theorem (IVT)
Last Minute AP Calculus Exam Review - Absolute Extrema
Intro to Integral Calculus: AP Calculus AB
Last Minute AP Calculus AB review Help session
AB Calc Trig Unit Review (Day 4) Mon Dec 9
AP Calculus 30L- Definite Integrals are the key to counting BIRDS! And for scaring them!!
Calculus 1 Final Exam Review
ancestors hear my plea: Calc AB edition
AB Calc 5.3b Interpreting Derivative Graphs
AB Calc 4.1a Integrals and Power Rule
💪Try factoring💪 if substitution 🙄doesn't work🙄 #apcalculus #apcalc #unit1 #shorts
AP Calculus AB notes 8-1 Understanding Rates of Change
Комментарии
 0:08:43
0:08:43
 0:12:48
0:12:48
 0:00:22
0:00:22
 0:41:31
0:41:31
 0:00:56
0:00:56
 0:15:34
0:15:34
 3:11:37
3:11:37
 0:18:08
0:18:08
 0:56:05
0:56:05
 2:03:58
2:03:58
 1:02:59
1:02:59
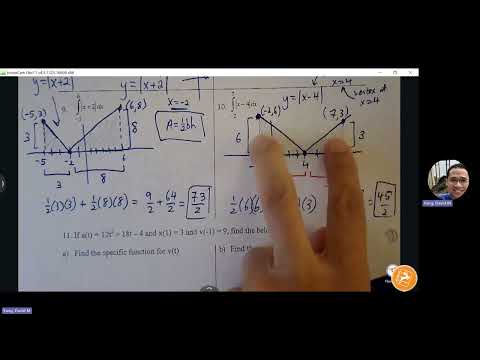 0:45:22
0:45:22
 0:42:48
0:42:48
 0:06:19
0:06:19
 0:36:26
0:36:26
 1:13:17
1:13:17
 0:51:13
0:51:13
 0:00:49
0:00:49
 0:55:58
0:55:58
 0:00:11
0:00:11
 0:52:39
0:52:39
 0:47:01
0:47:01
 0:00:35
0:00:35
 0:20:23
0:20:23